Solutions-Class-12-Chemistry-Chapter-4-Chemical Thermodynamics-Maharashtra Board

(a) ΔH < 0 and ΔS > 0

(a) —500 J

(a) Crystallization of liquid into solid

(b) zero

(c) 2Cl(g) → Cl₂(g)

(a) 43.4 kJ mol^—1

(b) 801.7 J K^—1

(b) 2 and 3

(c) 373.4 K

(c) 373.4 K

• When a gas expands against an external pressure P_ex, changing the volume from V₁ to V₂, the work obtained is given by

W = −P_ex (V₂ − V₁).

• Hence the work is performed by the system when it experiences the opposing force or pressure.
• Greater the opposing force, more is the work.
• In free expansion, the gas expands in vacuum (where it does not experience opposing force, (P = 0). Since external pressure is zero, no work is obtained.

W = −P_ex (V₂ − V₁)

= −0 x (V₂ − V₁) = 0

• Since during expansion in vacuum no energy is expended, it is called free expansion.

According to this law the total energy of a system and surroundings remains constant when the system changes from an initial state to final state.

The law is stated in different ways as follows :

• Energy can neither be created nor destroyed, however, it may be converted from one form into another.
• Whenever, a quantity of one kind of energy is consumed or disappears, an equivalent amount of another kind of energy appears.
• The total mass and energy of an isolated system remain constant, although there may be inter—conservation of energy from one form to another.
• The total energy of the universe remains constant.

Enthalpy of fusion (Δ_fusH) : The enthalpy change that accompanies the fusion of one mole of a solid into a liquid at constant temperature and pressure is called enthalpy of fusion.

For example,

H₂O(s)  H₂O(l), \(\underrightarrow{1\,atm\,273\,K}\)  Δ_fusH = 6.01 kJ mol⁻¹

This equation describes that when one mole of ice melts (fuses) at 0°C (273 K) and 1 atmosphere, 6.01 kJ of heat will be absorbed.

The thermodynamic standard state of a substance (compound) is the most stable physical state of it at 298 K and 1 atmosphere (or 1 bar). The enthalpy of the substance in the standard state is represented as Δ_fH⁰.

The given reaction, 2H(g) → H₂(g) is the formation of H₂(g) from free atoms.

Since two H atoms form one H₂ molecule, Δn = 1 — 2 = — 1 and disorder is decreased. Hence entropy change ΔS < 0 (or negative).

Second law of thermodynamics in terms of entropy :

The second law of thermodynamics states that the total entropy of the system and its surroundings (universe) increases in a spontaneous process.

It is expressed mathematically as

ΔS_total = ΔS_system  + ΔS_surr  > 0 (for spontaneous process)

ΔS_universe = ΔS_system  + ΔS_surr  > 0

The total entropy increases during a spontaneous process that finally reaches equilibrium.

(a) For endothermic reaction, ΔH > 0. This shows the system absorbs heat from surroundings.

∴ Δ_surr H < 0.

∴ Entropy change = Δ_surr S = \(\frac{Δ_{surr}\,H}{T}\)

There is decrease in entropy of surroundings.

(b) For exothermic reaction, ΔH < 0, hence for surroundings, Δ_surr H > 0

∴ Δ_surr > 0.

Spontaneity of reactions when ΔH is positive and ΔS is negative: If ΔH is positive, (ΔH > 0) and ΔS is negative (ΔS < 0), ΔG will be always positive (ΔG > 0) and hence the reaction will be non—spontaneous at all temperatures—

Consider following reversible reaction,

aA + bB ⇌ cC + dD

The reaction quotient Q is,

Q = \(\frac{[C]^c×[D]^d}{[A]^a×[B]^b}\)

The free energy change ΔG for the reaction is

ΔG = ΔG° + RT ln Q

Where ΔG° is the standard free energy change.

At equilibrium

Q = \(\frac{[C]^e_c×[D]^e_d}{[A]^e_a×[B]^e_b}\) = K

ΔG = ΔG° + RT ln K

at equilibrium ΔG = 0

0 = ΔG° + RT ln K

ΔG° = —RT ln K

ΔG° = — 2.303 RT log₁₀K.

(a) Entropy : Being a state function and thermodynamic function it is defined as entropy change (ΔS) of a system in a process which is equal to the amount of heat transferred in a reversible manner (Q_rev) divided by the absolute temperature (T), at which the heat is absorbed. Thus,

Entropy change = \(\frac{\text{Heat transferred reversibly}}{\text{Absolute temperature of transfer}}\)

∴ ΔS = Q_rev/T

Entropy is a measure of disorder in the system Higher the disorder, more is entropy of the system

(b) SI units of entropy are JK⁻¹ and c.g.s. units are cal K⁻¹. It is also expressed in terms of entropy unit (e.u.). Hence 1 e.u. = 1 J K⁻¹.

Reaction and bond enthalpies :

• In a chemical reaction bonds are broken and formed.
• The enthalpies of reactions involving substances having covalent bonds are calculated by knowing the bond enthalpies of reactants and those in products.
• The calculations assume all the bonds of a given type are identical.
• Energy is always required to break a chemical bond while energy is always released in the formation of the bond.

The enthalpy change of a gaseous reactions (Δ_rH°) involving substances with covalent bonds can be calculated with the help of bond enthalpies of reactants and products. (In case of solids we need lattice energy or heat of sublimation while in case of liquids we need heat of evaporation.)

ΔH°_(reaction) = [Sum of bond enthalpies of bonds broken of reactants] — [Sum of bond enthalpies of bonds formed of products]

= ∑ ΔH°_{(bonds broken)} − ∑ ΔH°_{(bonds formes)}

For example for a following reaction,

H₂(g) + Cl₂(g) → 2HCl(g) OR

H—H(g) + Cl—Cl(g) → 2H—Cl(g)

ΔH°_(reaction) = [ΔH°_H—H + ΔH°_Cl—Cl] — 2[ΔH°_H—Cl]

If the energy required to break the bonds of reacting molecules is more than the energy released in the bond formation of the products, then the reaction will be endothermic and ΔH° reaction will be positive.

On the other hand if the energy released in the bond formation of the products is more than the energy required to break the bonds of reacting molecules then the reaction will be exothermic and ΔH° reaction will be negative.

Standard enthalpy of combustion or standard heat of combustion : It is defined as the enthalpy change when one mole of a substance in the standard state undergoes complete combustion in a sufficient amount of oxygen at constant temperature (298 K) and pressure (1 atmosphere or 1 bar). It is denoted by Δ_CH°. p

E.g. C₂H₂(g)+ \(\frac{5}{2}\)O₂(g) → 2CO₂(g)+ H₂O(l),

Δ_CH⁰ = −1300 kJ mol⁻¹, (Δ_CH⁰ is always negative.)

In the above reaction, the standard enthalpy change of the oxidation reaction, −1300 kJ is the standard enthalpy of combustion of C₂H₂(g).

Enthalpy of atomisation (Δ_atoH) : The enthalpy change or amount of heat absorbed accompanying the dissociation of the molecules in one mole of a gaseous substance into free gaseous atoms at constant temperature and pressure is called enthalpy of atomization.

For example,

(a) Cl₂(g) → 2Cl(g) Δ_atoH = 242 kJ mol⁻¹

(b) CH₄(g) → C(g) + 4H(g) Δ_atoH = 1660 kJ mol⁻¹

Consider n₁ moles of gaseous reactants A of volume V₁ change to n₂ moles of gaseous products B of volume V₂ at temperature T and pressure P.

In the initial state, PV₁ = n₁RT

In the final state, PV₂ = n₂RT

PV₂ − PV₁ = n₂RT— n₁RT = (n₂ − n₁)RT = ΔnRT

where Δn is the change in number of moles of gaseous products and gaseous reactants.

Due to net changes in gaseous moles, there arises change in volume against constant pressure resulting in mechanical work, — PΔV.

W = −PΔV= −P (V₂ − V₁) = −ΔnRT

Meaning of each term :

• If n₁ = n₂, Δn = 0, W = 0. No work is performed.
• If n₂ > n₁, Δn > 0, there is a work of expansion by the system and W is negative.
• If n₂ < n₁, Δn< O, there is a work of compression, hence work is done on the system and W is positive.

Consider a certain amount of gas at constant pressure P is enclosed in a cylinder fitted with frictionless, rigid movable piston of area A. Let volume of the gas be V₁ at temperature T.

As the gas expands, it pushes the piston upward through a distance d against external force F pushing the surroundings.

The work done by the gas is,

W = opposing force x distance

= − F x d

− ve sign indicates the lowering of energy of the system during expansion.

If a is the cross section area of the cylinder or piston, then,

W = \(-\frac{F}{a}\) x d x a

Now the pressure IS P_ex = F/a

while volume change is, ΔV = d x a

∴ W = −P_ex x ΔV

If during the expansion, the volume changes from V₁ and V₂ then, ΔV = V₂ − V₁

∴ W = − P_ex(V₂ − V₁)

During compression, the work W is + ve, since the energy of the system is increased,

W = +P_ex(V₂ − V₁)

(a) Intensive property : A property which is independent of the amount of matter in a system is called intensive property.

• Intensive property is characteristic of the system. E.g. density, viscosity, pressure, temperature etc.
• The intensive properties are not additive

(b) Density is a ratio of two extensive properties namely, mass and volume. Since the ratio of two extensive properties represents an intensive property, density is an intensive property. It does not depend on the amount of a substance.

Given : 4CO(g) + 2NO₂(g) → 4CO₂(g) + N₂(g)

Molar mass of CO = 28 g mol^—1, Δ_rH⁰ = − 1200 kJ,

m_CO = 12 g, ΔH = ?

From the reaction,

For 4 x 28g CO ΔH°= − l200 kJ

For 12 g CO ΔH° = \(\frac{-1200×12}{4×28}\)  = − 128.6 kJ

Answer is : Heat evolved = 128.6 kJ.

Consider n moles of an ideal gas enclosed in an ideal cylinder fitted with a massless and frictionless movable rigid piston.

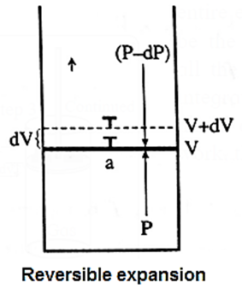
Let V be the volume of the gas at a pressure P and a temperature T.

If in an infinitesimal change pressure changes from P to P — dP and volume increases from V to V + dV. Then the work obtained is,

dW = − (P − dP)dV

= − PdV + dPdV

Since dP.dV is negligibly small relative to PdV

dW = − PdV

Let the state of the system change from A(P₁, V₁) to B (P₂, V₂) isothermally and reversibly, at temperature T involving number of infinitesimal steps.

A(P₁, V₁) ------T------> B (P₂, V₂)

Then the total work or maximum work in the process is obtained by integrating above equation.

W_max = \(\int_{V_1}^{V_2} dW = \int_A^B -pdv\)

‘.’ PV = nRT

∴ P = nRT/V

W_max = \(\int_A^B -nRT\frac{dV}{V}\)

= \(-nRT\int_A^B \frac{dV}{V}\)

= −nRT(lnV₂ − lnV₁)

= −nRT log_e\(\frac{V_2}{V_1}\)

∴ W_max = −2.303 nRT log₁₀ \(\frac{V_2}{V_1}\)

At constant temperature,

‘.’ P₁ x V₁ = P₂ x V₂

\(\frac{V_2}{V_1}\) = \(\frac{P_1}{P_2}\)

W_max = −2.303 nRT log₁₀ \(\frac{P_1}{P_2}\)

where n, P, V and T represent number of moles, pressure, volume and temperature respectively.

For the process,

Δ U = 0, Δ H = 0.

Note : The heat absorbed in reversible manner Q_rev, is completely convened into work Q_rev = W_max Hence work obtained is maximum.

Consider a reaction in which n₁ moles of gaseous reactant in initial state change to n₂ moles of gaseous product in the final state.

Let H₁, U₁, P₁, V₁ and H₂, U₂, P₂, V₂ represent enthalpies, internal energies, pressures and volumes in the initial and final states respectively then,

n₁A(g) → n₂B(g)

The heat of reaction is given by enthalpy change ΔH as,

ΔH = H₂ — H₁

By definition, H = U + PV

∴ H₁ = U₁ + P₁V₁ and H₂ = U₂ + P₂V₂

∴ ΔH = (U₂ + P₂V₂) — (U₁ + P₁V₁)

= (U₂ — U₁) + (P₂V₂ — P₁V₁)

Now, ΔU= U₂ — U₁

Since PV= nRT,

For initial state, P₁V₁= n₁RT

For final state, P₂V₂= n₂RT

∴ P₂V₂ — P₁V₁= n₂RT— n₁RT

= (n₂ — n₁) RT = ΔnRT

Where Δn = [ Number of moles of gaseous products] — [Number of moles of gaseous reactants]

ΔH = ΔU + ΔnRT

If Q_P, and Q_V, are the heats involved in the reaction at constant pressure and constant volume respectively, then since Q_P = ΔH and Q_V = ΔU.

∴ Q_P = Q_V + ΔnRT

The law states that, “Overall the enthalpy change for a reaction is equal to sum of enthalpy changes of individual steps in the reaction”. OR

Heat of reaction is same whether it is carried out in one step or in several steps.

Explanation :

Consider the formation of CO₂(g). .

In one step : C(g) + O₂(g) → CO₂(g) ΔH = — 394 kJ

In two steps : C(s) + 1/2 O₂(g) → CO(g) ΔH₁ = —83 kJ

CO(g)  + 1/2 O₂(g) → CO₂(g) ΔH₂ = —311 kJ

ΔH = ΔH₁+ ΔH₂

—394 kJ = —83kJ + (—311) kJ

Hess’s law treats thermochemical equations mathematically i.e., they can be added, subtracted or multiplied by numerical factors like algebraic equations.

Applications of Hess’s law :

The Hess's law has been useful to calculate the enthalpy changes for the reactions with their enthalpies being not known experimentally.

It is used,

• To calculate heat of formation, combustion, neutralisation, ionization, etc.
• To calculate the heat of reactions which may not take place normally or directly.
• To calculate heats of extremely slow or fast reactions.
• To calculate enthalpies of reactants and products.

Given = ΔS: — 327 JK^—1; ΔH = — 572 kJ

‘.’ ΔG = ΔH — TΔS, and ΔH << ΔS

∴ ΔG < 0, and hence the formation of H2O(l) is spontaneous.

Gibbs free energy, G is defined as,

G = H — TS

where H is the enthalpy, S is the entropy of the system at absolute temperature T.

Since H, T and S are state functions, G is a state function and a thermodynamic function.

At constant temperature and pressure, change in free energy ΔG for the system is represented as,

ΔG = ΔH — T ΔS

Free energy change = Total enthalpy change —Temperature x Entropy change

This is called Gibbs free energy equation for ΔG.

In this ΔS is total entropy change, i.e., ΔS_Total.

The SI units of ΔG are J or kJ (or J mol⁻¹ or kJ mol⁻¹).

The c.g.s. units of ΔG are cal or kcal (or cal mol⁻¹ or kcal mol⁻¹)

The second law explains the conditions of spontaneity as below :

• ΔS_total > o and ΔG < 0, the process is spontaneous.
• ΔS_total < 0 and ΔG > 0, the process is non spontaneous.
• ΔS_total = 0 and ΔG = 0, the process is at equilibrium.

Given : V₁ = 500 cm³ = 0.5 dm³; P_ext = 1.2 x10⁵ Pa = 1.2 bar; W = 36 J;

1dm³bar = 100 J; V₂ = ?

W = −P_ext(V₂ − V₁)

36 J = − 1.2(V₂ − 0.5) dm³ bar

= −1.2(V₂ − 0.5) x 100 J

V₂ − 0.5 = \(-\frac{36}{1.2×100}\) = −0.3

∴ V₂ = 0.5 − 0.3 = 0.2 dm³ = 200 cm³

Answer is : Final volume = 200 cm³.

Given : W_O2 = 24 g, P₁ = 1.6 bar, P₂ = 1 bar, T= 298 K, W_max = ?

W_max = −2.303 nRT log₁₀ \(\frac{P_1}{P_2}\)

= − 2.303 x \(\frac{W_{o2}}{M_{o2}}\) x 8.314 x 298 x log₁₀ \(\frac{1.6}{1}\)

= − 2.303 x \(\frac{24}{32}\) x 8.314 x 298 x 0.2041

= − 873.4 J

Answer is W_max = − 873.4 J.

Given :

NH₄NO₃ (s) → N₂O(g) + 2H₂O(g)

= 132 g;

Molar mass of NH₄NO₃ = (2 x 14) + (3 x 16) + (4 x 1) = 80 g mol^—1

= 80 g mol^—1

T = 273 + 100 = 373K; Δn = ?

For the reaction,

Δn = ∑n_{2 gaseous products} − ∑n_{1 gaseous products} = 3 − 0 = 3

Since there is an increase in number of gaseous moles, the work is done by the system.

Moles of NH₄NO₃ = n = \(\frac{m_{NH_4NO_3}}{M_{NH_4NO_3}}\) = \(\frac{132}{80}\) = 1.65 mol

The given reaction is for 1 mole of NH₄NO₃ Δn = 3 mol

For 1.65 moles of NH₄NO₃ the reaction is

1.65 NH₄NO₃ → 1.65 N₂O + (2 x 1.65) H₂O

∴ 1.65 NH₄NO₃ → 1.65 N₂O + (3.30) H₂O

Δn = (moles of gaseous products) – (moles of gaseous reactants)

= (1.65 + 3.30) – 0 = 4.95 mole              …..(‘.’ NH₄NO₃ is in solid state)

W = − ΔnRT = −4.95 x 8.314 x 373 = −15350 J = −15.35 KJ

Answer is : Work is done by the system. Work done = −15.35 KJ

Given: ΔH⁰ = 219 kJ; ΔS⁰ = −21 J/K = −21 x 10^—3 kJ/K, ΔG° = ?

For standard state, T = 298 K

ΔG° = ΔH° — TΔS°

= 219 — 298 x (—21) x 10^—3

= 219 + 6.258

= 225.3 kJ

Since ΔS < 0 and ΔG° > 0, the reaction is non spontaneous.

Given : 2H₂O(l) + O₂(g) → 2H₂O₂(l)

ΔH° = + 196 kJ

ΔS° = −126 JK⁻¹ = 0.126 kJ K⁻¹

T = 298 K

ΔG° = ?

Cross over temperature = T = ?

ΔG° = ΔH° — T ΔS°

= 196 — 298 (— 0.126)

= 196 + 37.55

= + 233.55 kJ

‘.’ ΔG° > 0, the reaction is non—spontaneous.

ΔH° > 0, ΔS° < 0,

Since at all temperatures, ΔG° > 0, there is no cross over temperature.

Answer is : The reaction is non—spontaneous.

There is no cross—over temperature for the reaction.

Given : C₂H₄(g) + HCl(g) → C₂H₅Cl(g), T = 298 K; ΔH = −72.3 kJ,

PV = ?, ΔU= ?

Δn = (moles of gaseous products) – (moles of gaseous reactants)

= 1− (1 + 1) = −1 mol

For PV work :

W= − ΔnRT

= − (−1) x 8.314 x 298 = 2478 J = 2.478 kJ

ΔH = ΔU + ΔnRT

∴ ΔU = ΔH − ΔnRT

= − 72.3 − (− 2.478)

= − 69.82 kJ

Answer is : PV work = 2.478 kJ

                    ΔU= − 69.82 kJ.

Given : V₁ = 8.0 dm³; V₂ = 4.0 dm³; P_ext = 43 bar

W = ? , What direction work energy flows?

W = −P_ext(V₂ − V₁)

= − 43 (4 − 8)

= 172 dm³ bar

= 172 x 100]

= 17200 J

= 17.2 kJ

In this compression process, the work is done on the system and work energy flows into the system.

Given :

(a) 4HCl(g) + O₂(g) → 2Cl₂(g) + 2H₂O(g)

n_HCl = 1mol, T = 273 + 200 = 473 K, W= ?

For 4 mol HCl

Δn = (2 + 2) − (4 + 1) = −1 mol

For 1 mo1 HCl Δn = − 1/4 = − 0.25 mol

W = − ΔnRT = − (−0.25) x 8.314 x 473 = 983.1 J

(b) Δn = (1 + 1) – 2 = 0 mol

W = –ΔnRT= – (0) x 8.314 x 473 = 0

Answer is : (a) W = 983.1 J

                   (b) W = 0.0 J.

Given : The given reaction is for 1 mol O₂ or 32 g O₂.

For 6.0 g O₂ ΔH⁰ = 38.55 kJ

For32g O₂ ΔH⁰ = \(\frac{32×38.55}{6}\) = 205.6 kJ

Answer is : ΔH° = 205.6 kJ.

Given :

Δ_C H⁰CH₃OH = −726 kJ mol⁻¹

Δ_fH⁰CO₂ = −393 kJ mol⁻¹

Δ_fH⁰H₂O = −286 kJ mol⁻¹

Δ_f H⁰CH₃OH = ?

Required thermochemical equation :

C(s) + 2H₂(g) + \(\frac{1}{2}\)O₂(g)  → CH₃OH(l) …..(1) ΔH⁰₁ = ?

Given equations :

CH₃OH(l)  + \(\frac{3}{2}\)O₂(g) → CO₂(g) + 2H₂O(l) …..(2) ΔH⁰₂

−             −                     −         −

C_(graphite) + O₂(g) → CO₂(g) ….. (3) ΔH⁰₃

+

H₂(g) + \(\frac{1}{2}\)O₂(g)  → H₂O(l) ….. (4) ΔH⁰₄ x 2

+

Eq.(1) =  − eq.(2) + eq.(3) + 2eq.(4)

∴ ΔH⁰₁ = −ΔH⁰₂  + ΔH⁰₂  + 2ΔH⁰₃

= − (−726) + (—293) + 2(—286)

= 726 − 393 − 572

= −239 kJ mol⁻¹

Answer is : Standard enthalpy of formation = Δ_f H⁰ = −239 kJ mol⁻¹

Given :

Given equations :

2H₃BO₃(aq) → B₂O₃(s) + 3H₂O(l) …..(1) ΔH⁰₁ = 14.4 kJ mol^—1

H₃BO₃(aq) → HBO₂(aq) + H₂O(l) …..(2) ΔH⁰₂ = −0.02 kJ mol^—1

H₂B₄O₇(s) → 2P₂O₃(s) + H₂O(l) .…..(3) ΔH⁰₃ =17.3 kJ mol^—1

Required equation :

H₂B₄O₇(s) + H₂O(l) → 4HBO₂(aq) .…..(4) ΔH⁰₄ = ?

To obtain eq.(4)

∴ eq.(4) = 4eq.(2) + eq.(3) – 2eq.(1)

∴ ΔH⁰₄ =  4ΔH⁰₂ + ΔH⁰₃ – 2ΔH⁰₁

= 4(−0.02) + 17.3 — 2(14.4)

= – 0.08 + 17.3 – 28.8

= – 11.58 kJ

Enthalpy change for the reaction = Δ_rH⁰ = – 11.58 kJ

Answer is : Δ_rH⁰ for the given reaction = – 11.58 kJ.

Given : Mass of ice = m = 180 g

T₁ = 273 + 0°C = 273K

T₂ = 273 +100 °C = 373 K

Δ_fusH⁰_(ice) = 6.01 kJ mol⁻¹

Δ_vapH⁰_{( )} =  40.7 kJ mol⁻¹

Specific heat of water = C = 4.18 J g⁻¹ K⁻¹

For converting 180 g ice into vapour, ΔH_Total = ?

Number of moles of H₂O = 180/18 = 10 mol

The total process can be represented as,

H₂O(s)_{10 mol}  \(\underleftrightarrow{ΔH_1}\) H₂O(l) \(\underrightarrow{ΔH_2}\) H₂O(l) \(\underrightarrow{ΔH_3}\) H₂O(g)

(a) ΔH₁ = Δ_fusH⁰ =10 mol x 6.01 kJ mol⁻¹ = 60.1 kJ

(b) When the temperature of water is raised from 0 °C to 100 °C (i.e., 273 K to 373 K), then

ΔH₂ = m x C x ΔT

= m x C x (T₂ − T₁)

=180 g x 4.18 Jg⁻¹ K⁻¹ x (373 − 273) x 10⁻³ kJ = 75.24 kJ

(c) ΔH₃ = Δ_vapH = 10 mol x 40.7 kJ mol⁻¹ = 407 kJ

Hence total enthalpy change,

ΔH_Total = ΔH₁ + ΔH₂ + ΔH₃

= 60.1 + 75.24 + 407

= 542.34 kJ

Answer is : (a) ΔH₁ =60.1 kJ (b) ΔH₂ = 75.24 kJ (c) ΔH_Total = 542.34 kJ.

Given : C₂H₄(g) + H₂(g) → C₂H₆(g)

100 mL      100 ml        100 ml

ΔH= − 620 J; V_(C2H4) = 100 mL; V_(H2) = 100 mL, P_ext = 1 bar, W = ?, ΔU=?

ΔV = 100 − (100 + 100) = −100mL= −0.1 dm³

W = −P_ext(V₂ − V₁)

= −P_ext x ΔV

= −1 x (−0.1)

= 0.1 dm³bar

= 0.1 x 100 J

= +10 J

ΔH = ΔU + PΔV

ΔU = ΔH − PΔV = −620 − (+10) = −610 J

Answer is : W = +10 J, ΔU = −610 J

Given : NH₄NO₃(s) → N₂O(g) + 2H₂O(g).

n = 2 mol, T = 273 + 100 = 373 K,

W = ?, Comment on work = ?

Δn_g = (1 + 2) — 0 = 3 mol

For 1 mol of NH₄NO₃ Δn_g = 3 m

For 2 mol of NH₄NO₃ Δn_g = 6 mol

Due to 6 moles of gaseous products from 2 mol NH₄NO₃, there is work of expansion, hence work is done by the system.

W = − ΔnRT = −6 x 8.314 x 373

= −18606 J

= −18.61 KJ

Answer is : Work is done by the system

                    W= − 18.61 kJ.