Solutions-Class-11-Science-Physics-Chapter-12-Magnetism-Maharashtra Board

(D) not necessarily 1/r³ at all points

(C) passing through the magnetic axis of Earth

(B) 45^o

(D) are in the direction from S pole to N pole

(A) 0^o

(D) one of the geomagnetic poles

(C) both a force and a torque

• A bar magnet can be cut into two halves, exposing two new faces with opposite poles. The magnetic dipole moment of each piece is half that of the original magnet.
• If cut transversely, the new exposed poles have the same pole strengths as the original but have half the magnetic length.
• If cut along its length, the new exposed poles have half the pole strengths as the original but have the same magnetic length.

Gauss‘ law in magnetism would be analogous to that in electrostatics : The net magnetic flux through a surface enclosing a monopole of pole strength p would be equal to the product μ₀p, where μ₀ is the permeability of free space.

\(\oint \limits_{s} \vec{B}.\vec{dS}\) = μ₀p

where the integration is over the closed surface S enclosing the magnetic monopole.

Gauss’ law in magnetism : The net magnetic flux Φ_m through a closed surface of arbitrary shape is zero.

In integral form, Φ_m = \(\oint \limits_{s} \vec{B}.\vec{dS}\) = 0

where the integration is over a closed surface S.

Explanation : Below figure schematically shows the magnetic field lines of a bar magnet and a current—carrying solenoid, and the electric field lines of an electric dipole. The dotted curves S₁ and S₂ represent Gaussian surfaces.

In Figs. (a) and (b), the number of magnetic field lines entering each of the surfaces S₁ and S₂ are equal to the number of lines leaving the respective surfaces. That is, the net flux Φ_m through them is zero. Although S₂ appears to enclose the north pole, Φ_m = 0 means that there is no magnetic charge there.

For an electrostatic case, however, Gauss’ law states that the net electric flux through a closed surface S is Φ_e = \(\oint \limits_{s} \vec{E}.\vec{dS}=\frac{∑q}{ε_0}\), where ∑q is the net electric charge enclosed by S.

In below Fig. Φ_e =0 through S₁ and ∑q is also zero, while there is a net outward flux through S₂ and ∑q = + q, both cases consistent with Gauss’ law and the fact that an isolated electric charge exists.

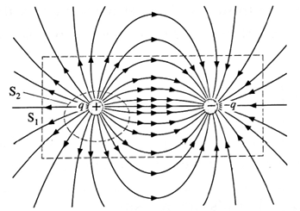
Thus, by Gauss’ law in magnetism,

• magnetic monopoles do not exist — magnetic field is dipolar in character.
• net magnetic flux through any volume is always zero.

• Geographic meridian : The vertical plane at a place through the geographic North and South Poles (i.e., containing the geographic axis) is called the geographic meridian at that place.
• Magnetic declination does not depend on latitude. The lines of flux are pretty random.

• Angle of Dip (or inclination) : At a place on the Earth's surface, the magnetic induction of the Earth \(\vec{B}\) is, in general, inclined to the surface. A dip needle placed with its plane in the magnetic meridian points in the direction of  at that place. The angle which a dip needle makes with the horizontal is the angle between \(\vec{B}\) and the horizontal. This angle is called the dip or inclination, δ at the place.
• On the magnetic equator, dip angle = 0°. At the north and south magnetic poles, the dip angle is 90° and 270° degrees.

Given : q_m = 100 A.m, d = 20 cm = 0.2 m, 2l = 5 x 10⁻² m, q_mB =200 A.m, \(\frac{μ_0}{4π}\)  = 10⁻⁷ T.m/A

Dipole moment of the bar magnet,

m = q_mB(2l) = (200)( 5 x 10⁻²) = 10 A·m²

The axial field of the short bar magnet,

B_axis = \(\frac{μ_0}{4π}.\frac{2m}{d^3}\) = (10⁻⁷)\((\frac{2(10)}{0.2^3})\)  = 2.5 x 10⁻⁴ T

∴ The force on the magnetic pole,

F = q_m x B_axis = (100) (2.5 x 10⁻⁴) = 2.5 x 10⁻² N = 25 mN

Here, the dip needle is placed in a plane that makes an angle of 30° with the magnetic meridian. Then, as shown in the figure, the dip needle lies in the plane OSTR which makes an angle θ = 30° with the plane OPQR which is in the magnetic meridian. In the figure, \(\vec{B}=\vec{B}_h+\vec{B}_v\) is the Earth's magnetic field at the place and \(\vec{B}_1\) is the component of \(\vec{B}\) along the dip needle.

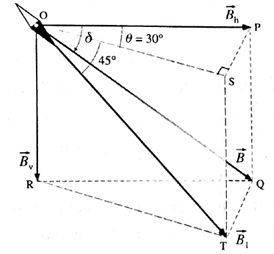
Bv =ST =OT sin ∠ SOT = B₁ sin 45°

Also, OS = OP cos 0

∴ OT cos 45° = OP cos θ

∴ B₁ cos 45° = B_h cos 30°

∴  B_h = B₁ cos 45° / cos 30°

∴ The true dip angle is given by

tan δ = \(\frac{B_v}{B_h}\) = \(\frac{B_1\,sin\,45°}{B_1\,cos\,45°/cos\,30°}\) = tan 45° cos 30° = (1)\((\frac{\sqrt{3}}{2})\)

∴ δ = tan^-1\((\frac{\sqrt{3}}{2})\) = 40°54'

Given : m₁ = m₂ = 1 A.m², distance between their centres = 2 m, \(\frac{μ_0}{4π}\) = 10⁻⁷ T.m/A

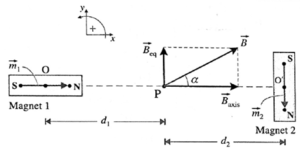
Point P lies on the axis of magnet 1 and on the equator of magnet 2, equidistant from both (Fig.). Since OO'= 2 m, d₁ = d₂ = 1m

Orienting the magnetic moments  and  as shown without loss of generality, the axial field of magnet 1 at P is

\(\vec{B}_1\) = \(\frac{μ_0}{4π}.\frac{2m_1}{d_1^3}\)

= (10⁻⁷)\((\frac{2(1)}{1^3})\)   = (2 x 10⁻⁷ T)\(\hat{i}\)

and the equatorial field of magnet 2 at P is,

\(\vec{B}_2\) = \(\frac{μ_0}{4π}.\frac{-\vec{m}_1}{d_2^3}\)

= (10⁻⁷)\((\frac{1}{1^3})\)   = (10⁻⁷ T)\(\hat{j}\)

∴ The net magnetic induction at P,

\(\vec{B}=\vec{B}_1+\vec{B}_2\) = \((2\hat{i}+\hat{j})\) x 10⁻⁷ T

∴ The magnitude of the net magnetic induction at P,

B = |(\vec{B}\)| = 10⁻⁷ x \(\sqrt{2^2+1^2}\) = \(\sqrt{5}\)  x 10⁻⁷ T

tan ∝ = B₂/B₁ = 1/2

∴ The angle made by  with  = ∝ = tan⁻¹ \((\frac{1}{2})\) = 26°36’

(i) For south poles of such circular magnets facing each other:

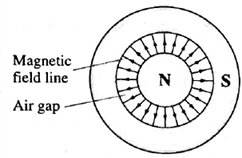
(ii) For south poles of bar magnets facing each other:

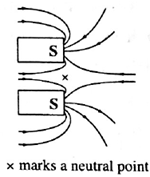
Note : The question is wrong as isolated magnetic monopoles are not observed.

The magnetic lines of force between two magnets will depend on their relative positions. Considering the magnets to be placed one beside the other as shown in the figure, the magnetic lines of force will be as shown.

In Fig. neutral point(s), where the resultant magnetic field is zero, are marked with a cross.