Notes-Part-1-Class-12-Chemistry-Chapter-4-Chemical Thermodynamics-Maharashtra Board

Different forms of energy and concept of interconversion of different forms of energy :

The energy of a system has many different forms as follows : .

• Kinetic energy which arises due to motion, like rotational, vibrational and translational.

• Potential energy which arises due to position and state of a matter. If depends upon the temperature of the system.
• Heat energy (or thermal energy) which is transferred from the hotter body to the colder body.
• Radiant energy which is associated with electro-magnetic or light radiation.
• Electrical energy produced in the galvanic cells.
• Chemical energy stored in chemical substances.

All these various forms of energy can be converted from one form to another without any loss, i.e. all these forms of energy are interconvertible

Examples :

• A body at very high level possesses higher potential energy. When it falls down, potential energy is converted into kinetic energy.
• Falling of water from high level is used to drive turbines converting potential energy into kinetic energy which is further converted into electrical energy,
• In galvanic cells, chemical energy is convened into electrical energy.
• In electrolytic cells, chemical energy is convened into electrical energy. But during interconversion, the energy can neither be created nor destroyed, and there is a conservation of energy.

Types of systems :

• Open system
• Closed system
• Isolated system
• Homogeneous system
• Heterogeneous system.

 Open system : It is defined as a system which can exchange both matter and energy with its surroundings.

• In this system, the total amount of matter does not remain constant.
• In this system, the total amount of energy does not remain constant.

• Example : Fig.(a) shows an open cup containing hot coffee placed in a room. We observe coffee cools down releasing heat to the surroundings. The water vapour from coffee simultaneously passes into surroundings. So that a system (coffee) which exchanges both energy and matter with the surroundings

Closed system : It is defined as a system which can exchange only energy but not the matter with its surroundings.

• In this system, the total amount of matter remains constant.
• In this system, the total amount of energy does not remain constant.
• Example : In Fig. (b), a cup containing hot coffee is covered with a saucer. Coffee cools down by giving away heat to the surroundings. The water vapour from coffee now does not pass into surroundings. So that a system that exchanges energy and not the matter with the surroundings.

Isolated system : It is defined as a system which can neither exchange energy nor matter with its surroundings.

• In this, the total amount of energy remains constant.

• Example : In Fig. (c), a cup containing hot coffee covered with a saucer is insulated from the surroundings. Coffee does not cool down. Moreover, there is no escape of water vapour into the surroundings. Such a system that does not allow exchange of either energy or matter with the surroundings.

In actual practice, perfectly isolated system is not possible. Universe represents an isolated system.

Properties of system :

The properties of a system are classified as

(A) Extensive property and (B) Intensive property

Extensive property : A property which depends on the amount of matter present in a system is called an extensive property.

Explanation :

• More the quantity (or amount) of the matter of the system, more is the magnitude of extensive property. e.g., mass, volume, heat, energy, enthalpy, etc.
• The extensive properties are additive.

Intensive property : A property which is independent of the amount of matter in a system is called intensive property.

Explanation :

• Intensive property is characteristic of the system. e.g., refractive index, density, viscosity, temperature, pressure, boiling point, melting point, freezing point of a pure liquid, surface tension, etc.
• The intensive properties are not additive

Features :

• In this process, the temperature at initial state, final state and throughout the process remains constant.
• In this process, system exchanges heat energy with its surroundings to maintain constant temperature. E.g., in case of exothermic process liberated heat is given to the surroundings and in case of endothermic process heat is absorbed from the surroundings so that temperature of the system remains constant and  ΔT = 0.
• Isothermal process is carried out with a closed system.
• Internal energy (U) of the system remains constant, hence, ΔU = 0.
• In this process, pressure and volume of a gaseous system change.
• In this process, the system exchanges heat with the surroundings. Q ≠ 0 (Closed system)
• In this process, the system is not thermally isolated.
• In this process, Q = − W as ΔU = 0.
• ΔH = 0.

Features :

• In this process, the volume (of gaseous system) changes against a constant pressure.
• Since the external atmospheric pressure remains always constant, all the processes carried out in open vessels, or in the laboratory are isobaric processes.
• In this volume and temperature change
• Internal energy of a system changes, hence ΔU ≠ 0.
• In this process, the system does not exchange heat with the surroundings. Q = 0 (Isolated system)
• In this process, the system is thermally isolated.
• In this process, W = ΔU,
• ΔH ≠ 0

Features :

• In this process, temperature and pressure of the system change but volume remains constant.
• Since ΔV = 0,no mechanical work is performed.
• In this internal energy (U) of the system changes.
• Example of this process in cooking takes place in a pressure cooker.

Features :

• An adiabatic process is carried out in an isolated system.
• In this process, temperature and internal energy of a system change, ΔT ≠ 0, ΔU ≠ 0.
• During expansion, temperature and energy decrease and during compression, temperature and energy increase.
• If the process is exothermic, the temperature rises and if the process is endothermic the temperature decreases in the adiabatic process.

Features :

• This is a hypothetical process.
• Driving force is infinitesimally greater than this opposing force throughout the change.
• The process can be reversed at any point by making infinitesimal changes in the conditions.
• The process takes place infinitesimally slowly involving infinite number of steps.
• At the end of every step of the process, the system attains mechanical equilibrium, hence throughout the process, the system exists in temperature-pressure equilibrium with its surroundings.
• In this process, maximum work is obtained.
• Temperature remains constant throughout the isothermal reversible process.

Features :

• It takes place without the aid of external agency.
• All irreversible processes are spontaneous and takes finite time for completion.
• All natural processes are irreversible processes.
• The thermodynamic equilibrium is attained only at the end of the process.
• They are real processes and are not hypothetical.
• The opposing force is significantly less than the driving force.
• It is a practical or real and spontaneous process.
• Work derived from such a process is always less than the maximum work.

Process of expansion :

Consider an ideal cylinder fitted with a piston and filled with H₂O₂(l).

2H₂O₂(l) → 2H₂O(l) + O₂(l)

The oxygen gas produced pushes the piston upwards lifting the mass. Thus, the system performs the work on the surroundings and loses energy by expansion. In this work is done by the system.

Process of compression :

Consider an ideal cylinder fitted with a piston and containing gaseous NH₃(g) and HCl(g).

NH₃(g) + HCl(g) → NH₄Cl(s)

As the reaction proceeds, due to consumption of gases, the volume decreases and there is work due to compression. In this work is done on the system by surroundings and the system gains energy.

Expression for pressure-volume (PV) work

Consider a certain amount of gas at constant pressure P is enclosed in a cylinder fitted with frictionless, rigid movable piston of area A. Let volume of the gas be V₁ at temperature T.

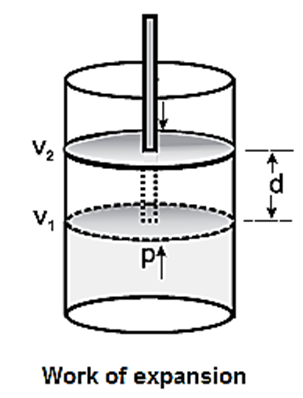
As the gas expands, it pushes the piston upward through a distance d against external force F pushing the surroundings.

The work done by the gas is,

W = opposing force x distance

= − F x d

− ve sign indicates the lowering of energy of the system during expansion.

If a is the cross section area of the cylinder or piston, then,

W =  \(-\frac{f}{a}×d×x\)

Now the pressure IS P_ex = F/a

while volume change is, ΔV = d x a

∴ W = −P_ex x ΔV

If during the expansion, the volume changes from V₁ and V₂ then, ΔV = V₂ − V₁

∴ W = − P_ex(V₂ − V₁)

During compression, the work W is + ve, since the energy of the system is increased,

W = +P_ex(V₂ − V₁)

Concept of maximum work : Eq. W = −P_ex shows the amount of work performed by a system is governed by the opposing force (P_ex).

• Larger the opposing force more work is done by the system to overcome it.
• Thus when the opposing force (P_ex) becomes greater than the driving force (P) the process gets reversed. Since the opposing force cannot be greater than the driving force it should be the maximum.
• The maximum work is obtained from the change which is thermodynamically reversible.

Expression for the work obtained in an isothermal reversible expansion of an ideal gas. OR Expression for maximum work :

Consider n moles of an ideal gas enclosed in an ideal cylinder fitted with a massless and frictionless movable rigid piston.

Let V be the volume of the gas at a pressure P and a temperature T.

If in an infinitesimal change pressure changes from P to P — dP and volume increases from V to V + dV. Then the work obtained is,

dW= − (P − dP)dV

= − PdV + dPdV

Since dP. dV is negligibly small relative to PdV

dW = − PdV

Let the state of the system change from A(P₁, V₁) to B (P₂, V₂) isothermally and reversibly, at temperature T involving number of infinitesimal steps.

Then the total work or maximum work in the process is obtained by integrating above equation.

W_max = \(\int_{A}^{B}\)dW =\(\int_{A}^{B}\)−PdV

‘.’ PV = nRT

∴ P = nRT/V

W_max = \(\int_{V_1}^{V_2}\)−nRT\(\frac{dV}{V}\)

= −nRT\(\int_{V_1}^{V_2} \frac{dV}{V}\)

= −nRT(lnV₂ − lnV₁)

= −nRT log_e  \(\frac{V_2}{V_1}\)

∴ W_max = −2.303 nRT log₁₀ \(\frac{V_2}{V_1}\)

At constant temperature,

‘.’ P₁ x V₁ = P₂ x V₂

\(\frac{V_2}{V_1}=\frac{P_1}{P_2}\)

W_max = −2.303 nRT log₁₀ \(\frac{P_1}{P_2}\)

Characteristics of maximum work :

• The process is carried out at constant temperature,
• During the complete process, driving force is infinitesimally greater than opposing force.
• Throughout the process, the system exists in equilibrium with its surroundings.
• The work obtained is maximum. This is given by,

W_max = −2.303 nRT log₁₀ \(\frac{V_2}{V_1}\)

OR

W_max = −2.303 nRT log₁₀ \(\frac{P_1}{P_2}\)

            where n, P, V and T represent number of moles, pressure, volume and

            temperature respectively.

• ΔU = 0, ΔH = 0.
• The heat absorbed in reversible manner 

         Q_rev, is completely converted into work

         Q_rev = − W_max

         Hence work obtained is maximum.

Explanation :

• The internal energy of a system is a state function and thermodynamic function. It is denoted by U.
• Its value depends on the state of a system.
• The change in internal energy (A U) depends only on the initial state and the final state of the system. ΔU = U₂ − U₁

• It is an extensive property of the system.
• It has same unit as heat and work.
• Total internal energy U of the system is, 

         Total energy = Potential energy + Kinetic energy

Formulation of first law of thermodynamics (mathematical equation for the first law of thermodynamics) :

• The first law of thermodynamics is based on the principle of conservation of energy.
• If Q is the heat absorbed by the system and if W is the work done by surroundings on the system then the internal energy of the system will increase by ΔU.
• From the conservation of energy we can write, Increase in internal energy of the system = Quantity of heat absorbed by the system + Work done on the system

ΔU = Q + W

• For an infinitesimal change,

dU = dQ + dW

First law of thermodynamics for various processes :

Isothermal process : This is a process which is carried out at constant temperature. Since internal energy, U of the system depends on temperature there is no change in the internal energy U of the system. Hence ΔU = 0.

By first law of thermodynamics,

ΔU = Q + W

∴ 0 = Q + W

∴ Q = −W Or W = −Q.

• Hence in expansion, the heat absorbed by the system is entirely converted into work on the surroundings.
• In compression, the work done on the system is converted into heat which is transferred to the surroundings by the system, keeping temperature constant.

Isobaric process : In this, throughout the process pressure remains constant. Hence the system performs the work of expansion due to volume change ΔV.

‘W = − P_ext x ΔV

Let Q_P, be the heat absorbed by the system at constant pressure.

By first law of thermodynamics,

ΔU = Q_P + W

∴. ΔU = Q_P − P_ext x ΔV

Or Q_P = ΔU + P_ext x ΔV

In this process, the heat absorbed Q_P is used to increase the internal energy (ΔU) of the system.

Adiabatic process : In this process, the system does not exchange heat, Q with its surroundings.

 Q = 0.

Since by first law of thermodynamics,

ΔU = Q + W

∴ ΔU = W_ad

Hence,

• The increase in internal energy ΔU is due to the work done on the system by surroundings. This results in increase in energy and temperature of the system.
• If the work is done by the system on surroundings, like expansion, then there is a decrease in internal energy ( −ΔU) and temperature of the system decreases.