Notes-Part-1-Class-11-Science-Physics-Chapter-4-Laws of Motion-Maharashtra Board

Trajectory of a particle :

Trajectory of any motion is decided by acceleration \(\vec{a}\) and the initial velocity \(\vec{u}\)  .

(i) Trajectory of a particle when, the particle is at rest and is given an acceleration which is constant in direction :

• Rectilinear, i.e., one-dimensional, motion. The particle will move with an increasing speed in the direction of the acceleration.

(ii) Trajectory of a particle when, the particle has a non-zero velocity \(\vec{u}\)  and is given an acceleration along ± \(\vec{u}\)   :

• Rectilinear, i.e., one-dimensional, motion. If the acceleration \(\vec{a}\) is in the direction of \(\vec{u}\) the particle will move with an increasing speed in the direction of the acceleration. If \(\vec{a}\) is in the direction of \(-\vec{a}\), the particle will decelerate, come to a momentary stop and move in the opposite direction with increasing speed.

(iii) Trajectory of a particle when, the particle has a non-zero velocity and an acceleration that is always perpendicular to the velocity and constant in magnitude :

• Uniform circular motion, in the plane containing the velocity and acceleration vectors.

(iv) Trajectory of a particle when, the particle has a non-zero velocity that is not along its acceleration which is constant in magnitude and direction :

• Parabolic motion, in the plane containing the velocity and acceleration vectors.

Discussion : Newton's first law of motion defines force while the second law measures it.

Newton's first law of motion gives, qualitatively, the relation between force and motion.

We know that there is a force \(\vec{F}\) at work (more precisely, a resultant or unbalanced force) only when a particle's velocity changes, i.e., it has an acceleration \(\vec{a}\). Thus, force is that physical quantity which causes acceleration.

An acceleration of one body is to be associated with the presence of one or more other bodies mutually interacting with it.

Then, according to Newton's first law of motion, if \(\vec{F}\) = 0, \(\vec{a}\) = 0. In other words, if a body is observed to be at rest or to move uniformly in a straight line, either there are no other bodies mutually interacting with it, or the other bodies are so positioned that they produce at all times a resultant acceleration of zero.

Newton's second law gives a quantitative measure of a force : \(\vec{F}=\frac{dv}{dt}\) = \(m\vec{a}\)

, where m ≡ mass of the body assumed to be constant and \(\vec{p}\) ≡ momentum.

We then define the SI unit of force, the newton, as that force which produces an acceleration of 1 m/s² in a body of mass 1 kg.

Thus, Newton’s first law of motion defines force and the second law measures it.

Importance of Newton's first law of motion :

• It shows an equivalence between ‘state of rest’ and ‘state of uniform motion along a straight line’ as both need a net unbalanced force to change the state. Both these are referred to as ‘state of motion’. The distinction between state of rest and uniform motion lies in the choice of the ‘frame of reference’. Newton's first law thus defines inertial frames of reference in which all the three laws hold.
• It qualitatively defines force as a physical quantity that causes acceleration.
• It ascribes to a material body a fundamental property called inertia that causes the body to resist any change in its motion.

Importance of Newton's second law of motion :

• It provides a quantitative measure of force.

• Newton’s own version of the second law, \(\vec{dp}\) = \(\vec{F}dt\), where  is the net force acting on a body, recognized momentum as a more useful concept in developing the science of dynamics.

Importance of Newton's third law of motion :

• Newton's laws assume that the force on a body has its origin in other bodies, i.e., bodies have an influence on one another, which we attribute to an entity called force. Newton's third law of motion says that this influence is mutual: one body cannot influence another without itself being affected by that body.
• Action and reaction forces are always on different objects, i.e. an isolated force does not exist. Forces act always in pairs, and simultaneously. Any of the pair can be called as action and the other as reaction. However, a given pair act on the two different interacting bodies; hence, they do not cancel each other.

Discussion : Newton's first law of motion can be deduced from his second law.

By Newton's second law of motion, \(\vec{dp}\) = \(\vec{F}dt\)  , where \(\vec{dp}\)  is the change in momentum of a body in time dt when a net force  is acting on the body.

If the mass of the body is constant,

\(\vec{dp} = d(m\vec{v})\) = \(md\vec{v}\)

So that, if \(\vec{F}\) = 0, \(\vec{v}\) is constant, Hence if there is no force, velocity will not change. This is nothing but Newton’s first law of motion.

Limitation of Newton’s laws of motion :

• Newton’s laws are applicable only in the inertial frames of reference which they define. If the body is in a frame of reference of acceleration (a), we need to use a pseudo force (−m\(\vec{a}\) ) in addition to all the other forces while writing the force equations.
• Newton’s laws are applicable for point objects.
• Newton’s laws are applicable to rigid bodies. A body is said to be rigid if the relative distances between its particles do not change for any deforming force.
• For objects moving with speeds comparable to that of light, Newton’s laws of motion do not give results that match with the experimental results and Einstein's special theory of relativity has to be used.
• Behaviour and interaction of objects having atomic or molecular sizes cannot be explained using Newton’s laws of motion, and quantum mechanics has to be used.

Ranges and Relative Strength :

• The gravitational force and the electromagnetic force are long-range forces, extending, in principle, to infinity.
• Both the strong nuclear force and the weak nuclear force are extremely short-range forces; the strong nuclear force becomes essentially zero for separations between neutrons and protons (collectively referred to as nucleons) greater than about 3 x 10⁻¹⁵ m, and the weak nuclear force within a nucleon has a range of about 10⁻⁸ m.
• In relative strength, the strong nuclear force is the strongest of the four while the gravitational force is the weakest : gravitational force < weak nuclear force < electromagnetic force < strong nuclear force.

Characteristics :

• It is an attractive force and obeys the inverse square law of distance.
• It does not depend on the intervening medium.
• Gravity is a very weak but long-range interaction. It is because G is so small that it is hard to measure it and makes gravity the weakest of the four basic forces in nature.
• Because gravity is so weak, it is of importance only when the interacting bodies are uncharged and at least one of the bodies is very massive, i.e., of astronomical size.
• Because it is always of attraction and the celestial bodies are practically uncharged it is responsible for the large scale structure of the universe.

Characteristics :

• Electric force can be attractive or repulsive but obeys the inverse square law of distance.
• Electromagnetic forces depend on the intervening medium.
• They are long-range forces.
• They act only when both the interacting bodies are charged and then they are enormously greater than the gravitational force between these bodies.

Characteristics :

• It is attractive and the strongest of the basic forces in nature.
• The strong interaction is an extremely short- range force, effective only at separations of about 10⁻¹⁵ m, i.e., only within a nucleus. At the subnuclear distances involved, it is the dominant force — about 10² times stronger than the electromagnetic force.
• Beyond about 3 x 10⁻¹⁵ m, the force is essentially zero, decreasing with distance much more rapidly than the inverse-square behavior of the gravitational and the electromagnetic interaction.
• At the subnuclear distances involved, it is an attractive force which acts between all pairs of nucleons, i.e., between proton and neutron, between proton and proton, and between neutron and neutron. That is, it is charge independent and its properties determine the structure of nuclei.

Characteristics :

• The weak nuclear force is an extremely short-range force, effective only within a nucleon (~ 10⁻¹⁸ m).
• It exists between all pairs of subatomic particles : While it is the exclusive force between an electron and an antineutrino, the same force also exists between two protons. In the latter case, it is overwhelmed by the electric repulsion and strong nuclear attraction.
• The weak nuclear force is not as weak as the gravitational force, but much weaker than the strong nuclear and EM forces.

Contact and Non-Contact Forces:

Forces can be grouped into two categories :

Contact forces :

• These are due to direct physical contact between interacting objects.
• Normal force (or normal reaction), forces exerted during collision, spring force, tension in a string, forces of solid and fluid friction are all examples of contact force. A normal force is the force exerted by a surface against an object that is pressing against the surface.
• Contact forces are fundamentally electromagnetic. However, in the context of Newtonian mechanics, their electromagnetic origin is not an important concern.

Non-contact forces :

• They act without the necessity of physical contact between interacting objects.
• All four fundamental forces of nature, namely, gravitational, electromagnetic, strong and weak, are non-contact interactions.

Concept of pseudo force in a non-inertial frame of reference :

Suppose a particle of mass m has an acceleration gas measured in an inertial frame of reference S. Use of Newton's laws would correctly attribute this to a real force \(\vec{F}=m\vec{a}\) .

However, the particle is at rest in a frame S’ attached to it. Newton's laws can explain the state of rest of the particle in S’ provided a force —mg (in the opposite direction to the acceleration of the frame S’ is introduced. This pseudo force exists only in frame S’ and is measurable in S’. It originates not from any interaction with another body but purely from the acceleration of the frame of reference.

For example, when a vehicle or elevator is accelerating, a person at rest inside feels a force opposite to the direction of the person's accelerated frame of reference.

Some pseudo forces are given special names.

For example, if a particle is at rest in a frame S’ which is rotating with respect to S, then the force \(-m\vec{a}\)  in S’ is called the centrifugal force. If, in addition, the particle moves with a constant velocity in such a frame S’ then there is an additional sideways force on the particle called the Coriolis force.

All the above pseudo forces are also non-Newtonian forces because Newton's third law of motion does not apply to them — there is no reaction force to them, i.e., no equal and opposite force acting on some other body.

Work done by a constant force :

The work done on a body by a constant force (constant in both magnitude and direction) is defined to be the product of the magnitude of the displacement of the body times the component of the force parallel to the displacement.

Suppose a constant force  acting on a body displaces it by \(\vec{s}\). Then, the work done by the force is

W = \(\vec{F}.\vec{s}\) = (F cos θ)s

where θ is the smaller angle between |(\vec{F}\) and \(\vec{s}\) and  so that F cos θ is the component of the force in the direction of the displacement. In rectangular component form,

W = F_xs_x + F_ys_y + F_zs_z

• Work done is a scalar quantity.
• SI unit : the joule (J); 1 J = 1 N.m.
• CGS unit : the erg; 1 erg = 1 dyn.cm.
• Dimensions : [ML²T⁻²]

If the force is not in the direction of the displacement — for example, a body moving from point A to B acted on by a force \(\vec{F}\)  that varies in magnitude and direction (see below Fig.), then at every instant we must consider the component of the force iin the direction parallel to the displacement

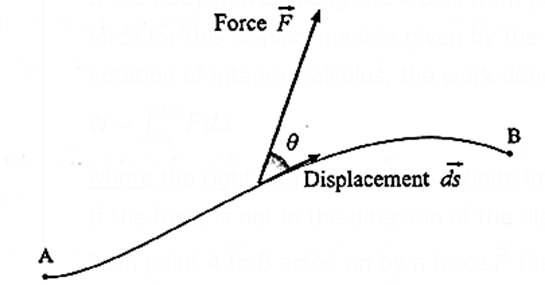
Let \(\vec{F}\) be the force at a point along the path, and let θ be the angle between \(\vec{F}\) and \(\vec{s}\) at this point. Then, the work done during the displacement \(\vec{ds}\) is

dW = (F cos θ) ds = \(\vec{F}.\vec{ds}\)

Then, W = \(\int \limits_{A}^{B} dW\) =\(\int \limits_{A}^{B}\vec{F}.\vec{ds}\)

This is the formula for calculating work done by variable force.

Expression for the power delivered by the force in terms of F, m and t when a constant force \(\vec{F}\)  acts on a body of mass m, initially at rest, for time t :

The power P delivered by a force is the time rate of work done by the force :

P = dW/dt

Under the action of a constant force \(\vec{F}\) if the displacement of the body in an infinitesimal time interval dt is \(\vec{ds}\) , the infinitesimal work done by the force in this time dt is

dW = \(\vec{F}.\vec{ds}\)

∴ P = \(\vec{F}.\frac{\vec{ds}}{dt}\) = \(\vec{F}.\vec{v}\) = Fv cos θ

Since F is constant, the acceleration \(\vec{a}=\frac{\vec{F}}{m}\)  is also constant.

∴ \(\vec{v}=\vec{u}+\vec{a}t\) = \(\vec{a}t\)  ('.'u = 0)

∴ \(\vec{v}= \frac{\vec{F}}{m}t\)

∴ P = \(\vec{F}.\frac{\vec{F}}{m}t\)=\(\frac{F^2m}{t}\) is the required expression.

Explanation :

For example, suppose only a conservative force facts on a particle as it moves from point A to point B along either path 1 or path 2, see above Fig.(a). Then the work done on the particle is the same along the two paths.

W_{AB, path1} = \(\int \limits_{A,path1}^{B}\vec{F}.\vec{ds}\)  = W_{AB, path2} = \(\int \limits_{A,path2}^{B}\vec{F}.\vec{ds}\)

If the particle moves from A and eventually returns to that position, i.e., the particle moves along a closed path beginning and ending at the initial position, then the total work done by the conservative force during the round trip along this and any other closed path is zero.

Thus, the net work done by a conservative force on a particle around every closed path is zero.

W _{closed path} = \(\oint \vec{F}.\vec{ds} \)

The above two equations are equivalent because any closed path is the sum of two paths : the first going from A to B, and the second going from B to A, Fig.(b), where

A and B are any two points on the closed path,

The work done in going along a path from B to A is the negative of the work done in going along the same path from A to B.

\(\oint \vec{F}.\vec{ds} \) = \(\int \limits_{A,path1}^{B}\vec{F}.\vec{ds}\) + \(\int \limits_{B,path2}^{A}\vec{F}.\vec{ds}\)

= \(\int \limits_{A,path1}^{B}\vec{F}.\vec{ds} - \int \limits_{A,path2}^{B}\vec{F}.\vec{ds}\)

In the field of a conservative force, the total energy of the particle — kinetic plus potential — at any two points is the same, i.e., it is conserved.

Concept of potential energy in the field of a conservative force :

Work done against a conservative force is stored in the sense that we can get it back again in the form of kinetic energy. We consider the ”stored work” as potential energy U, in the sense that it can be released as kinetic energy.

Changes in potential energy are all that ever matter physically. Hence, we define change in potential energy formally in terms of the work done by a conservative force. The change in potential energy associated with a conservative force is the negative of the work done by that force as it acts over any path from point A to point B :

ΔU_AB = \(-\int \limits_{A}^{B}\vec{F}.\vec{ds}\)

The minus sign indicates that if a conservative force does positive work (as does gravity on a falling object), then potential energy of the system must decrease, i.e., ΔU must be negative.

Conversely, if a conservative force does negative work (as does gravity on an object being lifted), then energy is stored in the system and potential energy must increase, i.e., ΔU must be positive.

When force and displacement are parallel or antiparallel, the above equation simplifies to

ΔU_AB = \(-\int \limits_{x_1}^{x_2}F(x)dx\)   where x₁ and x₂ are the starting and ending points on the x-axis, taken to coincide with the path. If the force is constant during this displacement,

ΔU_AB = −F(x₂ − x₁)

We usually drop the subscripts AB and write simply All for a change in potential energy.

Keeping the subscript is important, though, when we need to be clear about whether we are going from A to B or from B to A.

Since integration is the inverse operation of differentiation, we can as well start with the potential energy and take its derivative, with respect to displacement, to get the force. The infinitesimal increment of potential energy is the dot product of the force \(\vec{F}\) and the infinitesimal displacement \(d\vec{l}\)

dU = \(-\vec{F}.d\vec{l}\) = −F_l dl

where F_l, is the component of \(\vec{F}\) in the direction of the displacement.

This equation gives the relation between force and the potential energy associated with it.

That is, the component of a conservative force, in a particular direction, equals the negative of the derivative of the corresponding potential energy with respect to a displacement in that direction