Solutions-Practice Set-Class-10-Mathematics-2-Chapter-6-Trigonometry-Maharashtra Board

Given : sin θ = \(\frac{7}{25}\)

We have,

sin² θ + cos² θ = 1

∴ \((\frac{7}{25})^2\) + cos² θ = 1

∴ cos² θ = 1 - \(\frac{49}{625}\)

∴ cos² θ = \(\frac{625-49}{625}\) = \(\frac{576}{625}\)

∴ cos θ = \(\frac{24}{25}\)      ... (Taking square roots of both the sides)

tan θ = \(\frac{sin\,θ}{cos\,θ}\) = \(\frac{7/25}{24/25}\) = \(\frac{7}{25}×\frac{25}{24}\)

∴ tan θ = \(\frac{7}{24}\)

Answer is : cos θ = \(\frac{24}{25}\), tan θ = (\frac{7}{24}\)

Given : tan θ =

We have,

1 + tan² θ = sec² θ

∴ 1 + \((\frac{3}{4})^2\)  = sec² θ

∴ 1 + \(\frac{9}{16}\)  = sec² θ

∴ \(\frac{16+9}{16}\)  = sec² θ

∴ sec² θ = \(\frac{25}{16}\)

∴ sec θ = \(\frac{5}{4}\) ... (Taking square roots of both the sides)

cos θ = \(\frac{1}{sec\,θ}\) = \(\frac{1}{5/4}\)

∴ cos θ = \(\frac{4}{5}\)

Answer is : sec θ = \(\frac{5}{4}\), cos θ = \(\frac{4}{5}\)

Given : cot θ =

1 + cot² θ = cosec² θ

∴ 1 + \((\frac{40}{9})^2\) = cosec² θ

∴ 1 + \(\frac{1600}{81}\) = cosec² θ

∴ \(\frac{81+1600}{81}\) = cosec² θ

∴ cosec² θ = \(\frac{1681}{81}\)

∴ cosec θ = \(\frac{41}{9}\)  ... (Taking square roots of both the sides)

sin θ = \(\frac{1}{cosec\,θ}\) = \(\frac{1}{41/9}\)

∴ sin θ = \(\frac{9}{41}\)

Answer is : cosec θ = \(\frac{41}{9}\)  , sin θ = \(\frac{9}{41}\)

Given : 5 sec θ – 12 cosec θ = 0

∴ 5 sec θ = 12 cosec θ

∴ \(\frac{sec\,θ}{cosec\,θ}\) = \(\frac{12}{5}\)

∴ sec θ ÷ cosec θ = \(\frac{12}{5}\)

∴ \(\frac{1}{cos\,θ}÷\frac{1}{sin\,θ}\) = \(\frac{12}{5}\)       ….. [∵ sec θ = \(\frac{1}{cos\,θ}\) and cosec θ = \(\frac{1}{sin\,θ}\)  ]

∴ \(\frac{1}{cos\,θ}×\frac{sin\,θ}{1}\) = \(\frac{12}{5}\)

∴   \(\frac{sin\,θ}{cos\,θ} \) = \(\frac{12}{5}\)         ……(1)

∴ tan θ = \(\frac{12}{5}\)            ……(2)

1 + tan² θ = sec² θ

∴ 1 + \((\frac{12}{5})^2\) = sec² θ

∴ 1 + \(\frac{144}{25}\) = sec² θ

∴ \(\frac{25+144}{25}\) = sec² θ

∴ sec² θ = \(\frac{169}{25}\)

∴ sec θ = \(\frac{13}{5}\)             ……(3) ... (Taking square roots of both the sides)

cos θ = \(\frac{1}{sec\,θ}\)

∴ cos θ = \(\frac{1}{13/5}\) = \(\frac{5}{13}\)              ……(4)

\(\frac{sin\,θ}{cos\,θ} \) = \(\frac{12}{5}\)    ……[From (1)]

∴ sin θ = \(\frac{12}{5}\) × cos θ = \(\frac{12}{5}\) × \(\frac{5}{13}\)

∴ sin θ = \(\frac{12}{13}\)

Answer is : sec θ = \(\frac{13}{5}\), cos θ = \(\frac{5}{13}\) and sin θ = \(\frac{12}{13}\) 

Given : tan θ = 1

We know that tan 45° = 1

∴ θ = 45°

sin θ = sin 45° = \(\frac{1}{\sqrt{2}}\)

cos θ = cos 45° = \(\frac{1}{\sqrt{2}}\)

cosec θ = cosec 45° = \(\sqrt{2}\)

\(\frac{sin\,θ+cos\,θ}{sec\,θ+cosec\,θ}\) = \(\frac{sin\,45°+cos\,45°}{sec\,45°+cosec\,45°}\)

= \(\frac{\frac{1}{\sqrt{2}}+\frac{1}{\sqrt{2}}}{\sqrt{2}+\sqrt{2}}\)

= \(\frac{(\frac{2}{\sqrt{2}})}{2\sqrt{2}}\)

= \(\frac{2}{2\sqrt{2}\sqrt{2}}\) = \(\frac{1}{2}\)

Answer is : \(\frac{sin\,θ+cos\,θ}{sec\,θ+cosec\,θ}\) = \(\frac{1}{2}\)

Proof :

LHS = \(\frac{sin^2\,θ}{cos\,θ}\) + cos θ

= \(\frac{sin^2\,θ+cos^2\,θ}{cos\,θ}\)

= \(\frac{1}{cos\,θ}\)    ... (∵ sin² θ + cos² θ = 1)

= sec θ      ... (∵ \(\frac{1}{cos\,θ}\) = sec θ )

 ∴ LHS = RHS

∴ \(\frac{sin^2\,θ}{cos\,θ}\) + cos θ = sec θ

Proof :

LHS = cos² θ (1 + tan² θ)

= cos² θ × sec² θ            ... (∵ 1 + tan² θ = sec² θ )

= (cos θ x sec θ)²

= 1²    ... ( ∵ cos θ x sec θ = 1)

= 1

= RHS

∴ LHS = RHS

∴ cos² θ(1 + tan² θ) = 1.

Proof :

LHS = \(\sqrt{\frac{1-sin\,θ}{1+sin\,θ}}\)

= \(\sqrt{\frac{1-sin\,θ}{1+sin\,θ}×\frac{1-sin\,θ}{1-sin\,θ}}\)

= \(\sqrt{\frac{(1-sin\,θ)^2}{1^2-sin^2\,θ}}\)

 = \(\sqrt{\frac{(1-sin\,θ)^2}{cos^2\,θ}}\)   ….[∵ sin² θ +cos² θ = 1,  ∴ 1 - sin² θ = cos² θ]

= \(\frac{1-sin\,θ}{cos\,θ}\)

= \(\frac{1}{cos\,θ}-\frac{sin\,θ}{cos\,θ}\)

    = sec θ − tan θ   ….[∵ sec θ = \(\frac{1}{cos\,θ}\), tan θ = \(\frac{sin\,θ}{cos\,θ}\) ]

= RHS

∴ LHS = RHS

∴ \(\sqrt{\frac{1-sin\,θ}{1+sin\,θ}}\) = sec θ − tan θ

Proof :

LHS = (sec θ − cos θ)(cot θ + tan θ)

We know, sec θ = \(\frac{1}{cos\,θ}\) , cot θ = \(\frac{cos\,θ}{sin\,θ}\) and tan θ = \(\frac{sin\,θ}{cos\,θ}\)

∴ LHS  = \((\frac{1}{cos\,θ}-cos\,θ)\) \((\frac{cos\,θ}{sin\,θ}+\frac{sin\,θ}{cos\,θ}\)

= \((\frac{1-cos^2\,θ}{cos\,θ})\)\((\frac{cos^2\,θ+sin^2\,θ}{cos\,θ\,sin\,θ})\)

 =  \(\frac{sin^2\,θ}{cos\,θ}×\frac{1}{cos\,θ\,sin\,θ}\)     ….[∵ sin² θ + cos² θ = 1,  ∴  sin² θ = 1 - cos² θ]

 = \(\frac{sin\,θ}{cos\,θ}×\frac{1}{cos\,θ}\) = tan θ × sec θ   …[ ∵ \(\frac{sin\,θ}{cos\,θ}\) = tan θ and \(\frac{1}{cos\,θ}\) = sec θ]

= RHS

∴ LHS = RHS

∴ (sec θ − cos θ)(cot θ + tan θ) = tan θ sec θ  

Proof :

LHS = cot θ + tan θ

= \(\frac{cos\,θ}{sin\,θ}+\frac{sin\,θ}{cos\,θ}\)            …[∵ cot θ = \(\frac{cos\,θ}{sin\,θ}\)  and tan θ = ]

= \(\frac{cos^2\,θ+sin^2\,θ}{sin\,θ×cos\,θ}\)

= \(\frac{1}{sin\,θ×cos\,θ}\)           ….[∵ sin² θ +cos² θ = 1]

=\(\frac{1}{sin\,θ}×\frac{1}{cos\,θ}\)   = cosec θ × sec θ         …[∵ \(\frac{1}{sin\,θ}\) = cosec θ and \(\frac{1}{cos\,θ}\) = sec θ]

= RHS

∴ LHS = RHS

∴ cot θ + tan θ = cosec θ sec θ

Proof :

LHS = \(\frac{1}{sec\,θ-tan\,θ}\)

= \(\frac{1}{sec\,θ-tan\,θ}\) × \(\frac{sec\,θ+tan\,θ}{sec\,θ+tan\,θ}\)

= \(\frac{1(sec\,θ+tan\,θ)}{(sec\,θ-tan\,θ)(sec\,θ+tan\,θ)}\)

= \(\frac{sec\,θ+tan\,θ}{sec^\,θ-tan^\,θ}\)

= \(\frac{sec\,θ+tan\,θ}{1}\)                ….[∵ 1 + tan² θ = sec² θ,  ∴  sec² θ - tan² θ = 1]

= RHS

∴ LHS = RHS

∴ \(\frac{1}{sec\,θ-tan\,θ}\)  = sec θ + tan θ

Proof :

[Note : In the textbook the question is Prove sec⁴ θ − cos⁴ θ = 1 – 2 cos² θ, whereas it should be sin⁴ θ − cos⁴ θ = 1 – 2 cos² θ]

Proof :

LHS = sin⁴ θ − cos⁴ θ

= (sin² θ) ² - (cos² θ) ²

= (sin² θ + cos² θ) (sin² θ - cos² θ)      ... [a² - b² = (a + b)(a - b)]

= 1 (sin² θ - cos² θ)    ... (∵ sin² θ + cos² θ = 1)

= sin² θ - cos² θ

= 1 - cos² θ - cos² θ      ... [∵ sin² θ + cos² θ = 1. ∴ sin² θ = 1 - cos² θ]

= 1 - 2 cos² θ

= RHS

∴ LHS = RHS

∴ sin⁴ θ − cos⁴ θ = 1 – 2 cos² θ.

Proof :

LHS = sec θ + tan θ

= \(\frac{1}{cos\,θ}+\frac{sin\,θ}{cos\,θ}\)        …( ∵ sec θ =  and tan θ = )

= \(\frac{1+sin\,θ}{cos\,θ}\)

= \(\frac{(1+sin\,θ)(1-sin\,θ)}{cos\,θ(1-sin\,θ)}\)  ..[Multiplying the numerator and the denominator by (1-sin θ)]

= \(\frac{1^2-sin^2\,θ}{cos\,θ(1-sin\,θ)}\)

= \(\frac{cos^2\,θ}{cos\,θ(1-sin\,θ)}\)

= \(\frac{cos\,θ}{1-sin\,θ}\)

= RHS

∴ LHS = RHS

∴ sec θ + tan θ = \(\frac{cos\,θ}{1-sin\,θ}\)

tan θ + \(\frac{1}{tan\,θ}\) = 2     …(given) …(1)

Squaring both the sides, we get,

(tan θ + \(\frac{1}{tan\,θ}\))² = (2)²

∴ tan² θ + 2(tan θ)\(\frac{1}{tan\,θ}\) + \(\frac{1}{tan^2\,θ}\) = 4     ... [∵ (a + b)² = a² + 2ab + b²]

∴ tan² θ + 2 + \(\frac{1}{tan^2\,θ}\) = 4

∴ tan² θ + + \(\frac{1}{tan^2\,θ}\) = 4 - 2

∴ tan² θ + + \(\frac{1}{tan^2\,θ}\) =  2

Proof :

LHS = \(\frac{tan\,A}{(1+tan^2\,A)^2}\) + \(\frac{cot\,A}{(1+cot^2\,A)^2}\)

= \(\frac{tan\,A}{(sec^2\,A)^2}\) + \(\frac{cot\,A}{(cosec^2\,A)^2}\)  …. [∵ sec² A = 1 + tan² A, cosec² A = 1 + cot² A]

= \(\frac{tan\,A}{sec^4\,A}\) + \(\frac{cot\,A}{cosec^4\,A}\)

= (tan A ÷ sec⁴ A) + (cot A ÷ cosec⁴ A)

We know tan A = \(\frac{sin\,A}{cos\,A}\), sec A = \(\frac{1}{cos\,A}\), cot A = \(\frac{cos\,A}{sin\,A}\) and cosec A = \(\frac{1}{sin\,A}\)

∴ = \((\frac{sin\,A}{cos\,A}÷\frac{1}{cos^4\,A})\) + \((\frac{cos\,A}{sin\,A}÷\frac{1}{sin^4\,A})\)

= \((\frac{sin\,A}{cos\,A}×\frac{cos^4\,A}{1})\) + \((\frac{cos\,A}{sin\,A}×\frac{sin^4\,A}{1})\)

= sin A x cos³ A + cos A x sin³ A

= sin A x cos A (cos² A + sin² A)

=sin A x cos A(1) ... ( sin² A + cos² A = 1)

= sin A x cos A

= RHS

∴ LHS = RHS

∴ \(\frac{tan\,A}{(1+tan^2\,A)^2}\) + \(\frac{cot\,A}{(1+cot^2\,A)^2}\) = sin A cos A

Proof :

LHS = sec⁴A (1 − sin⁴A) − 2tan²A

= \(\frac{1}{cos^4\,A}\) × (1 − sin²A) (1 + sin²A) - 2\(\frac{sin^2\,A}{cos^2\,A}\)

= \(\frac{1}{cos^4\,A}\) × (cos²A) (1 + sin²A) - 2\(\frac{sin^2\,A}{cos^2\,A}\)     ….[∵ sin²A + cos²A = 1 ∴ 1- sin²A = cos²A]

= \(\frac{1+sin^2\,A}{cos^2\,A}-\frac{2\,sin^2\,A}{cos^2\,A}\)

= \(\frac{1+sin^2\,A-2\,sin^2\,A}{cos^2\,A}\)

= \(\frac{1-sin^2\,A}{cos^2\,A}\) = \(\frac{cos^2\,A}{cos^2\,A}\) = 1

(12) \(\frac{tan\,θ}{sec\,θ-1}\) = \(\frac{tan\,θ+sec\,θ+1}{tan\,θ+sec\,θ-1}\)

1 + tan² θ = sec² θ

∴ tan² θ = sec² θ - 1

∴ tan θ x tan θ = (sec θ + 1)(sec θ - 1)

∴ \(\frac{tan\,θ}{sec\,θ-1}\) = \(\frac{sec\,θ+1}{tan\,θ}\)

By theorem on equal ratios,

\(\frac{tan\,θ}{sec\,θ-1}\) = \(\frac{sec\,θ+1}{tan\,θ}\) = \(\frac{tan\,θ+sec\,θ+1}{sec\,θ-1+tan\,θ}\)

∴ \(\frac{tan\,θ}{sec\,θ-1}\) = \(\frac{tan\,θ+sec\,θ+1}{tan\,θ+sec\,θ-1}\)

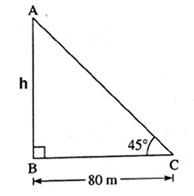
Let AB be the church and C be the position of the person from the church.

Suppose the height of the church AB = h m.

∠ ACB is the angle of elevation.

BC = 80 m, ∠ ACB = 45°   …(given)

In right angled Δ ABC,

tan 45° = \(\frac{AB}{BC}\) = \(\frac{h}{80}\)

∴ 1 = \(\frac{h}{80}\)     …( ∵ tan 45° = 1)

∴ h = 80 m

Answer is : The height of the church AB = 80 m.

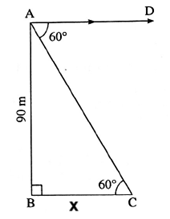
Let AB be the lighthouse and C be the position of the ship from the lighthouse.

Suppose the distance of the ship from the lighthouse be x m.

AD is the horizontal line and ∠ DAC is the angle of depression.

AB = 90 m and ∠ DAC = 60°              …(given)

 ∠ ACB and ∠ DAC are alternate angles.

∴ ∠ ACB = ∠ DAC = 60°.

In right angle Δ ABC,

tan 60° = \(\frac{AB}{BC}\) = \(\frac{90}{x}\)

∴ \(\sqrt{3}\) = \(\frac{90}{x}\)      …( ∵ tan 60° = \(\sqrt{3}\) )

∴ x = \(\frac{90}{\sqrt{3}}\) = \(\frac{90}{\sqrt{3}}×\frac{\sqrt{3}}{\sqrt{3}}\)  = 30

∴ x = \(\frac{90\sqrt{3}}{3}\) = 30\(\sqrt{3}\) = 30 x 1.73     ….( \(\sqrt{3}\) = 1.73)

∴ x = 51.9 m

Answer is: The ship is at the distance of 51.9 m from the lighthouse.

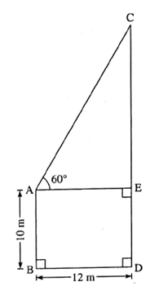
Let AB and CD be two building, with

AB = 10 m

And angle of elevation from top of AB to top of CD = ∠ CAE = 60°

Width of road = BD = 12 m

Clearly, ABDE is a rectangle

With

AB = ED = 10 m

BD = AE = 12 m

And AEC is a right-angled triangle, In Δ AEC

tan 60° = \(\frac{CE}{AE}\)       ...(By definition)

∴ \(\sqrt{3}\) = \(\frac{CE}{12}\)

∴ CE = 12\(\sqrt{3}\) m

Also,

CD = CE + ED = (12\(\sqrt{3}\) + 10) m

Hence, height of other building is (12\(\sqrt{3}\) + 10 m).

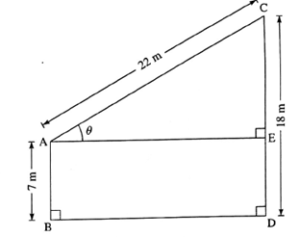
Let seg AB and seg CD represent the two poles.

AC represents the wire and seg AE ⊥ seg CD  ... C-E-D.

AB =7 m, CD = 18 m, AC = 22 m.

∠ CAE is the angle made by the wire with the horizontal.

Let ∠ CAE = θ.

□ ABDE is a rectangle.

DE = AB = 7 m          ... (Opposite sides of rectangle are equal)

CE + ED = CD ... (C-E-D)

∴  CE + 7 = 18

∴  CE = 18 - 7

∴ CE = 11 m

In right angled Δ CEA,

sin θ = \(\frac{CE}{AC}\)       ... (By definition)

∴ sin θ = \(\frac{11}{22}\) = \(\frac{1}{2}\)

We know that, sin 30° = \(\frac{1}{2}\)

∴ θ = 30°

Answer is: The angle made by the wire with the horizontal is 30°.

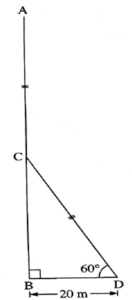
Let seg AB represent the tree before the storm.

Due to storm, tree gets broken at C. Broken part AC takes the position CD.

∴ AC = CD     ….(1)

BD = 20 m and ∠ CDB = 60°

In right angled Δ CBD,

tan 60° = \(\frac{CB}{BD}\)            ……(By definition)

∴ \(\sqrt{3}\) = \(\frac{CB}{20}\)          ………(tan 60° = \(\sqrt{3}\) )

CB = 20\(\sqrt{3}\) m

Also sin 60° = \(\frac{CB}{CD}\)         …..(By definitlon)

∴ \(\frac{\sqrt{3}}{2}\) = \(\frac{20\sqrt{3}}{CD}\)         ………(sin 60° = )

∴ CD = \(\frac{2×20\sqrt{3}}{\sqrt{3}}\)

∴ CD = 40 m

AC = CD = 40m   …..[From(1)]

AB = AC + CB       …..(A-C-B)

∴ AB = (40 + 20\(\sqrt{3}\)) m

Answer is : The height of the tree is (40 + 20\(\sqrt{3}\) ) m

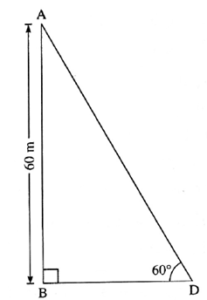
Let A represent the position of kite.

seg BD represent the surface of the ground.

seg AB is the height of the kite above the ground.

AB = 60 m.

AD represents the string.

∠ ADB is the angle made by the string with the ground.

∠ ADB = 60°

In right angled Δ ABD,

sin 60° = \(\frac{AB}{AD}\)       ….(By definition)

∴ \(\frac{\sqrt{3}}{2}\) = \(\frac{60}{AD}\)     ………(sin 60° = \(\frac{\sqrt{3}}{2}\))

∴ AD × \(\sqrt{3}\) = 60 × 2

∴ AD = \(\frac{60×2}{\sqrt{3}}\) = \(\frac{120×\sqrt{3}}{\sqrt{3}×\sqrt{3}}\) = \(\frac{120×\sqrt{3}}{3}\)

AD = 40\(\sqrt{3}\)

∴ AD = 40 x 1.73  ….(∵ \(\sqrt{3}\) = 1.732)

∴ AD = 69.2 m

Answer is : The length of the string is 69.2 m.