Solutions-Part-2
Solutions Part-2
|
Practice Set 2.4
Question 1. Compare the given quadratic equations to the general form and write values of a, b, c.
(1) x2 − 7x + 5 = 0
Comparing x2− 7x + 5 = 0 with the standard form ax2 + bx + c = 0 a = 1, b = −7, c = 5.
(2) 2m2 = 5m − 5
2m2 = 5m − 5 ∴ 2m2−5m + 5 = 0 Comparing 2m2−5m + 5 = 0 with the standard form ax2 + bx + c = 0 a =2, b = −5, c = 5.
(3) y2 = 7y
y2 = 7y ∴ y2 − 7y + 0 = 0 Comparing y2 − 7y + 0 with the standard form ax2 + bx + c = 0 a =1, b = −7, c = 0.
Question 2. Solve using formula.
(1) x2 + 6x + 5 = 0
x2 + 6x + 5 = 0 Comparing with ax2 + bx + c = 0 a = 1, b = 6, c = 5. b2 − 4ac = (6)2 − 4(1)(5) = 36 − 20 = 16 x = \(\frac{-b±\sqrt{b^2-4ac}}{2a}\) = \(\frac{-6±\sqrt{16}}{2×1}\) = \(\frac{-6±4}{2}\) ∴ x = \(\frac{-6+4}{2}\) or x = \(\frac{-6-4}{2}\) ∴ x = \(\frac{-2}{2}\) or x = \(\frac{-10}{2}\) ∴ x = −1 or x = −5 Answer is : −1, −5 are the roots of the given quadratic equation.
(2) x2 − 3x − 2 = 0
x2 − 3x − 2 = 0 Comparing with ax2 + bx + c = 0 a = 1, b = −3, c = −2. b2 − 4ac = (−3)2 − 4(1)(−2) = 9 + 8 = 17 x = \(\frac{-b±\sqrt{b^2-4ac}}{2a}\) = \(\frac{-(-3)±\sqrt{17}}{2×1}\) = \(\frac{3±\sqrt{17}}{2}\) ∴ x = \(\frac{3+\sqrt{17}}{2}\) or x = \(\frac{3-\sqrt{17}}{2}\) Answer is : \(\frac{3+\sqrt{17}}{2}\), \(\frac{3-\sqrt{17}}{2}\) are the roots of the given quadratic equation.
(3) 3m2 + 2m − 7 = 0
3m2 + 2m − 7 = 0 Comparing with ax2 + bx + c = 0 a = 3, b = 2, c = −7. b2 − 4ac = (2)2 − 4(3)(−7) = 4 + 84 = 88 m =\(\frac{-b±\sqrt{b^2-4ac}}{2a}\) = \(\frac{-2±\sqrt{88}}{2×3}\) = \(\frac{-2±\sqrt{4×22}}{6}\) = \(\frac{-2±2\sqrt{22}}{6}\) = \(\frac{-1±\sqrt{22}}{3}\) ∴ m = \(\frac{-1+\sqrt{22}}{3}\) or m = \(\frac{-1-\sqrt{22}}{3}\) Answer is : \(\frac{-1+\sqrt{22}}{3}\), \(\frac{-1-\sqrt{22}}{3}\) are the roots of the given quadratic equation.
(4) 5m2 − 4m − 2 = 0
5m2 − 4m − 2 = 0 Comparing with ax2 + bx + c = 0 a = 5, b = −4, c = −2. b2 − 4ac = (−4)2 − 4(5)(−2) = 16 + 40 = 56 m =\(\frac{-b±\sqrt{b^2-4ac}}{2a}\) = \(\frac{-(-4)±\sqrt{56}}{2×5}\) = \(\frac{4±\sqrt{4×14}}{2×5}\) = \(\frac{4±2\sqrt{14}}{2×5}\) = \(\frac{2±\sqrt{14}}{5}\) ∴ m = \(\frac{2+\sqrt{14}}{5}\) or m = \(\frac{2-\sqrt{14}}{5}\) Answer is : \(\frac{2+\sqrt{14}}{5}\), \(\frac{2-\sqrt{14}}{5}\) are the roots of the given quadratic equation.
(5) y2 + \(\frac{1}{3}\)y = 2
y2 + \(\frac{1}{3}\)y = 2 y2 + \(\frac{1}{3}\)y − 2 = 0 Comparing with ax2 + bx + c = 0 a = 1, b = \(\frac{1}{3}\), c = −2. b2 − 4ac = \((\frac{1}{3})^2\) − 4(1)(−2) = \(\frac{1}{9}\) + 8 = \(\frac{1+72}{9}\) = \(\frac{73}{9}\) y = \(\frac{-b±\sqrt{b^2-4ac}}{2a}\) = \(\frac{-\frac{1}{3}±\sqrt{\frac{73}{9}}}{2×1}\) = \(\frac{-\frac{1}{3}±\frac{1}{3}\sqrt{73}}{2}\) = \(\frac{-1±\sqrt{73}}{6}\) ∴ y = \(\frac{-1+\sqrt{73}}{6}\) or y = \(\frac{-1-\sqrt{73}}{6}\) Answer is : \(\frac{-1+\sqrt{73}}{6}\), \(\frac{-1-\sqrt{73}}{6}\) are the roots of the given quadratic equation.
(6) 5x2 + 13x + 8 = 0
5x2 + 13x + 8 = 0 Comparing with ax2 + bx + c = 0 a = 5, b = 13, c = 8. b2 − 4ac = (13)2 − 4(5)(8) = 169 − 160 = 9 x = \(\frac{-b±\sqrt{b^2-4ac}}{2a}\) = \(\frac{-13±\sqrt{9}}{2×5}\) = \(\frac{-13±3}{10}\) ∴ x = \(\frac{-13+3}{10}\) or x = \(\frac{-13-3}{10}\) ∴ x = \(\frac{-10}{10}\) or x = \(\frac{-16}{10}\) ∴ x = −1 or x = \(-\frac{8}{5}\) Answer is : −1, \(-\frac{8}{5}\) are the roots of the given quadratic equation.
(3) With the help of the flow chart given below solve the equation x2 + x + 3 = 0 using the formula.
Step 1 : Comparing x2 + \(2\sqrt{3}\)x + 3 = 0 with ax2 + bx + c = 0 ,a = 1, b = , c = 3. Step 2: b2 − 4ac = \((2\sqrt{3})^2\) − 4(1)(3) = 12 − 12 = 0 Step 3: x = \(\frac{-b±\sqrt{b^2-4ac}}{2a}\) .... (Formula) Step 4: x = \(\frac{-2\sqrt{3}±\sqrt{0}}{2×1}\) = \(\frac{-2\sqrt{3}}{2}\) = \(-\sqrt{3}\) Here, the value of b2 − 4ac = 0. the roots are real and equal Answer is : \(-\sqrt{3}\), \(-\sqrt{3}\) are the roots of the given quadratic equation.
Practice Set 2.5
Question 1. Activity : Fill in the gaps and complete.
(3) If a, b are roots of quadratic equation,
(3) If a, b are roots of quadratic equation,
Question 2. Find the value of discriminant.
(1) x2 + 7x − 1 = 0
x2 + 7x − 1 = 0 Comparing with ax2 + bx + c = 0 a = 1, b = 7, c = −1. Δ = b2 − 4ac = (7)2 − 4(1)(−1) = 49 + 4 = 53 Answers is : Δ = 53.
(2) 2y2 − 5y + 10 = 0
Comparing 2y2 − 5y + 10 = 0 with the standard form ax2 + bx + c = 0 a = 2, b = −5, c = 10 Δ = b2 − 4ac = (− 5)2 − 4(2)(10) = 25 − 80 = − 55 Answers is : Δ = − 55.
(3) \(\sqrt{2}\)x2 + 4x + 2\(\sqrt{2}\) = 0
Comparing x2 + 4x + 2 = 0 with the standard form ax2 + bx + c = 0 a = \(\sqrt{2}\), b = 4, c = 2\(\sqrt{2}\) Δ = b2 − 4ac = (4)2 − 4(\(\sqrt{2}\))( 2\(\sqrt{2}\) ) = 16 − 16 = 0 Answers is : Δ = 0.
Question 3. Determine the nature of roots of the following quadratic equations.
(1) x2 − 4x + 4 = 0
Comparing x2 − 4x + 4 = 0 with the standard form ax2 + bx + c = 0 a = 1, b = −4, c = 4 Δ = b2 − 4ac = (−4)2 − 4(1)(4) = 16 − 16 = 0 Here Δ = 0. ∴ The roots are real and equal
(2) 2y2 − 7y + 2 = 0
Comparing 2y2 − 7y + 2 = 0 with the standard form ax2 + bx + c = 0 a = 2, b = −7, c = 2 Δ = b2 − 4ac = (−7)2 − 4(2)(2) = 49 − 16 = 33 Here Δ > 0. ∴ The roots are real and unequal
(3) m2 + 2m + 9 = 0
Comparing m2 + 2m + 9 = 0 with the standard form ax2 + bx + c = 0 a = 1, b = 2, c = 9 Δ = b2 − 4ac = (2)2 − 4(1)(9) = 4 − 36 = −32 Here Δ < 0. ∴ The roots are not real
Question 4. Form the quadratic equation from the roots given below.
(1) 0 and 4
Let α = 0 and β = 4 Then α + β = 0 + 4 = 4 and αβ =0 × 4 = 0 The required quadratic equation is x2−( α + β)x + αβ = 0 i.e. x2 − 4x + 0 = 0 Answer is : x2 − 4x + 0 = 0 is the required quadratic equation
(2) 3 and −10
Let α = 3 and β = −10 Then α + β = 3 + (−10) = −7 and αβ = 3 × −10 = −30 The required quadratic equation is x2−( α + β)x + αβ = 0 i.e. x2 − (−7)x + (−30) = 0 i.e. x2 + 7x − 30 = 0 Answer is : x2 + 7x − 30 = 0 is the required quadratic equation
(3) \(\frac{1}{2}\), \(-\frac{1}{2}\)
Let α = \(\frac{1}{2}\) and β = \(-\frac{1}{2}\) Then α + β = \(\frac{1}{2}\) + (\(-\frac{1}{2}\) ) = 0 and αβ = \(\frac{1}{2}\) × \(-\frac{1}{2}\) = \(-\frac{1}{4}\) The required quadratic equation is x2−( α + β)x + αβ = 0 i.e. x2 − (0)x + (\(-\frac{1}{4}\)) = 0 i.e. x2 \(-\frac{1}{4}\) = 0 Answer is : x2 \(-\frac{1}{4}\) = 0 is the required quadratic equation
(4) 2 −\(\sqrt{5}\) , 2 +\(\sqrt{5}\)
Let α = 2 −\(\sqrt{5}\) and β = 2 +\(\sqrt{5}\) Then α + β = (2 − \(\sqrt{5}\) ) + (2 + \(\sqrt{5}\) ) = 4 and αβ = (2 − \(\sqrt{5}\) ) × (2 + \(\sqrt{5}\) ) = (2)2 − \((\sqrt{5})^2\) = 4 − 5 = −1 The required quadratic equation is x2−( α + β)x + αβ = 0 i.e. x2 − (4)x + (−1) = 0 i.e. x2 − 4x − 1 = 0 Answer is : x2 − 4x − 1 = 0 is the required quadratic equation
Question 5. Sum of the roots of a quadratic equation is double their product. Find k if equation is x2 − 4kx + k + 3 = 0
x2 − 4kx + k + 3 = 0 i.e. x2 − 4kx + (k + 3) = 0 Here, a = 1, b = −4k, c = k + 3 If α and β are the roots of the equation, α + β = 2αβ ….(Given) (1) α + β = \(-\frac{b}{a}\) = \(-\frac{-4k}{1}\) = 4k …....(2) αβ = \(\frac{c}{a}\) = \(\frac{k+3}{1}\) = k + 3 ∴ 2αβ = 2k + 6 …....(3) From (1), (2) and (3), 4k = 2k + 6 ∴ 4k − 2k = 6 ∴ 2k = 6 ∴ k = 3 Answer is : The value of k is 3.
Question 6. α , β are roots of y2 − 2y − 7 = 0 find,
(1) α2 + β2 (2) α3 + β3
The given equation is y2 − 2y − 7 = 0 Here, a = 1, b= −2, c = −7. α + β = \(-\frac{b}{a}\) = \(-\frac{-2}{1}\) = 2 …...(1) αβ = \(\frac{c}{a}\) = \(\frac{-7}{1}\) = −7 ...(2) (1) α2 + β2 = (α + β)2 − 2αβ = (2)2 − 2( − 7) ….[From (1) and (2)1 = 4 + 14 = 18. (2) α3 + β3 = (α + β)3 − 3αβ(α + β) =(2)3 −3(−7)(2) …..[From (1) and (2)1 = 8 + 42 = 50 Answer is : (1) α2 + β2 = 18 (2) α3 + β3 = 50.
Question 7. The roots of each of the following quadratic equations are real and equal, find k.
(1) 3y2 + ky + 12 = 0
3y2 + ky + 12 = 0 Here, a = 3, b = k, c = 12 Δ = b2 − 4ac = k2 − 4(3)(12) = k2 − 144 The roots are real and equal. (Given) ∴ Δ = 0 k2 − 144= 0 (k + 12)(k − 12) = 0 K + 12 = 0 or k − 12 = 0 K = −12 or k = 12 Answer is : The value of k is 12 or − 12.
(2) kx(x − 2) + 6 = 0
kx(x − 2) + 6 = 0 kx2 − 2kx + 6 = 0 …..(In the standard form) Here, a = k, b = −2k, c = 6 Δ = b2 − 4ac = ( −2k)2 − 4(k)(6) = 4k2 − 24k The roots are real and equal. …..(Given) ∴ Δ = 0 4k2 − 24k = 0 4k(k − 6) = 0 4k = 0 or k−6 = 0 ∴ k = 0 or k =6 k = 0 is unacceptable, because if k = 0, the coefficient of the quadratic term becomes zero. Hence, the equation will not be a quadratic equation. ∴ k= 6 Answer is : The value of k is 6.
Practice Set 2.6
Question 1. Product of Pragati’s age 2 years ago and 3 years(3 years from now ) hence is 84. Find her present age. [Note : The question has been modified.]
Let Pragati’s present age be x years. Her age 2 years ago was (x − 2) years and her age 3 years from now will be (x + 3) years. The numbers denoting the ages are (x − 2) and (x + 3). From the given condition, (x − 2)(x + 3) = 84 x(x+ 3) − 2(x+ 3) = 84 x2 +3x − 2x − 6 − 84 = 0 x2 + x − 90 = 0 x2 + 10x − 9x − 90 = 0 x(x + 10) − 9( x + 10) = 0 (x + 10)(x − 9) = 0 x + 10 = 0 or x − 9 = 0 X = −10 or x = 9 But the age cannot be negative. x = − 10 is unacceptable. ∴ x = 9 Answer is : Pragati’s present age is 9 years.
Question 2. Sum of squares of 2 consecutive natural even numbers is 244; find the numbers.
Let the two consecutive even natural numbers be x and x + 2. Then x2 + (x + 2)2 = 244 ∴ x2 + x2 + 4x + 4 − 244 = 0 ∴ 2x2 + 4x + 4 − 244 = 0 ∴ x2 + 2x − 120 = 0 ……(Dividing by 2) ∴ x2 + 12x − 10x − 120 = 0 ∴ x(x + 12) − 10(x + 12) = 0 ∴ (x + 12) (x − 10) = 0 ∴ x + 12 = 0 or x −10 = 0 But− 12 is not a natural number. ∴ 12 is unacceptable. ∴ x = 10 and x + 2 = 10 + 2 = 12 Answer is : The required two consecutive even natural numbers are 10 and 12.
Question 3. In the orange garden of Mr. Madhusudan there are 150 orange trees. The number of trees in each row is 5 more than that in each column. Find the number of trees in each row and each column with the help of following flow chart.
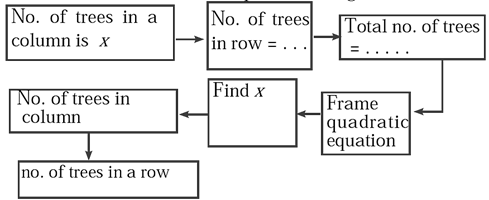
Let the number of trees in each column be x. Then the number of trees in each row = (x + 5). The total number of trees = x (x + 5). The total number of trees is given to be 150. ∴ x(x + 5) = 150 ∴ x2 + 5x – 150 = 0 ∴ x2 + 15x − 10x − 150 = 0 ∴ x(x + 15) − 10(x + 15) = 0 ∴ (x + 15)(x − 10) = 0 ∴ x + 15 = 0 or x – 10 = 0 ∴ x = − 15 or x = 10 But the number of trees cannot be negative. x = − 15 is unacceptable. ∴ x = 10 and x + 5 = 10 + 5 = 15 Answer is : The number of trees in each colmnn and each row is 10 and 15 respectively. The flow chart with answer is as follows
Question 4. Vivek is older than Kishor by 5 years. The sum of the reciprocals of their ages is 1/6. Find their present ages.
Let the present age of Kishor be x years. Then the present age of Vivek is (x + 5) years. The multiplicative inverses (reciprocals) of their ages are \(\frac{1}{x}\) and \(\frac{1}{x+5}\) respectively. From the given condition, \(\frac{1}{x}\) + \(\frac{1}{x+5}\) = \(\frac{1}{6}\) ∴ \(\frac{x+5+x}{x(x+5)}\) = \(\frac{1}{6}\) ∴ \(\frac{2x+5}{x^2+5x}\) = \(\frac{1}{6}\) ∴ 6(2x + 5) = 1(x2 + 5x) …..(By cross multiplication) ∴ 12x + 30 = x2 + 5x ∴ x2 + 5x = 12x + 30 ∴ x2 + 5x −12x – 30 = 0 ∴ x2 − 7x − 30 = 0 ∴ x2 − 10x + 3x − 30 = 0 ∴ x(x − 10) + 3(x − 10) = 0 ∴ (x − 10)(x + 3) = 0 ∴ x − 10 = 0 or x + 3 = 0 ∴ x = 10 or x = −3 But the age cannot be negative. ∴ x = − 3 is unacceptable. ∴ x = 10 and x + 5 = 10 + 5 = 15 Answer is : The present ages of Kishor and Vivek are 10 years end 15 years respectively.
Question 5. Suyash scored 10 marks more in second test than that in the first. 5 times the score of the second test is the same as square of the score in the first test. Find his score in the first test.
Let Suyash scored x marks in the first unit test. Then he scored (x + 10) marks in the second unit test. 5 times the marks in the second unit test = 5(x + 10) The square of the marks in the first unit test = x2. From the given condition, ∴ 5(x + 10) = x2 ∴ x2 = 5(x + 10) ∴ x2 = 5x + 50 ∴ x2 − 5x − 50 = 0 ∴ x2 −10x + 5x – 50 = 0 ∴ x(x − 10) + 5(x − 10) = 0 ∴ (x − 10)(x + 5) = 0 ∴ x − 10 = 0 or x + 5 = 0 ∴ x = 10 or x = −5 But the marks cannot be negative. ∴ x = − 5 is unacceptable. ∴ x = 10 Answer is : Suyash scored 10 marks in the first unit test
Question 6. Mr. Kasam runs a small business of making earthen pots. He makes certain number of pots on daily basis. Production cost of each pot is ` 40 more than 10 times total number of pots, he makes in one day. If production cost of all pots per day is 600, find production cost of one pot and number of pots he makes per day.
Let Kasam make x earthen pots per day Then the cost of each earthen pot is Rs. (10x + 40). Production cost of all pots per day is 600 ∴ x(10x + 40) = 600 ∴ 10x2 + 40x − 600 = 0 ∴ x2 + 4x − 60 = 0 …..(Dividing by 10) ∴ x2 + 10x − 6x − 60 = 0 ∴ x(x + 10) − 6(x + 10) = 0 ∴ (x + 10)(x − 6) = 0 ∴ x + 10 = 0 or x − 6 = 0 ∴ x = −10 or x = 5 But the number of earthen pots cannot be negative. ∴ x = − 10 is unacceptable. ∴ x = 6 The cost of each pot earthen pot = 10x + 40 = 10(6) +40 = 60 + 40 = 100 Answer is : He makes 6 earthen pots per day and the cost of each earthen pot is Rs. 100.
Question 7. Pratik takes 8 hours to travel 36 km downstream and return to the same spot. The speed of boat in still water is 12 km. per hour. Find the speed of water current.
The speed of the boat in still water is 12 km/h. (Given) Let the speed of the water current be x km/h. (Here, x < 12) Then the speed of the boat downstream = (12 + x) km/h and that in upstream = (12 − x) km/ h. Time = \(\frac{Distance}{Speed}\) Time to cover 36 km downstream is \(\frac{36}{12+x}\) hours and time to cover 36 km upstream is \(\frac{36}{12-x}\) hours. From the given condition, \(\frac{36}{12+x}\) + \(\frac{36}{12-x}\) = 8 ∴ \(36(\frac{1}{12+x} + \frac{1}{12-x})\) = 8 ∴ \(\frac{36(12-x+12+x)}{(12+x)(12-x)}\) = 8 ∴ 36(12 − x + 12 + x) = 8(12 + x)(12 − x) ∴ 36(24) = 8(144 − x2) ∴ 36 × 3 = 144 − x2 ….(Dividing both the sides by 8) ∴ 108 = 144 − x2 ∴ x2 = 144 − 108 ∴ x2 = 36 ∴ x = 6 or x = − 6 …..(Taking square root) But the speed cannot be negative. x = − 6 is unacceptable. ∴ x = 6
Answer is : The speed of the water current is 6 km/h
Question 8. Pintu takes 6 days more than those of Nishu to complete certain work. If they work together they finish it in 4 days. How many days would it take to complete the work if they work alone.
Let Nishu take x days to complete the work. Then Pintu takes (x + 6) days to complete the same work. In one day, Nishu does \(\frac{1}{x}\) part of the work and Pintu does \(\frac{1}{x+6}\) part of the work. ∴ in 1 day they together do \(\frac{1}{x}+\frac{1}{x+6}\) part of the work \(\frac{1}{x}+\frac{1}{x+6}\) = \(\frac{x+6+x}{x(x+6)}\) = \(\frac{2x+6}{x^2+6}\) ∴ total number of days taken by them together to complete the work is \(\frac{x^2+6}{2x+6}\) From the given condition, \(\frac{x^2+6}{2x+6}\) = 4 ∴ x2 + 6x = 4(2x + 6) ∴ x2 + 6x = 8x + 24 ∴ x2 + 6x − 8x − 24 = 0 ∴ x2 − 2x – 24 = 0 ∴ x2 − 6x + 4x – 24 = 0 ∴ x(x − 6) + 4(x − 6) = 0 ∴ (x − 6)(x + 4) = 0 ∴ x−6 = 0 or x + 4 = 0 ∴ x = 6 or x = −4 But the number of days cannot be negative. ∴ x = − 4 is unacceptable. ∴ x = 6 and x + 6 = 6 + 6 = 12 Answer is : Nishu takes 6 days and Pintu takes 12 days to complete the work individually.
Question 9. If 460 is divided by a natural number, quotient is 6 more than five times the divisor and remainder is 1. Find quotient and diviser.
Let the divisor be x. Then the quotient is (5x + 6) Dividend = divisor × quotient + remainder ∴ 460 = x(5x + 6) + 1 ∴ 460 = 5x2 + 6x + 1 ∴ 5x2 + 6x + 1 = 460 ∴ 5x2 + 6x + 1 – 460 = 0 ∴ 5x2 + 6x −459 = 0 ….( a×c = 5×(−459)=5 ×(−9×51) = −45×51 .. b = −45+51) ∴ 5x2 − 45x + 51x – 459 = 0 ∴ 5x(x − 9) + 51(x − 9) = 0 ∴ (x − 9)(5x + 51) = 0 ∴ x – 9 = 0 or 5x + 51 = 0 ∴ x = 9 or 5x = −51 ∴ x = 9 or x = \(-\frac{51}{5}\) But \(-\frac{51}{5}\) is not a natural number. ∴ x = \(-\frac{51}{5}\) is not acceptable. ∴ x = 9 And 5x + 6 = 5(9) + 6 = 45 + 6 = 51
Answer is : The quotient is 51 and the divisor is 9
Question 10.
In the adjoining fig. ABCD is a trapezium AB || CD and its area is 33 cm2. From the information given in the figure find the lengths of all sides of the ¨ ABCD. Fill in the empty boxes to get the solution.
¨ ABCD is a trapezium. AB || CD A (¨ ABCD) = (AB + CD) × [AM] ∴ 33 = (x + 2x + 1) × [x − 4] ∴ 66 = (3x + 1) × [x − 4] ∴ 3x2 – [21x] + [10x] – [70] = 0 ∴ 3x (x − 7) +10(x − 7) = 0 ∴ (3x +10)(x − 7) = 0 ∴ (3x +10) = 0 or [x – 7] = 0 ∴ x = −10/3 or x = [7] But length is never negative. ∴ x ≠ −10/3 ∴ x = [7] AB = 7 cm, CD = 15 cm , AD = BC = 5 cm
We reply to valid query.