Mechanical Properties of Solids
Maharashtra Board-Class-11-Science-Physics-Chapter-6
Solutions
Question 1. Choose the correct answer:
(i) Change in dimensions is known as…..
(A) deformation
(B) formation
(C) contraction
(D) strain.
Answer :
(A) deformation
(ii) The point on stress-strain curve at which strain begins to increase even without increase in stress is called….
(A) elastic point
(B) yield point
(C) breaking point
(D) neck point
Answer :
(B) yield point
(iii) Strain energy of a stretched wire is 18×10-3 J and strain energy per unit volume of the same wire and same cross section is 6×10-3 J/m3. Its volume will be....
(A) 3 cm3
(B) 3 m3
(C) 6 m3
(D) 6 cm3
Answer :
(B) 3 m3
(iv) ----- is the property of a material which enables it to resist plastic deformation.
(A) elasticity
(B) plasticity
(C) hardness
(D) ductility
Answer :
(C) hardness
(v) The ability of a material to resist fracturing when a force is applied to it, is called……
(A) toughness
(B) hardness
(C) elasticity
(D) plasticity.
Answer :
(A) toughness
Question 2. Answer in one sentence:
(i) Define elasticity.
Answer :
Elasticity is the property of a body or material which enables it to regain its original shape and size after removal of the deforming force.
(ii) What do you mean by deformation?
Answer :
A change in the shape or size or both of a body due to an external force is called deformation. [size ≡ volume]
(iii) State the SI unit and dimensions of stress.
Answer :
The SI unit of stress is the Pascal or the newton per square metre (Pa ≡ N/m2). The dimensions of stress are [L-1M1T-2].
(iv) Define strain.
Answer :
Strain is defined as the ratio of change in the physical dimensions of a stressed body to its original dimensions.
(v) What is Young’s modulus of a rigid body?
Answer :
A perfectly rigid body cannot be deformed by any external force. Hence, there is no question of stress, strain and Young's modulus in this case.
(vi) Why bridges are unsafe after a very long use?
Answer :
After extensive use, bridges acquire wear and tear and lose their elastic qualities. Hence, they become unsafe.
(vii) How should be a force applied on a body to produce shearing stress?
Answer :
To produce shear stress in a body, the applied force or its component should be tangential to the surface of the body.
(viii) State the conditions under which Hooke’s law holds good.
Answer :
Hooke's law holds good up to a certain point in deformation, where the applied force is minimal. In an elastic deformation, the atoms/molecules, of the body shift only a small fraction of an interatomic/intermolecular spacing in response to deforming forces and the internal restoring force is proportional to the strain produced by the applied force.
(ix) Define Poisson’s ratio.
Answer :
Within the elastic limit, the ratio of lateral strain to the linear strain is called Poisson’s ratio.
(x) What is an elastomer?
Answer :
A material which can be elastically stretched to a large value of strain is called an elastomer.
(xi) What do you mean by elastic hysteresis?
Answer :
In a material like vulcanized rubber, the material does not regain its original state immediately on removal of the applied force. Strain lags behind stress. This behaviour is called elastic hysteresis.
(xii) State the names of the hardest material and the softest material.
Answer :
- Hardest material : Diamond
- Softest material : Plasticine clay
(xiii) Define friction.
Answer :
The property which resists the relative motion between two surfaces in contact is called friction.
(xiv) Why force of static friction is known as ‘self-adjusting force’?
Answer :
Up to a certain limit, the force of static friction changes with the applied force such that the two forces are equal in magnitude and opposite in direction, keeping the body at rest Hence, the force of static friction is known as ‘self- adjusting force.
(xv) Name two factors on which the coefficient of friction depends.
Answer :
The coefficient of friction depends on the normal reaction between the surfaces in contact, the materials and nature of the surfaces.
Question 3. Answer in short:
(i) Distinguish between elasticity and plasticity.
Answer :
| Elasticity | Plasticity |
| Elasticity is the property of a body or material which enables it to regain its original shape and size after removal of the deforming force. | Plasticity refers to a body or material's ability to maintain its original shape and size even after deformation, preventing it from regaining its original shape and size. |
| Materials with strong internal restoring forces possess this property. | Materials with weak internal restoring forces possess this property. |
(ii) State any four methods to reduce friction.
Answer :
- Polishing the bearings
- Using a lubricant
- Using ball bearings
- Giving a body an aerodynamic shape
- Using antifriction metals.
(iii) What is rolling friction? How does it arise?
Answer :
Friction between two bodies in contact when one body rolls over the other, is called rolling friction. In this case, the point of contact between the surfaces keeps changing.
(iv) Explain how lubricants help in reducing friction?
Answer :
Lubricating moving parts with oil creates a thin oil film between them, reducing contact friction significantly. Hence, substituting contact friction with fluid friction greatly reduces the contact friction between the moving parts. Petroleum oils and greases are common lubricants.
Examples of solid lubricants : Graphite, molybdenum disulphide and talc.
(v) State the laws of static friction.
Answer :
(i) The limiting force of static friction (fs) is directly proportional to the magnitude of the normal reaction (N) between the two surfaces in contact: fs ∝ N.
(ii) The limiting force of static friction is independent of the apparent geometrical area between the surfaces in contact, so long as the normal reaction remains the same.
(iii) The limiting force of static friction depends upon materials in contact and the nature of their surfaces.
(vi) State the laws of kinetic friction.
Answer :
- The magnitude of the force of kinetic friction (fk) is directly proportional to the magnitude of the normal reaction (N) between the two surfaces in contact.
fs ∝ N.
- The force of kinetic friction is independent of shape and apparent area of the surfaces in contact.
- The force of kinetic friction depends upon the nature and material of the surfaces in contact.
- The magnitude of the force of kinetic friction is independent of the relative velocity between the object and the surface provided that the relative velocity is neither too large nor too small.
(vii) State advantages of friction.
Answer :
It is due to friction that,
- locomotion, such as walking or running, is possible
- machines work.
- a wheel can roll on a surface.
- we can hold objects.
(viii) State disadvantages of friction.
Answer :
Disadvantages of friction :
- Friction between moving parts of a machine or that between the tyres and road decreases the speed.
- The work done by frictional force is dissipated as heat. Thus, only a part of the input energy is available for useful work. Fuel is wasted in order to overcome the frictional force and maintain the desired speed.
- Friction leads to wear and tear of the surfaces in contact which are in relative motion.
- Friction produces noise.
(ix) What do you mean by a brittle substance? Give any two examples.
Answer :
A substance is said to be brittle if fracture occurs soon after the elastic limit.
Examples : glass and ceramics.
Question 4. Long answer type questions:
(i) Distinguish between Young’s modulus, bulk modulus and modulus of rigidity.
Answer :
| Young’s modulus | Bulk modulus | Modulus of rigidity |
| Young’s modulus is the modulus of elasticity associated with the change in length of a wire, rod or bar under normal stress. | Bulk modulus is the modulus of elasticity associated with the change in the volume of a material (solid, liquid or gas) with a change in hydrostatic pressure. | Modulus of rigidity is the modulus of elasticity associated with the change of shape of a body under shear stress. |
| It is the ratio of the longitudinal stress to the corresponding longitudinal strain. | It is the ratio of the volume stress to the corresponding volume strain. | It is the ratio of the shear stress to the corresponding shear strain. |
| In this case, the force is applied along the length of the wire, rod or bar. | In this case, the forces are applied normal to the surface of the body. | In this case, the force is applied tangential to the surface of the body. |
(ii) Define stress and strain. What are their different types?
Answer :
- Stress is defined as the net internal restoring force per unit area of the surface of a body over which the deforming force is applied.
- Strain is defined as the ratio of change in the physical dimensions of a stressed body to its original dimensions.
Types of stresses and corresponding strains :
- Normal or longitudinal stress, which changes the length of a body. Longitudinal strain is the resulting fractional change in length of the body.
- Volume stress, which changes the volume of a body without producing a change in its shape. Volume strain is the resulting fractional change in volume of the body.
- Shear stress, which changes the shape of a body. The corresponding strain is called the shear strain or shear.
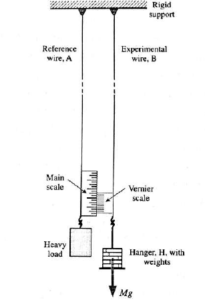
(iii) What is Young’s modulus? Describe an experiment to find out Young’s modulus of material in the form of a long straight wire.
Answer :
Determination of Young's modulus of the material of a long and thin wire :
Apparatus :
- The experimental wire ‘B’ is suspended from a rigid wall-support along
- with a compensating or reference wire ‘A’ of the same length, material and cross section.
- A main scale graduated in millimetre is attached to the wire ‘A’.
- A Vernier scale attached to the wire ‘B’ can move alongside the main scale.
Procedure :
(1) The length L and diameter d of the experimental wire are measured. The radius r (=\(\frac{1}{2}d\)) of the wire is calculated.
(2) Wire B is initially loaded with 1 kg to 2 kg to keep it taut and free from kinks. This load is treated as a zero-load. The main scale and Vernier scale readings are noted as those for zero-load.
(3) The stretching load Mg on wire B is increased by 0.5 kg at a time. The scale readings are noted for each increase in load, after allowing sufficient time for the wire to elongate.
(4) After 6-8 such readings, the wire is unloaded 0.5 kg at a time, and the corresponding scale readings are noted. Within the limits of experimental error, the readings for the same load—while loading and unloading—should be the same.
(5) The average elongation per unit mass, ΔL/M is found.
(6) Young's modulus of the material of the wire is calculated using the formula.
Y = \(\frac{longitudinal\,stress}{longitude\,strain} = \frac{Mg/πr^2}{ΔL/L}=\frac{gL}{πr^2(ΔL/M)}\)
(iv) Derive an expression for strain energy per unit volume of the material of a wire.
Answer :
Expression for strain energy per unit volume of the material of a wire :
Consider a uniform wire of length L and area of cross section A is suspended from a rigid support and stretched by applying a load Mg at the other end.
The internal restoring force gradually and uniformly increases from O to F (=Mg). During the time the wire stretches, at some instant, let the magnitude of the applied force be f and the elongation produced be x.
When fully stretched, x becomes l.
Young's modulus = \(\frac{\text{longitudinal stress}}{\text{longitudinal strain}}=\frac{f/A}{x/L}=\frac{fL}{Ax}\)
∴ f = \(\frac{YAx}{L}\) ….(1)
The work done by the force f, against the internal restoring force, in stretching the wire through a further infinitesimal length dx is
dW = fdx = \(\frac{YAx}{L}dx\)
The total work done in producing the elongation ΔL is
W = ∫ dW = \(\int_{0}^{ΔL}\frac{YAx}{L}dx\)= \(\frac{YA}{L}\int_{0}^{ΔL}x\,dx\)
= \(\frac{YA}{L}[\frac{x^2}{2}]_{0}^{ΔL}\)=\(\frac{YAΔL^2}{2L}\)=\(\frac{1}{2}(\frac{YAΔL}{L})ΔL\)
But Y = \(\frac{F/A}{ΔL/L}=\frac{Mg/A}{ΔL/L}=\frac{MgL}{AΔL}\)
∴ Maximum restoring force, F = Mg = \(\frac{YAΔL}{L}\)
Hence W = \(\frac{1}{2}FΔL=\frac{1}{2}MgΔL\) ….(2)
Thus, the total work done in stretching the wire = \(\frac{1}{2}\)stretching force x elongation.
The work done by the applied force in stretching the wire is stored in the stretched (or strained) wire as the potential energy which is then called the strain energy.
Thus, strain energy = \(\frac{1}{2}FΔL\) ….(3)
∴ Strain energy per unit volume of the stretched wire = \(\frac{\frac{1}{2}FΔL}{AL}\) = \(\frac{1}{2}(\frac{F}{A})(\frac{ΔL}{L})\)
= \(\frac{1}{2}\)(tensile stress).(tensile strain) ……(4)
= \(\frac{1}{2Y}\)(tensile stress)2 .....\(['.'Y=\frac{tensile\,stress}{tensile\,strain}]\)
Also, strain energy per unit volume
= \(\frac{1}{2}\)(tensile stress).(tensile strain = \(\frac{1}{2}Y\)(tensile strain)2
(v) What is friction? Define coefficient of static friction and coefficient of kinetic friction. Give the necessary formula for each.
Answer :
The property which resists the relative motion between two surfaces in contact is called friction.
The coefficient of static friction (μs) is defined as the ratio of the limiting force of
friction (fs) to the normal reaction (N) between the two surfaces in contact : μs = \(\frac{f_s}{N}\)
The coefficient of kinetic friction (μk) is defined as the ratio of the force of kinetic friction (fk) to the normal reaction (N) between the two surfaces in contact.
μk = \(\frac{f_k}{N}\)
(vi) State Hooke’s law. Draw a labeled graph of tensile stress against tensile strain for a metal wire up to the breaking point. In this graph show the region in which Hooke’s law is obeyed.
Answer :
Hooke's law: Within the elastic limit, the stress developed in a body is directly proportional to the strain produced in it.
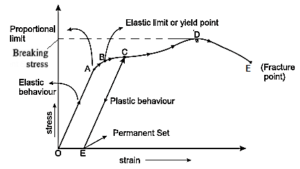
Fig. Graph of tensile stress against tensile strain for a metal wire up to the breaking point
Question 5. Answer the following
(i) Calculate the coefficient of static friction for an object of mass 50 kg laced on horizontal table pulled by attaching a spring balance. The force is increased gradually it is observed that the object just moves when spring balance shows 50N.
Answer :
Given : m = 50 kg, g = 9.8 m/s2, fs = 50N
fs = μs N = μsmg
μs = \(\frac{f_s}{mg}=\frac{50}{50×9.8}\) = 0.102
0.102 is the coefficient of static friction.
(ii) A block of mass 37 kg rests on a rough horizontal plane having coefficient of static friction 0.3 . Find out the least force required to just move the block horizontally.
Answer :
Given : m = 37 kg, μ s = 0.3, g = 9.8 m/s2,
Required force fs = μsmg = 0.3 x 37 x 9.8 = 108.8 N
108.8 N is the required force.
(iii) A body of mass 37 kg rests on a rough horizontal surface. The minimum
horizontal force required to just start the motion is 68.5 N. In order to keep the body moving with constant velocity, a force of 43 N is needed. What is the value of a) coefficient of static friction? and b) coefficient of kinetic friction?
Answer :
Given = m = 37kg, fs = 68.5 N, fk = 43 N, g = 9.8 m/s2
(a) μs = \(\frac{f_s}{mg}=\frac{68.5}{37×9.8}\) = 0.1889
0.1889 is the coefficient of static friction.
(b) μk = \(\frac{f_s}{mg}=\frac{43}{37×9.8}\) = = 0.1186
0.1186 is the coefficient of kinetic friction
(iv) A wire gets stretched by 4 mm due to a certain load. If the same load is applied to a wire of same material with half the length and double the diameter of the first wire. What will be the change in its length?
Answer :
Given : Δ L = 4 mm, L2 = \(\frac{L_1}{2}\), d2 = 2d1 , r2 = 2r1,
same load F, same Y
Y = \(\frac{FL}{πr^2ΔL}\) ∴ ΔL = \(\frac{FL}{πr^2Y}\)
ΔL1 = \(\frac{FL_1}{πr_1^2Y}\) and ΔL2 = \(\frac{FL_2}{πr_2^2Y}\)
∴ \(\frac{ΔL_1}{ΔL_2}=(\frac{L_2}{L_1})(\frac{r_1}{r_2})^2\) = \((\frac{1}{2})(\frac{1}{2})^2\) = \(\frac 18\)
ΔL2 = \(\frac{ΔL_1}{8}=\frac{4}{8}\) = 0.5mm
0.5 mm is the change in the length of the second wire.
(v) Calculate the work done in stretching a steel wire of length 2 m and cross = 0.0225 mm2 sectional area 0.0225 mm2 when a load of 100 N is slowly applied to its free end. [Young’s modulus of steel= 2 × 1011 N/m2 ]
Answer :
Given : L = 2m, F = 100 N,
A = 2.25 x 10-8 m2,
Y = 2 x 1011 N/m2
W = \(\frac{1}{2}\)FΔL, Y = \(\frac{FL}{AΔL}\) ∴ ΔL = \(\frac{FL}{AY}\)
W = \(\frac{1}{2}\)F x \(\frac{FL}{AY}\) = \(\frac{F^2L}{2AY}\)
= \(\frac{(100)^2(2)}{(2)(2.25×10^{-8})(2×10^{11})}\)
= \(\frac{20}{4×2.25}\) = 2.222 J
The work done in stretching the wire is 2.222 J.
(vi) A solid metal sphere of volume 0.31 m3 is dropped in an ocean where water pressure is 2 × 107 N/m2. Calculate change in volume of the sphere if bulk modulus of the metal is 6.1 × 1010 N/m2
Answer :
Given : v = 0.31 m3, p = 2 x 107 N/m2, K = 6.1 x 1010 N/m2
K = \(-\frac{p}{ΔV/V}\) = \(-\frac{pV}{ΔV}\)
ΔV = \(-\frac{pV}{K}\) = \(-\frac{(2×10^7)(0.31)}{6.1×10^{10}}\)
= \(-\frac{0.62}{6.1}×10^{-3}\)
= −0.1016 X 10−3
= −1.016 x 10-4 m3
The change (decrease) in the volume of the sphere is −1.016 x 10-4 m3 .
(vii) A wire of mild steel has initial length 1.5 m and diameter 0.60 mm is extended by 6.3 mm when a certain force is applied to it. If Young’s modulus of mild steel is 2.1 x 1011 N/m2, calculate the force applied.
Answer :
Given : L = 1.5 m, d = 0.60 mm, Δ L = 6.3 mm = 6.3 x 10-3m, Y = 2.1 x 1011 N/m2
r = \(\frac{d}{2}\) = 0.30mm = 3 x 10-4 m
Y= \(\frac{FL}{AΔL}\) ∴ F = \(\frac{YAΔL}{L}\) = \(\frac{Yπr^2ΔL}{L}\)
∴ F = \(\frac{2.1×10^{11}×3.14(3×10^{-4})^2×6.3×10^{-3}}{1.5}\)
= 2.1 x 3.142 x 6 x 6.3 = 249.4 N
The applied force is 249.4 N.
(viii) A composite wire is prepared by joining a tungsten wire and steel wire end to end. Both the wires are of the same length and the same area of cross section. If this composite wire is suspended to a rigid support and a force is applied to its free end, it gets extended by 3.25 mm. Calculate the increase in length of tungsten wire and steel wire separately.
[Given: Ysteel = 2 × 1011N/m2, Tungsten = 3.40 × 108 N/m2]
Answer :
Given : LT = LS, AT = AS, Δ LT + Δ LS = 3.25 mm, YT and YS are not given, F same
Y = \(\frac{FL}{AΔL}\), ∴ ΔL = \(\frac{FL}{AY}\)
ΔLT = \(\frac{FL_T}{AY_T}\) and ΔLS = \(\frac{FL_S}{AY_S}\)
\(\frac{ΔL_T}{ΔL_S}\) = \(\frac{Y_S}{Y_T}\) (as LT = LS and AT = AS) …(1)
ΔLT + ΔLS = 3.25mm …(2)
We have two equations and two unknown quantities (ΔLT and ΔLS) if the ratio \(\frac{Y_S}{Y_T}\) is known. Since the ratio is not given, ΔLT and ΔLS cannot be determined.
(ix) A steel wire having cross sectional area 1.2 mm2 is stretched by a force of 120 N. If a lateral strain of 1.455 mm is produced in the wire, calculate the Poisson’s ratio.
Answer :
Given : A = 1.2 mm2 = 1.2 x 10-6 m2, F = 120 N, lateral strain = 0.1455 mm = 1.455 x 10-4 m, YS = 2 x 1011 N/m2
Y = \(\frac{Fy}{AΔx}\)
∴ \(\frac{Δx}{y}\) = \(\frac{F}{AY}=\frac{120}{1.2×10^{-6}×2×10^{11}}\)
= \(\frac{100}{2×10^5}\) = 50 x 10-5 = 5 x 10-4 m
This is the linear strain produced in the wire.
Poisson’s ratio, σ = \(\frac{lateral\,strain}{linear\,strain}\) = \(\frac{1.455×10^{-4}}{5×10^{-4}}\) = 0.291
(x) A telephone wire 125 m long and 1mm in radius is stretched to a length 125.25 m when a force of 800 N is applied. What is the value of Young’s modulus for material of wire?
Answer :
Given : L = 125 m, r = 1 mm = 1 x 10-3 m, L+ Δ L = 125.25 m, F = 800 N
Δ L= 0.25 m
Y = \(\frac{FL}{AΔL}\) = \(\frac{FL}{πr^2ΔL}\) =
= \(\frac{800×125}{3.14×(1×10^{-3})^2×0.25}\) = \(\frac{40}{3.14}\)x 1010 = 12.73 x 1010 = 1.273 x 1011 N/m2
Value of Young's modulus for the material of the wire = 1.273 x 1011 N/m2
(xi) A rubber band originally 30 cm long is stretched to a length of 32 cm by certain load. What is the strain produced?
Answer :
Given : L1 = 30 cm, L2 = 32 cm
The strain produced in the rubber band = \(\frac{L_2-L_1}{L}\) = \(\frac{32-30}{30}\)
= 0.06667 = 6.667 x 10-2
(xii) What is the stress in a wire which is 50 m long and 0.01 cm2 in cross section, if the wire bears a load of 100 kg?
Answer :
Given : L = 50 m, A = 0.01 cm2 = 1 x 10-6m2, M = 100 kg, g = 9.8 m/s2
The stress in the wire = \(\frac{F}{A}\) = \(\frac{Mg}{A}\) = \(\frac{100×9.8}{1×10^{-6}}\) = 9.8 x 108 N/m2
(xiii) What is the strain in a cable of original length 50m whose length increases by 2.5 cm when a load is lifted?
Answer :
Given = L = 50m, ΔL = 2.5 cm = 2.5 x 10-2 m
Strain = \(\frac{ΔL}{L}=\frac{2.5×10^{-2}}{50}\) = 5 x 10-4
The strain produced in wire is 5 x 10-4
Main Page : – Maharashtra Board Class 11th-Physics – All chapters notes, solutions, videos, test, pdf.
Previous Chapter : Chapter 5: Gravitation – Online Solution
Next Chapter : Chapter 7: Thermal Properties of Matter – Online Solution
We reply to valid query.