Notes
Introduction :
- Changes can be physical or chemical and reversible or irreversible.
- Examples of natural irreversible processes : Natural waterfall, spreading of smoke from burning inscence stick, diffusion of fragrance of flowers, are irreversible physical changes.
Irreversible chemical reaction : A reaction which proceeds only in one direction, from reactants to products and the products do not have any tendency to reform the reactants is called an irreversible reaction.
- Irreversible reactions proceed until one of the reactants is exhausted.
- Their direction is indicated by an arrow (→) pointing towards the products.
Example :
(i) C(s) + O2(g) → CO2(g)
(ii) 2KClO3(s) \(\underrightarrow{Δ}\) 2KCl(s) + 3O2(g)
Reversible chemical reaction : Reversible reactions are those in which the reactants react to generate the products and the products have the tendency to react and regenerate the reactants.
- Reversible reactions proceed in both directions.
- The direction from reactants to products is the forward reaction whereas the opposite reaction from products to reactants is the reverse or backward reaction.
- The reversible reaction is denoted by a double arrow in opposite directions (⇌).
- Reversible reaction does not go to completion.
Examples :
(i) N2(g) + 3H2(g) ⇌ 2NH3(g)
(ii) In the below reaction (text book activity), the change in colour of the solution is caused by the chemical reaction which reverses its direction with change of temperature.
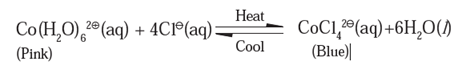
The reaction in the above activity is a reversible reaction.
Remember :
- In a closed system, there is no exchange of matter with the surroundings but exchange of heat can occur.
- In an open system, exchange of both matter and heat occurs with the surroundings. An isolated system does not exchange heat nor matter with surroundings.
Decomposition of CaCO, occurring in (a) an open system and (b) a container :
(a) In an open system, the decomposition of CaCO, is represented as,
CaCO3(s) \(\underrightarrow{heat}\) CaO(s) + CO2(g)
Since CO2(g) formed in the reaction escapes, this is a unidirectional irreversible reaction.
(b) In a closed container, the reaction goes in both opposite directions, hence it is a reversible reaction.
CaCO3(s) \(\underleftrightarrow{heat}\) CaO(s) + CO2(g)
Equilibrium in physical processes :
Physical equilibrium : The equilibrium attained between two phases of the same substance when the rates of forward and reverse changes are equal is called physical equilibrium.
(a) Liquid - Vapour equilibrium :
Consider evaporation of liquid water into water vapour in a closed vessel.
- Initially the vapour content above liquid in a closed vessel is very less.
- The liquid molecules have tendency to escape into vapour phase increasing vapour pressure.
- The vapour molecules have a tendency to condense. Hence, it is a reversible process.
H2O(l) ⇌ H2O (vapour)
- At start, the rate of evaporation is high and the rate of condensation is low. But with times, the rate of evaporation decreases and the rate of condensation increases.
- After a certain time, both the opposite rates become equal achieving an equilibrium state.
Saturated vapour pressure or aqueous tension : The pressure exerted by the water molecules in the vapour at equilibrium at a given temperature over the liquid water is called saturated vapour pressure or aqueous tension.
Effect of temperature :
- As the temperature increases, the saturated vapour pressure or aqueous tension increases.
- In case of water, the saturated vapour pressure is 1.013 bar or 1 atm at 100 °C, which is referred to as a boiling point.
(b) Solid - liquid equilibrium :
- Consider a mixture of ice and water in a perfectly insulated thermos flask at 273 K.
Ice(s) ⇌ water(l)
- In this, solid ice exists in equilibrium with water. The slight increase or decrease in temperature, shifts the equilibrium in forward or backward direction.
(c) Solid - vapour equilibrium :
The examples of solid-vapour equilibrium :
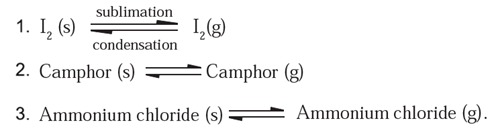
Explanation :
- Place some iodine crystals in a closed glass vessel.
- After some time the vessel gets filled up with violet vapour of iodine and its intensity increases with time.
- The intensity of violet colour becomes stable after certain time which indicates a state of equilibrium between solid and vapour iodine is reached.
Saturated solution : Saturated solution is the solution when additional solute cannot be dissolved in it at the given temperature. The concentration of solute in a saturated solution depends on temperature.
Equilibrium in chemical processes :
Chemical equilibrium : When there is no further change in concentration of reactant and product, we say that the reaction has attained equilibrium, with the rates of forward and reverse reactions being equal.
- Example : N2(g) + 3H2(g) ⇌ 2NH3(g)
Explanation :
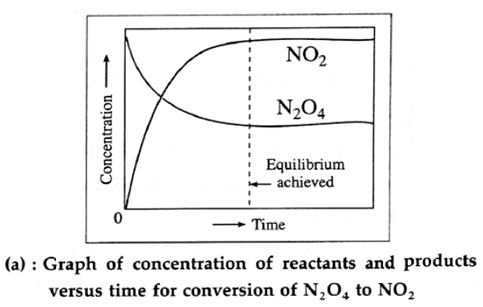
For a reversible reaction N2O4(g) ⇌ 2NO2(g)
(i) Variation in concentration of N2O4(g) and NO2(g)with time :
As the time passes, the concentration of N2O4 decreases while the concentration of NO2 increases.
(ii) variation in forward rate and backward rate of the reaction with time :
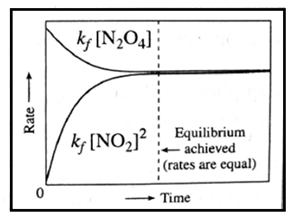
- As the time passes, the concentration of N2O4(g) decreases while the concentration of NO2(g) increases, hence the rate of decomposition of N2O4 decreases but the rate of formation of NO2
- A stage reaches when both the opposite rates become equal and a chemical equilibrium is attained.
Remember : For any reversible reaction in a closed system the rate of forward reaction is high in the beginning, while the rate of reverse reaction is very slow. As the forward reaction proceeds and products accumulate, rate of forward reaction slows down while rate of reverse reaction increases. Finally the rates become equal and equilibrium is established.
Law of mass action and equilibrium constant :
Equilibrium constant (KC) : The chemical equilibrium is mathematically described in terms of equilibrium constant (KC).
Mathematical Expression for equilibrium constant KC :
Consider a reversible reaction,
A(g) + B(g) ⇌ C(g) + D(g)
Rate of forward reaction is,
rf ∝ [A] x [B]
∴ rf = kf [A] x [B]
Rate of backward reaction (rb) is,
rb ∝ kb [C] x [D]
rb = kb [C] x [D]
where kf and kb are rate constants for forward and backward reactions.
At equilibrium, Rf = Rb
kf [A] x [B] = kb [C] x [D]
∴ \(\frac{k_f}{k_b}=\frac{[C]×[D]}{[A]×[B]}\) since kf and kb are constant.
\(\frac{k_f}{k_b}\) = kC
∴ kC = \(\frac{[C]×[D]}{[A]×[B]}\)
where kC is equilibrium constant.
Rate of chemical reaction :
- The rate of reaction can be determined by measuring the extent to which the concentration of a reactant decreases in the given time interval, or extent to which the concentration of a product increases in the given time interval.
Rate = — d[Reactant]/dT = d[Product]/dT
Law of mass action :
Statement : The law of mass action states that the rate of a chemical reaction is directly proportional to the product of the active masses of the reactants, with each active mass term raised to a power equal to its respective stoichiometric coefficient in the balanced equation at a given temperature.
Explanation :
Consider a general equation,
aA + bB → Products
If [A] and [B] are the active masses i.e., molar concentrations of the reactants A and B respectively, then by a law of mass action,
Rate ∝ [A]a x [B]b
Hence,
Rate = k [A]a x [B]b
This equation is called rate equation.
The proportionality constant k is known as rate constant or specific reaction rate for the reaction.
Expression for KC for the reaction :
Consider the reversible chemical reaction
aA + bB ⇌ cC + dD
Where A, B are the reactants and C, D are the products, while a, b, c and d are stoichiometric coefficients of the corresponding substances.
By the law of mass action, the rate of the forward reaction (rf) is,
rf ∝ [A]a x [B]b and
rf = kf [A]a x [B]b
where kf is the rate constant for the forward reaction.
The rate of the reverse or backward reaction (rb) is,
rb ∝ [C]c x [D]d and
rt = kb [C]c x [D]d
where kb is the rate constant for the backward reaction.
At equilibrium, the rate of forward and backward reactions are equal.
∴ rf = rb
∴ kf [A]a x [B]b = kb [C]c x [D]d
∴ \(\frac{k_f}{k_b}=\frac{[C]^c×[D]^d}{[A]^a×[B]^b}\)
The ratio kf / kb = Kc is constant called equilibrium constant of the reversible reaction.
Hence, the expression for KC is,
KC = \(\frac{[C]^c×[D]^d}{[A]^a×[B]^b}\)
Examples :
Equilibrium that occurs in the Haber process for synthesis of ammonia:
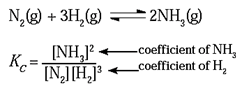
and for the equilibrium reaction written as
2NH3(g) ⇌ N2(g) + 3H2(g),
KC = \frac{[N_2]×[H_2]^3}{[NH_3]^2}\)
Equilibrium constant with respect to partial pressure (KP) :
Consider a gaseous reversible reaction,
aA(g) + bB(g) ⇌ cC(g) + dD(g)
For gases, the active mass or concentration is expressed in partial pressures. Hence, the equilibrium constant KP is represented as,
KP = \(\frac{[P_C]^c×[P_D]^d}{[P_A]^a×[P_B]^b}\)
where PA, PB, PC and PD are the equilibrium partial pressures of A, B, C and D respectively.
Relationship between partial pressure (KP) and concentration (KC) :
Consider the equilibrium constants designated as KP and KC for the concentrations
expressed in pressures and molar concentrations respectively.
Consider the following gaseous reversible reaction,
aA(g) + bB(g) ⇌ cC(g) + dD(g)
Then, KP = \(\frac{[P_C]^c×[P_D]^d}{[P_A]^a×[P_B]^b}\)
where PA, PB, PC and PD are the equilibrium partial pressures of A, B, C and D respectively.
The partial pressure, p of each component is directly proportional to its molar concentration.
If gaseous equilibrium mixture contains nA, nB, nC and nD moles of A, B, C and D respectively in a vessel of volume V, then the molar concentrations are, [A] = nA/V [B] = nB/V, [C] = nC/V, [D] = nD/V mol L-1
By the general gas equation,
pAV = nART,
∴ pA = \(\frac{n_A}{V}RT\) = [A]RT
Similarly,
pB = [B]RT, pC = [C]RT and pD = [D]RT
Since,
KP = \(\frac{[p_C]^c×[p_D]^d}{[p_A]^a×[p_B]^b}\) = \(\frac{([C]RT)^c×([D]RT)^d}{([A]RT)^a×([B]RT)^b}\)
= \(\frac{[C]^c×[D]^d}{[A]^a×[B]^b}×\frac{(RT)^{c+d}}{(RT)^{a+b}}\)\)
= \(\frac{[C]^c×[D]^d}{[A]^a×[B]^b}× (RT)^{(c+d)-(a+b)}\)
= \(\frac{[C]^c×[D]^d}{[A]^a×[B]^b}× (RT)^{Δn}\)
Since,
KC = \(\frac{[C]^c×[D]^d}{[A]^a×[B]^b}\)
∴ KP = KC (RT)Δn
where, Δn = (c + d) — (a + b)
= [Number of moles of gaseous products]—[Number of moles of gaseous reactants]
= n(g)(products) — n(g)(reactants)
(R is a gas constant where R = 0.08206 L atmK-1 mol-1)
Homogeneous and Heterogeneous equilibria :
Homogeneous reactions : In this equilibrium, the physical states of all the reactants and products are in the one homogeneous phase.
For example, 2HI(g) ⇌ H2(g) + I2(g)
Heterogeneous reactions : Reversible reaction involving reactants and products those are in different phases is called heterogeneous reaction.
For example, NH3(g) + Cl2(g) ⇌ NH4Cl(s)
- Equilibria involving homogeneous or heterogeneous reactions are called homogeneous and heterogeneous equilibria respectively.
Remember : Heterogeneous equilibrium involves the substances at equilibrium in different phases. For solids and pure liquids the active mass or concentration is assumed constant.
For example, NH3(g) + Cl2(g) ⇌ NH4Cl(s)
K = \(\frac{[NH_4Cl]}{[NH_3]×[Cl_2]}=\frac{1}{[NH_3]×[Cl_2]}\)
Units of equilibrium constant
The unit of equilibrium constant depends upon the expression of KC which is different for different equilibria. Therefore, the unit of KC is also different.
To calculate units of equilibrium constant :
To calculate units of KC find out the difference between the number of moles in the numerator and the number of moles in the denominator in the expression for equilibrium constant.
Examples :
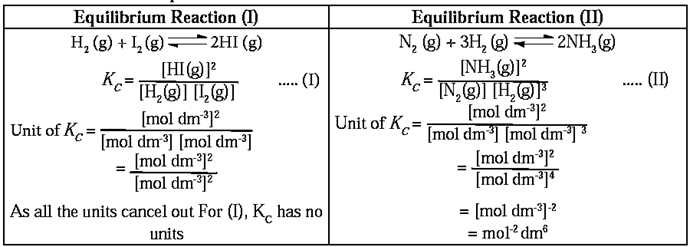
Remember :
- At equilibrium the ratio of product multiplicative term denoting the ratio of concentration of products to that of the reactants is unchanged and equals KC. The value of KC depends upon the temperature.
- It is interesting to note that though the concentration ratio remains unchanged, both the forward as well as reverse reactions do proceed at equilibrium, but at the same rate.
- Therefore, the chemical equilibrium is a dynamic equilibrium.
Characteristics of equilibrium constant :
- The value of equilibrium constant is independent of initial concentrations of either the reactants or products.
- Equilibrium constant is temperature dependent. Hence KC, KP change with change in temperature.
- Equilibrium constant has a characteristic value for a particular reversible reaction represented by a balanced equation at the given temperature.
- Higher value of KC or KP means more product is formed and the equilibrium point is more towards right hand side and vice versa.
Application of equilibrium constant :
Some applications of equilibrium constant are discussed below.
(1) Prediction of the direction of the reaction :
For the reversible reaction,
aA + bB ⇌ cC + dD,
the equilibrium constant is
KC = \(\frac{[C]^c×[D]^d}{[A]^a×[B]^b}\)
where all concentrations are equilibrium concentrations.
The ratio is called reaction quotient, QC, when the concentrations are not necessarily
equilibrium concentrations.
QC = \(\frac{[C]^c×[D]^d}{[A]^a×[B]^b}\)
- When QC < KC : This indicates, equilibrium is not reached. It implies that the reaction will proceed from left to right i.e., reaction will go forward until the equilibrium state is established.
- When QC > KC: This indicates, the concentrations are not at equilibrium. It also implies that concentrations of the products must decrease and that of the reactants must increase. Hence, such a reaction will proceed backward until the equilibrium state is established.
- When QC = KC: This indicates the reaction is at equilibrium, hence the concentrations of the reactants and the products are the equilibrium concentrations. Therefore, there will be no change in the concentrations of the reactants and the products with time.
(2) To know the extent of a reaction : The magnitude of the equilibrium constant indicates the extent of conversion of the reactants into products.
- If Kc is very large (generally, Kc > 103), then the reaction goes nearly to completion.
- If KC is very small (generally, Kc < 10-3), then the reactants react to a very less extent and at equilibrium the concentration of the reactants is much higher than that of the products.
- If Kc is intermediate having value between 10-3 and 103 then at equilibrium the concentrations of the reactants and the products present at equilibrium are msignificant.
(3) To calculate equilibrium concentrations : From the value of equilibrium constant and by knowing all the other equilibrium concentrations except one, the unknown concentration can be calculated.
Link between chemical equilibrium and chemical kinetics :
If KC is equilibrium constant for a reversible reaction then,
KC = kf/kr
where kf and kr are velocity or rate constants of the forward and reverse reactions respectively.
This equation can be used to determine the composition of the reaction mixture.
| kf > kr | kf ≈ kr |
| KC is very large | kf and kr have comparable values
KC is nearly one. |
| Reaction goes almost to completion. | Reaction never goes to completion. |
| If kf is much larger than KC, the reaction may be irreversible (Reverse reaction is too slow to be detected). | Comparable c0ncentrations of reactants and products are present at equilibrium. |
Remember : The equilibrium refers to the relative amounts of reactants and products and thus a shift in equilibrium in a particular direction will imply the reaction in that direction will be favoured.
Le Chaterlier's principle and factors altering the composition at equilibrium :
Principal goal of chemical synthesis : The principal goal of chemical synthesis is to achieve maximum conversion of reactants to products with minimum expenditure of energy.
Le Chatelier's Principle : It states that, when a system at equilibrium is subjected to a change in any of the factors determining the equilibrium conditions, system will respond in such a way as to minimize the effect of change.
Factors affecting equilibrium :
Le Chatelier's principle explains the various factors affecting chemical equilibrium.
(a) Effect of change in concentration : At equilibrium, all the reactants and the products present are at equilibrium concentrations.
Consider the following reaction at the equilibrium state.
Na2(g) + 3H2(g) ⇌ 2NH3(g)
At equilibrium QC = KC
(i) Addition of the reactants : By the addition of one or more reactants, (H2, N2) a stress is applied of the increase in the concentration of the reactants and QC < KC.
According to Le Chatelier’s principle, this stress will be reduced by shitting the equilibrium forward by decreasing the concentration of the reactants and increasing the concentration of the products (NH3).
- Addition of products : By addition of the products (NH3), a stress is applied to the chemical equilibrium on the right hand side and QC > Kc. This stress will be reduced by shifting the equilibrium backward thus by decreasing the concentration of the products.
- Removal of the reactants : By removal of the reactants (N2 or H2), the stress produced will be reduced by shifting the equilibrium backward, by decreasing the concentrations of the products.
- Removal of the products : By removal of the products (NH3), the stress produced will be reduced by shifting the equilibrium forward, by converting more reactants into the products.
(b) Effect of Pressure and Volume :
Chemical equilibrium involving only gaseous reactants and products is influenced by the changes in pressure and volume. Concentrations of solids and liquids are not affected by changes in pressure and volume.
This influence depends on the change in number of moles (Δn) in the reaction where,
Number of
Δn = [Number of moles of gaseous products] — [Number of moles of gaseous reactants]
(i) If Δn = 0 : Consider the following gaseous reaction,
H2(g) + I2(g) ⇌ 2HI(g)
Δn = [nHI]—[nH2+nI2] = 2 — (1 + 1) = 0
The composition of equilibrium mixture is not affected by the changes in pressure and volume.
(ii) If Δn > 0 : Consider the following gaseous reaction,
PCl5(g) ⇌ PCl3(g) + Cl2(g)
Δn = (nPCL3 + nCl2) — (nPCl5)
= (1 + 1) — 1
= 1 mol
By Le Chatelier’s principle decrease in pressure or increase in volume will shift the equilibrium forward giving more products.
(iii) If Δn < 0: Consider the following gaseous reaction,
N2(g) + 3H2(g) ⇌ 2NH3(s)
Δn = (nNH3) —(nN2 + nH2)
= 2 — (1 + 3)
= — 2 moi
By Le Chatelier's principle, the increase in pressure or decrease in volume will shift the equilibrium forward increasing the concentration of products.
Remember :
- A reaction in which decrease in volume takes place, reaction will be favoured by increasing pressure and the reaction with increase in volume will be favoured with lowering pressure, temperature being constant.
- In a reversible reaction, the reverse reaction has an energy change that is equal and opposite to that of the forward reaction.
(c) Effect of Temperature :
(i) For endothermic reaction equilibrium :
Consider the following endothermic reaction,
N2(g) + O2(g) ⇌ 2NO(g) — 79.9 kJ
- In this equilibrium, forward reaction is endothermic while backward reaction is exothermic.
- By increasing the temperature, according to Le Chatelier’s principle, the equilibrium will be shifted to the forward endothermic direction giving more products. Hence, the endothermic reaction is favoured at high temperature.
(ii) For exothermic reaction equilibrium :
Consider the following exothermic reaction,
PCl5(g) ⇌ PCl3(g) + Cl2(g) + 95.5 kJ
- In this equilibrium, the forward reaction is exothermic, while backward reaction is endothermic.
- By increasing the temperature, heat is added to a reaction system at equilibrium. This applies a stress to the system. According to Le Chatelier’s principle, the equilibrium is shifted to the backward endothermic reaction absorbing the heat which decreases a stress. But decrease in temperature will shift the equilibrium forward exothermic reaction yielding more products PCl3(g) and Cl2(g).
- Hence exothermic reaction is favoured at low temperature.
(d) Effect of a catalyst on equilibrium :
- An addition of a catalyst to the reaction system at equilibrium increases the rates of forward and backward reactions equally.
- The position of equilibrium is not affected by a catalyst.
- A catalyst does not change the composition of the equilibrium mixture.
- The value of the equilibrium constant does not change in the presence of a catalyst.
- Catalyst only helps to attain the equilibrium state faster.
- For example, CH3COOH(l) + C2H5OH(l) ⇌ CH3COOC2H5(l) + H2O(l)
Summary of effects of all four factors on the position of equilibrium and value of KC :
| Effect of | Position of equilibrium | Value of KC |
| Concentration | Changes | No change |
| Pressure | Changes if reaction involves change in number of gas molecules | No change |
| Temperature | Changes | Changes |
| Catalyst | No change | No change |
Remember : In all the cases of change in concentration, pressure, temperature and presence of catalyst, once the equilibrium has been re-established after the change, the value of KC will be unaltered
Industrial Application :
The Haber process : (Industrial preparation of ammonia)
The Haber process is the process of synthesis of ammonia gas by reacting together
hydrogen gas and nitrogen gas in a particular stoichiometric ratio by volumes and at selected optimum temperature.
N2(g) + 3H2(g) \(\underleftrightarrow{Fe}\) 2NH3(g) + Heat
- Iron (Fe) (containing a small quantity of molybdenum) is used as catalyst.
(i) Effect of concentration : By increasing the concentration of the reactants N2(g) and H2(g) the reaction proceeds forward increasing the yield of NH3(g).
(ii) Effect of temperature : Since the formation of NH3(g) is an exothermic reaction, it is carried out at high temperature. The optimum temperature is 773 K.
(iii) Effect of pressure :
For this reversible reaction,
Δn = (n2)product — (n1)reactant = 2 —(1 + 3)= -2 mol
Since during the formation of ammonia, there is a decrease in number of moles, the reaction is carried out at high pressure. The optimum pressure required is about 500 atm.
