Solutions
Maharashtra Board-Class-12-Chemistry-Chapter-2
Solution
Question 1.
Choose the most correct option.
(i) The vapour pressure of a solution containing 2 moles of a solute in 2 moles of water (vapour pressure of pure water = 24 mm Hg) is
(a) 24 mm Hg
(b) 32 mm Hg
(c) 48 mm Hg
(d) 12 mm Hg
(d) 12 mm Hg
(ii) The colligative property of a solution is
(a) vapour pressure
(b) boiling point
(c) osmotic pressure
(d) freezing point
(c) osmotic pressure
(iii) In calculating osmotic pressure the concentration of solute is expressed in
(a) molarity
(b) molality
(c) mole fraction
(d) mass percent
(a) molarity
(iv) Ebullioscopic constant is the boiling point elevation when the concentration of solution is
(a) 1m
(b) 1M
(c) 1 mass%
(d) 1 mole fraction of solute.
(a) 1m
(v) Cryoscopic constant depends on
(a) nature of solvent
(b) nature of solute
(c) nature of solution
(d) number of solvent molecules
(a) nature of solvent
(vi) Identify the correct statement
(a) vapour pressure of solution is higher than that of pure solvent.
(b) boiling point of solvent is lower than that of solution
(c) osmotic pressure of solution is lower than that of solvent
(d) osmosis is a colligative property.
(b) boiling point of solvent is lower than that of solution
(vii) A living cell contains a solution which is isotonic with 0.3 M sugar solution. What osmotic pressure develops when the cell is placed in 0.1 M KCl solution at body temperature?
(a) 5.08 atm
(b) 2.54 atm
(c) 4.92 atm
(d) 2.46 atm
(c) 4.92 atm
(viii) The osmotic pressure of blood is 7.65 atm at 310 K. An aqueous solution of glucose isotonic with blood has the percentage (by volume)
(a) 5.41 %
(b) 3.54 %
(c) 4.53 %
(d) 53.4 %
(a) 5.41 %
(ix) Vapour pressure of a solution is
(a) directly proportional to the mole fraction of the solute
(b) inversely proportional to the mole fraction of the solute
(c) inversely proportional to the mole fraction of the solvent
(d) directly proportional to the mole fraction of the solvent
(d) directly proportional to the mole fraction of the solvent
(x) Pressure cooker reduces cooking time for food because
(a) boiling point of water involved in cooking is increased
(b) heat is more evenly distributed in the cooking space
(c) the higher pressure inside the cooker crushes the food material
(d) cooking involves chemical changes helped by a rise in temperature.
(a) boiling point of water involved in cooking is increased
(xi) Henry’s law constant for a gas CH3Br is 0.159 moldm-3 atm at 250 0C. What is the solubility of CH3Br in water at 25 0C and a partial pressure of 0.164 atm?
(a) 0.0159 mol L-1
(b) 0.164 mol L-1
(c) 0.026 M
(d) 0.042 M
(c) 0.026 M
(xii) Which of the following statement is NOT correct for 0.1 M urea solution and 0.05 M sucrose solution?
(a) osmotic pressure exhibited by urea solution is higher than that exhibited by sucrose solution
(b) urea solution is hypertonic to sucrose solution
(c) they are isotonic solutions
(d) sucrose solution is hypotonic to urea solution
(c) they are isotonic solutions
Question 2.
Answer the following in one or two sentences
(i) What is osmotic pressure?
Osmotic pressure : The osmotic pressure is defined as the excess mechanical pressure required to be applied to a solution separated by a semipermeable membrane from pure solvent or a dilute solution to prevent the osmosis or free passage of the solvent molecules at a given temperature. The osmotic pressure is a colligative property.
(ii) A solution concentration is expressed in molarity and not in molality while considering osmotic pressure. Why?
While calculating osmotic pressure by equation, p = CRT, the concentration is expressed in molarity but not in molality. This is because the measurements of osmotic pressure are made at a certain constant temperature. Molality depends upon temperature but molality is independent of temperature. Hence in osmotic pressure measurements, concentration is expressed in molarity.
(iii) Write the equation relating boiling point elevation to the concentration of solution.
The boiling point elevation is directly proportional to the molality of the solution. Thus, ΔTb ∝ m or ΔTb = Kbm where m is the molality of solution. The proportionality constant Kb is called boiling point elevation constant or molal elevation constant or ebullioscopic constant. If m = 1, ΔTb = Kb Thus, ebullioscopic constant is the boiling point elevation produced by 1 molal solution.
(iv) A 0.1 m solution of K2SO4 in water has freezing point of -4.3 0C. What is the value of van’t Hoff factor if Kf for water is 1.86 K kg mol-1?
Given : m = 0.1 m, ΔTf = 0 - (- 0.43) = 0.43 0C, Kf = 1.86 K kg mol-1, i=? ΔTf = i x Kf x m ∴ i = \(\frac{ΔT_f}{k_f×m}=\frac{0.43}{1.86×0.1}\)= 2.312 Answer is van’t Hoff factor = i = 2.312.
(v) What is van’t Hoff factor?
Van’t Hoff factor : To obtain the colligative properties of electrolyte solutions by using relations for nonelectrolytes, van’t Hoff suggested a factor i. It is defined as a ratio of the observed colligative property of the solution to the theoretically calculated colligative property of the solution without considering molecular change. The van’t Hoff factor can be represented as, This colligative property may be the lowering of vapour pressure of a solution, the osmotic pressure, the elevation in the boiling point or the depression in the freezing point of the solution. Hence, \(i=\frac{\text{Observed lowering of vapour pressure}}{\text{Theoretical lowering of vapour pressure}}\) = ΔP(ob)/ΔP(th) \(i=\frac{\text{Observed elevation in boiling point}}{\text{Theoretical elevation in boiling point}}\) = ΔTb(ob)/ΔTb(th) \(i=\frac{\text{Observed depression in freezing point}}{\text{Theoretical depression in freezing point}}\) = ΔTf(ob)/ΔTf(th) \(i=\frac{\text{Observed osmotic pressure}}{\text{Theoretical osmotic pressure}}\) = π(ob)/ π(th) From the value of the van’t Hoff factor, the degree of dissociation of electrolytes, degree of association of nonelectrolytes can be obtained. van’t Hoff factor gives the important information about the solute molecules in the solution and chemical bonding in them.
(vi) How is van’t Hoff factor related to degree of ionization?
Consider 1 dm3 of a solution containing m moles of an electrolyte AxBy. The electrolyte on dissociation gives x number of Ay+ ions and y number of Bx- ions. Let a be the degree of dissociation. At equilibrium, AxBy ⇔ xAy+ + yBx- For 1 mole of electrolyte : 1 − α, xα, yα and For ‘m’ moles of an electrolyte : m(1 − α), mxα, myα are the number of particles. Total number of moles at equilibrium, will be, Total moles = m(1 − α) + mxα + myα = m[(1 − α) + xα + yα] = m[1 + xα + yα − α] = m[1 + α(x + y − 1)] The van’t Hoff factor i will be, \(i=\frac{\text{Observed colligative property}}{\text{Theoretical colligative property}}\) ∴\(i=\frac{m[1 + α(x + y − 1)]}{m}\) i = 1 + α(x + y − 1) If total number of ions from one mole of electrolyte is denoted by n, then (x + y) = n ∴ i = 1 + α(n − 1) ∴α(n − 1) = i − 1 ∴ α = \(\frac{i-1}{n-1}\) …..(1) This is a relation between van’t Hoff factor i and degree of dissociation of an electrolyte.(degree of ionization)
(vii) Which of the following solutions will have higher freezing point depression and why ?
a. 0.1 m NaCl
b. 0.05 m Al2(SO4)3
The number of particles (ions) from electrolytes are, a, 0.1 m NaCl NaCl —> Na+ + Cl- (Total ions = 0.1 + 0.1=0.2m) b. 0.05 m Al2(SO4)3 Al2(SO4)3 --> 2Al3+ + 3SO41- , (Total ions = 2 x 0.05 + 3 x 0.05 =0.1 +0.15 = 0.25 m) Therefore, Al2(SO4)3 solution will have higher freezing point depression. .
(viii) State Raoult’s law for a solution containing a nonvolatile solute
Statement of Raoult’s law : The law states that the vapour pressure of a solvent over the solution of a nonvolatile solute is equal to the vapour pressure of the pure solvent multiplied by mole fraction of the solvent at constant temperature. Explanation : Let P0 and P be the vapour pressures of a pure solvent and a solution respectively. If x1 is the mole fraction of the solvent then Raoult’s law can be represented as, P = x1P0 For a binary solution containing one solute, if x1 and x2 are mole fractions of a solvent and a solute respectively then, x1 + x2 = 1 x1 = 1 - x2 P = x1P0 = (1 - x2)P0 = P0 - x2P0 P0 - P = x2P0 ∴ x2 = \(\frac{P_0-P}{P_0}\) P0 - P = ΔP is the lowering of vapour pressure ∴ x2 = \(\frac{ΔP}{P_0}\) In the equation, is called relative lowering of vapour pressure. Hence Raoult’s law can also be stated as the relative lowering of vapour pressure is equal to mole fraction of the solute.
(ix) What is the effect on the boiling point of water if 1 mole of methyl alcohol is added to 1 dm3 of water? Why?
(x) Which of the four colligative properties is most often used for molecular mass determination? Why?
Question 3.
Answer the following.
(i) How vapour pressure lowering is related to a rise in boiling point of solution?
To understand the elevation of boiling point, let us compare the vapour pressures of solution and those of pure solvent. The vapour pressures of solution and of pure solvent are plotted as a function of temperature as shown in Fig. When a nonvolatile solute is added to a solvent, its vapour pressure decreases, hence the boiling point increases. If T0 and T are the boiling points of a pure solvent and a solution, then the elevation in the boiling point is given by, ΔTb = T − T0 The curve AB, represents the variation in the vapour pressure of a pure solvent with temperature while curve CD represents the variation in the vapour pressure of the solution. This elevation in the boiling point is proportional to the lowering of the vapour pressure, i.e., P0 − P, where P0 and P are the vapour pressures of the pure solvent and the solution. [ΔTb ∝ (P0 − P) or ΔTb ∝ ΔP]

(ii) What are isotonic and hypertonic solutions?
Hypotonic solutions : When two solutions have different osmotic pressures, then the solution having lower osmotic pressure is said to be a hypotonic solution with respect to the other solution. Explanation : Consider two solutions of the substances A and B having osmotic pressures πA and πB. If πB is less than πA, then the solution B is a hypotonic solution with respect to the solution A. Hence, if CA and CB are their concentrations, then, CB < CA. Hence, for equal volumes of the solutions, nB < nA. Hypertonic solutions : When two solutions have different osmotic pressures, then the solution having higher osmotic pressure is said to be a hypertonic solution with respect to the other solution. Explanation : Consider two solutions of substances A and B having osmotic pressures πA and πB. If pB is greater than πA, then the solution B is a hypertonic solution with respect to the solution A. Hence, if CA and CB are their concentrations, then, CB > CA. Hence, for equal volumes of the solutions, nB > nA.
(iii) A solvent and its solution containing a nonvolatile solute are separated by a semipermable membrane. Does the flow of solvent occur in both directions? Comment giving reason.
(iv) The osmotic pressure of CaCl2 and urea solutions of the same concentration at the same temperature are respectively 0.605 atm and 0.245 atm. Calculate van’t Hoff factor for CaCl2
Given : πcacl2 = 0.605 atm, πurea = 0.245 atm For urea solution, van’t Hoff factor, i = 1 πcacl2 = i x (CRT)CaCl2 πurea = (CRT) urea πcacl2/πurea = (i x (CRT)CaCl2)/(CRT) urea ∴ i = πcacl2/πurea = 0.605/0.245 = 2.47 Answer is : van’t Hoff factor = i = 2.47
(v) Explain reverse osmosis.
Reverse osmosis : osmosis is a flow of solvent through a semipermeable membrane into the solution. The direction of osmosis can be reversed by applying a pressure larger than the osmotic pressure. The phenomenon of the passage of solvent like water under high pressure from the concentrated aqueous solution like sea water into pure water through a semipermeable membrane is called reverse osmosis. The osmotic pressure of sea water is about 30 atmospheres. Hence when pressure more than 30 atmospheres is applied on the solution side, regular osmosis stops and reverse osmosis starts. Hence pure Water from sea water enters the other side of pure water. For this purpose a suitable semipermeable membrane is required which can withstand high pressure conditions over a long period. This method is used successfully in Florida since 1981 producing more than 10 million litres of pure water per day.
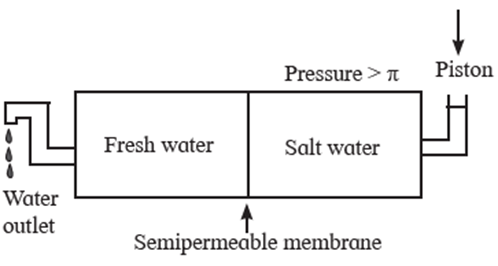
(vi) How molar mass of a solute is determined by osmotic pressure measurement?
Consider V dm3 (litres) of a solution containing W2 mass of a solute of molar mass M2 at a temperature T. Number of moles of solute, n2 = W2/M2 The osmotic pressure p is given by, π = \(\frac{W_2RT}{M_2V}\) ∴ \(M_2=\frac{W_2RT}{πV}\) By measuring osmotic pressure of a solution, the molar mass of a solute can be calculated. Since osmotic pressure can be measured more precisely, it is widely used to measure molar masses of the substances
(vii) Why vapour pressure of a solvent is lowered by dissolving a nonvolatile solute into it?
When a nonvolatile solute is added to a pure solvent, the surface area is covered by the solute molecule decreasing the rate of evaporation, hence its vapour pressure decreases. This decrease in vapour pressure is called lowering of vapour pressure.
(viii) Using Raoult’s law, how will you show that ΔP = P01 x2 ? Where x2 is the mole fraction of solute in the solution and P01 vapour pressure of pure solvent.
If x1 and x2 are the mole fractions of a solvent and a solute respectively, then x1 + x2 = 1 By Raoult’s law, P = x1P0. where P0 is the vapour pressure of a pure solvent and P is the vapour pressure of the solution at given temperature. ∴ P/P0 = x1 1− P/P0 = 1 − x1 = x2 If P0 − P = ΔP then ΔP/ P0 = x2. ∴ΔP = P0x2
(ix) While considering boiling point elevation and freezing point depression a solution concentration is expressed in molality and not in molarity. Why?
Question 4.
Derive the relationship between degree of dissociation of an electrolyte and van’t Hoff factor.
Consider 1 dm3 of a solution containing m moles of an electrolyte AxBy. The electrolyte on dissociation gives x number of Ay+ ions and y number of Bx- ions. Let a be the degree of dissociation. At equilibrium, AxBy ⇔ xAy+ + yBx- For 1 mole of electrolyte : 1 − α, xα, yα and For ‘m’ moles of an electrolyte : m(1 − α), mxα, myα are the number of particles. Total number of moles at equilibrium, will be, Total moles = m(1 − α) + mxα + myα = m[(1 — α) + xα + yα] = m[1 + xα + yα − α] = m[1 + α(x + y − 1)] The van’t Hoff factor i will be, \(i=\frac{\text{Observed colligative property}}{\text{Theoretical colligative property}}\) ∴\(i=\frac{m[1 + α(x + y − 1)]}{m}\) i = 1 + α(x + y − 1) If total number of ions from one mole of electrolyte is denoted by n, then (x + y) = n ∴ i = 1 + α(n − 1) ∴α(n − 1) = i −1 ∴ α = \(\frac{i−1}{n−1}\) …..(1) This is a relation between van’t Hoff factor i and degree of dissociation of an electrolyte.
Question 5.
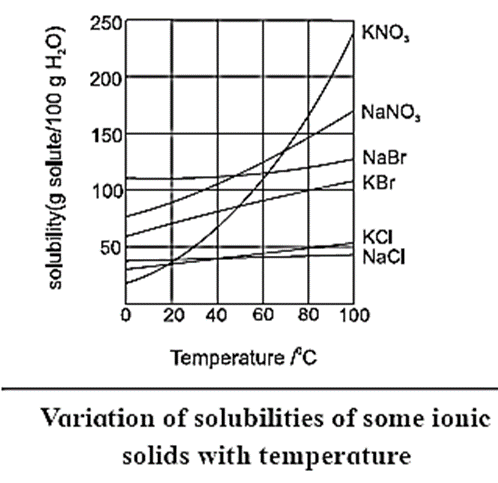
What is effect of temperature on solubility of solids in water? Give examples.
The solubility of a solid solute depends upon temperature. Generally rise in temperature increases the solubility. This is due to expansion of holes or empty spaces in the liquid solvent. Generally 10 °C rise in temperature, increases the solubility of solids two fold. Figure shows the result of experimental determination of solubilities of some ionic solids in water at various temperatures. Following are some experimental observations from Fig This variation in solubility with temperature can be used to separate the salts from the mixture by fractional clystallisation.
Question 6.
Obtain the relationship between freezing point depression of a solution containing nonvolatile nonelctrolyte and its molar mass.
The freezing point depression, ΔTf of a solution is directly proportional to molality (m) of the solution. ΔTf ∝ m ΔTf = Kf m where Kf is a molal depression constant. The molality of a solution is given by, m = \(\frac{\text{ Number of moles of the solute}}{\text{Weight of the solvent in kg}}\) If W1 grams of a solvent contain W2 grams of a solute of the molar mass M2, then the molality m of the solution is given by, m = \(\frac{W_2×1000}{W_1M_2}=\frac{W_2}{W_1M_2}\) mol kg−1 ∴ ΔTf = Kf x \(\frac{W_2×1000}{W_1M_2}\) ∴ M2 = \(\frac{K_fW_2×1000}{ΔT_fW_1M_2}\) or M2 = \(\frac{K_fW_2}{ΔT_fW_1M_2}\) mol kg−1 If the weights and molecular weight are expressed in kg, then, ∴ ΔTf = Kf x \(\frac{W_2}{W_1M_2}\) The unit of Kf is K kg mol-1 . Hence, from the measurement of the depression in the freezing point of the solution, the molar mass of the substance can be determined.
Question 7.
Explain with diagram the boiling point elevation in terms of vapour pressure lowering.
To understand the elevation of boiling point, let us compare the vapour pressures of solution and those of pure solvent. The vapour pressures of solution and of pure solvent are plotted as a function of temperature as shown in Fig. When a nonvolatile solute is added to a solvent, its vapour pressure decreases, hence the boiling point increases. If T0 and T are the boiling points of a pure solvent and a solution, then the elevation in the boiling point is given by, ΔTb = T − T0 The curve AB, represents the variation in the vapour pressure of a pure solvent with temperature while curve CD represents the variation in the vapour pressure of the solution. This elevation in the boiling point is proportional to the lowering of the vapour pressure, i.e., P0 − P, where P0 and P are the vapour pressures of the pure solvent and the solution. [ΔTb ∝ (P0 − P) or ΔTb ∝ ΔP]

Question 8.
Fish generally needs O2 concentration in water at least 3.8 mg/L for survival. What partial pressure of O2 above the water is needed for the survival of fish? Given the solubility of O2 in water at 0 0C and 1 atm partial pressure is 2.2 × 10-3 mol/L
Given : Required concentration of O2 = 3.8 mg/L = Solubility of O2 = 2.2 x 10-3 mol L-1 P = 1 atm Partial pressure of O2 needed = Po2 = ? S = KH x Po2 ∴ KH = S/ Po2 = (2.2 x 10-3)/1 mol L-1 atm-1 = 2.2 x 10-3 mol L-1 atm-1 Po2 = S/KH = = 0.05397 atm Ans. Pressure needed = Po2 = 0.05397 aim.
Question 9.
The vapour pressure of water at 200C is 17 mm Hg. What is the vapour pressure of solution containing 2.8 g urea in 50 g of water?
Given : Vapour pressure of pure solvent (water) = P0 = 17 mm Hg Weight of solvent = W1 = 50 g Weight of solute (urea) = W2 = 2.8 g Molecular weight of a solvent = M1 = 18 Molecular weight of a solute (urea) = M2 = 60 g mol-1 \(\frac{P_0-P}{P_0} =\frac{W_2/M_2}{W_1/M_1}\) \(\frac{17-P}{17} =\frac{2.8×18}{50×60}=0.0168\) 17-P = 17 x 0.0168 ∴ P = 17 − 0.2856 = 16.7144 mm Hg Vapour pressure of solution = 16.7144 mm Hg
Question 10.
A 5% aqueous solution (by mass) of cane sugar (molar mass 342 g/mol) has freezing point of 271K. Calculate the freezing point of 5% aqueous glucose solution.
Given : W2 = 5 g cane sugar, W1 = 100 − 5 = 95 g, M2 = 342 g mol-1, Tf1 = 271 K; ΔTf1 =273 − 271 = 21K, Tf = ? W2’ = 5 g glucose, W1’ = 100 − 5 = 95 g, M2’ = 180 g mol-1, ΔTf2 = ? ΔTf = Kf x \(\frac{W_2×1000}{W_1M_2}\) Kf = \(\frac{ΔT_fW_1M_2}{W_2×1000}\) ΔTf2 = Kf x \(\frac{W'_2×1000}{W'_1M'_2}=\frac{13×5×1000}{95×180}=3.801 K\) Freezing point of solution = Tf = = 273 − 3.801 = 269.199 K Freezing point of solution = 269.2 K.
Question 11.
A solution of citric acid C6H8O7 in 50 g of acetic acid has a boiling point elevation of 1.76 K. If Kb for acetic acid is 3.07 K kg mol-1, what is the molality of solution?
Given : W1 = 50 g acetic acid ΔTb = 1.76 K Kb = 3.07 K kg mol-1 m = ? ΔTb = Kb x m m = ΔTb/ Kb = 1.76/3.07 = 0.5733 m Molality of solution = 0.5733 m
Question 12.
An aqueous solution of a certain organic compound has a density of 1.063 gmL-1, an osmotic pressure of 12.16 atm at 250C and a freezing point of −1.03 0C. What is the molar mass of the compound?
Given: Density of a solution d = 1.063 g mL-1 = 1.063, Osmotic pressure of solution π = 12.16 atm, Temperature T = 25 °C = 298.15 K, Freezing point of solution = Tf = –1.03 0C, ∴ ΔTf = 0 – (−1.03) =1.03 0C R = 0.08206 dm3 atm K-1 mol-1, Kf of water = 1.86 K kg mol-1 ΔTf = Kf m ∴ m = ΔTf/ Kf = 1.03/ 1.86 = 0.554 mol kg-1 = 0.554 M π = CRT 12.16 = C x 0.08206 x 298.15 ∴ C = 0.497 mol dm–3 = 0.497 M Mass of solvent W1 = C/m = (0.497/0.554) x 1 dm3 = 0.897 kg = 897 g Mass of Solution = 1.063 x g mL–1 x 1000 mL = 1063g = 1.063 kg =1063 g ∴ Mass of Solute W2 = 1.063 – 0.897 = 0.166 kg =166 g m = \(\frac{W_2×1000}{W_1M_2}\) ∴ M2 = \(\frac{W_2×1000}{W_1m}= \frac{166g×1000g\,Kg^{-1}}{897g×0.554mol\,kg^{-1}}\) = 334 g mol-1 Molar mass of the compound = 334 g mol-1
Question 13.
A mixture of benzene and toluene contains 30% by mass of toluene. At 300C, vapour pressure of pure toluene is 36.7 mm Hg and that of pure benzene is 118.2 mm Hg. Assuming that the two liquids form ideal solutions, calculate the total pressure and partial pressure of each constituent above the solution at 300C.
Given : 30% by mass of toluene (T) and 70% by mass of benzene (B). WT = 30 g, WB = 70 g, P0T = 36.7 mm Hg, P0B = 118.2 mmHg MT = 92 g mol-1, MB = 78 g mol-1 PT = ?, PB = ?, Psoln = ? nT = WT/ MT = 30/92 = 0.3260 mol nB = WB/ MB = 70/78 = 0.8974 mol Total number of moles = nTotal = nT + nB = 0.326 + 0.8974 = 1.2234 mol Mole fractions : xT = nT/ nTotal = 0.326/1.2234 = 0.2665 xB = 1-0.2665 = 0.7335 Psoln = xT x P0T + xB x P0B = 0.2665 x 36.7 + 0.7335 x 118.2 = 9.780 + 86.7 = 96.48 mm Hg Partial pressures : PT = xT x Psoln = 0.2665 x 96.48 = 25.71 mm Hg PB = xB X Psoln = 0.7335 x 96.48 = 70.77 mm Hg Answer is : Total pressure = Psoln = 96.48 mm Hg Partial pressures Toluene : PT = 25.71 mm Hg Partial pressures Benzene : PB = 70.77 mm Hg
Question 14.
At 25 0C a 0.1 molal solution of CH3COOH is 1.35 % dissociated in an aqueous solution. Calculate freezing point and osmotic pressure of the solution assuming molality and molarity to be identical.
Given: T= 273 + 25 = 298 K, C= 0.1 m ≅ 0.1 M, Kf = 1.86 K kg mol-1, Per cent dissociation = 1.35, Freezing point = tf = ?, p = ? α = 1.35/100 = 0.013 CH3COOH ↔ CH3COO + H+ 1 − α α α i = 1 − α + α + α = 1 + α = 1 + 0.0135 = 1.0135 (i) ΔTf = i x Kf x m = 1.0135 x 1.86 x 0.1 = 0.1885 °C Freezing point of solution = 0 − 0.1885 = − 0.1885 °C (ii) π = iCRT = 1.035 x 0.1 x 0.08206 x 298 = 2.53 atm Answer is : (i) Freezing point of solution = − 0.1885 °C (ii) Osmotic pressure = π = 2.53 atm.
Question 15.
A 0.15 m aqueous solution of KCl freezes at −0.510 0C. Calculate i and osmotic pressure at 0 0C. Assume volume of solution equal to that of water
Given : c = 0.15 m KCl ≅ 0.15 M KCl, ΔTf = 0 − Tf = 0 − (− 0.510) = 0.510 °C T = 273 K, Kf = 1.86 K kg mol-1 i = ?; π = ? ΔTf = i x Kf x m ∴ i = \(\frac{ΔT_f}{K_fm}\) π = iCRT = 1.828 x 0.15 x 0.08206 x 273 = 6.143 atm Answer is i = 1.828, Osmotic pressure = p = 6.143 atm
Henry’s law : S = KHP Raoult’s law : Psoln = x1P0 Mole fractions, x1 + x2 = 1 Psoln = P01 x1 + P02 x2 Psoln = (P02 - P01)x2 + P01 Mole fractions of components in vapour phase : and For ideal solutions : ΔVmix = 0; Δmix H = 0 Relative lowering of vapour pressure = \(\frac{p^0-P}{P^0}\), \(x_2=\frac{p^0-P}{P^0}\) \(\frac{p^0-P}{P^0}=\frac{W_2×M_1}{W_1×M_2}\) ΔTb = Kb x m and ΔTf = Kf x m ΔTb = Kb x \(\frac{W_2×1000}{W_1×M_2}\) ΔTf = Kf x \(\frac{W_2×1000}{W_1×M_2}\) π = cRT and π = \(\frac{WRT}{MV}\) \(i=\frac{\text{Colligative property of electrolyte solution}}{\text{Colligative property of nonelectrolyte solution of the same concentration}}\) van’t Hoff factor (i) = \(\frac{ΔT_{b(ob)}}{ΔT_{b(th)}}\) = \(\frac{ΔP_{(ob)}}{ΔP_{(th)}}\) = \(\frac{ΔT_{f(ob)}}{ΔT_{f(th)}}\) = \(\frac{π_{(ob)}}{π_{(th)}}\) = \(\frac{M_{(ob)}}{M_{(th)}}\) Colligative properties considering van’t Hoff factor :
Important Formulae :
PDF : Class-12-Chemistry-Chapter-2-Solutions-Text Book
PDF : Class-12-Chemistry-Chapter-2-Solutions- Notes
PDF : Class-12-Chemistry-Chapter-2-Solutions- Solution
All 16 Chapters Notes -Class-12-Chemistry (16-PDF)
All 16 Chapters Solutions -Class-12-Chemistry (16-PDF)
All 16 Chapters Notes+Solutions -Class-12-Chemistry (32-PDF)
Main Page : – Maharashtra Board Class 12th-Chemistry – All chapters notes, solutions, videos, test, pdf.
Previous Chapter : Chapter-1-Solid State – Online Solution
Next Chapter : Chapter-3-Ionic Equilibria – Online Solution
We reply to valid query.