Triangles
Class-9-Mathematics-2-Chapter-3-Maharashtra Board
Notes
|
Topics to be learn :
|
Know This :
(i) Consider Δ PQR. Extend side QR and mark point T on it such that Q−R−T.
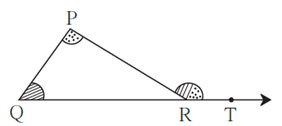
- ∠PRT forms a linear pair with one of the interior angles, i.e. ∠
- ∠PRT is an exterior angle of Δ PQR with respect to ∠
- The other two angles, ∠QPR and ∠PQR are remote interior angles.
(ii) A triangle has six exterior angles.
Theorem of remote interior angles of a triangle :
Theorem: The measure of an exterior angle of a triangle is equal to the sum of its remote interior angles.
Given : ∠PRS is an exterior angle of Δ PQR.

Prove : ∠PRS = ∠PQR + ∠QPR
Proof : The sum of all angles of a triangle is 180°.
∴ ∠PQR + ∠QPR + ∠PRQ = 180°.......(I)
∠PRQ + ∠PRS = 180°.....angles in linear pair......(II)
∴ from (I) and (II)
∠PQR + ∠QPR + ∠PRQ = ∠PRQ + ∠PRS
∴ ∠PQR + ∠QPR = ∠PRS .......eliminating ∠PRQ from both sides
∴ the measure of an exterior angle of a triangle is equal to the sum of its remote
interior angles.
Alternative proof :
Construction : Through R, draw a line parallel to seg PQ. Mark a point T on it as shown.
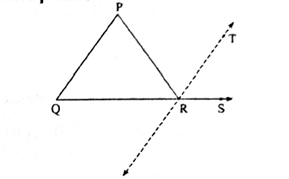
Proof :
seg PQ || line RT ... (Construction)
Line PR is the transversal,
∴ ∠PRT = ∠QPR ... (Alternate angles) ... (I)
Also, line QR is the transversal,
∴ ∠TRS = ∠PQR ... (Corresponding angles) ... (II)
Adding (I) and (II), we get,
∠PRT + ∠TRS = ∠QPR + ∠PQR
∴ ∠PRS = ∠PQR + ∠QPR ... (Angles addition postulate)
Property of an exterior angle of triangle :

If ∠PRS is an exterior angle of D PQR then ∠PRS > ∠QPR , ∠PRS > ∠PQR
∴ an exterior angle of a triangle is greater than its remote interior angle.
Examples :
Q.1. Observe below figure and find the measures of ∠PRS and ∠RTS.
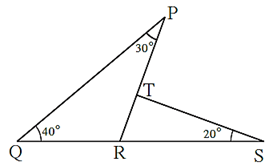
Solution : ∠PRS is an exterior angle of Δ PQR.
So from the theorem of remote interior angles,
∠PRS = ∠PQR + ∠QPR = 40° + 30° = 70°
In ΔRTS
∠TRS + ∠RTS + ∠TSR = [180°] ........ sum of all angles of a triangle
∴ [70°] + ∠RTS + [20°] = 180°
∴ ∠RTS + 90° = 180°
∴ ∠RTS = [90°]
Q.2. In figure bisectors of ∠B and ∠C of Δ ABC intersect at point P.
Prove that ∠BPC = 90 + \(\frac{1}{2}\)∠BAC.
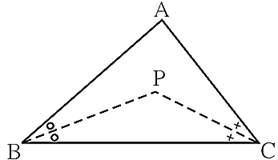
Proof : In Δ ABC,
∠BAC + ∠ABC + ∠ACB = [180°] ...... sum of measures of angles of a triangle
∴ \(\frac{1}{2}\)∠BAC + \(\frac{1}{2}\)∠ABC + \(\frac{1}{2}\)∠ACB = \(\frac{1}{2}\) × [180°] ....multiplying each term by
∴ \(\frac{1}{2}\) ∠BAC + ∠PBC + ∠PCB = 90°
∴ ∠PBC + ∠PCB = 90° − \(\frac{1}{2}\)∠BAC ......(I)
In Δ BPC
∠BPC + ∠PBC + ∠PCB = 180° ......sum of measures of angles of a triangle
∴ ∠BPC + [90° + \(\frac{1}{2}\) ∠BAC ] = 180° ......from (I)
∴ ∠BPC = 180° − (90° − \(\frac{1}{2}\)∠BAC)
= 180° − 90° + \(\frac{1}{2}\)∠BAC
= 90° + \(\frac{1}{2}\)∠BAC
Congruence of triangles :
- Consider ΔABC and ΔPQR of any shape and size.
- There are six different ways of putting one triangle over the other among the two sets of vertices of triangles.
They are
(i) ABC ⇔ PQR (ii) ABC ⇔ QPR
(iii) ABC ⇔ QRP (iv) ABC ⇔ RQP
(v) ABC ⇔ RPQ (vi) ABC ⇔ PRQ
- Out of six correspondences, between the vertices of the triangles, if there exists at least one in which the triangles cover each other exactly, then we say the triangles are congruent with respect to that correspondence.
- Suppose by correspondence ABC ⇔ QPR the triangles cover each other exactly, then we write Δ ABC ≅ Δ
- If two triangles are congruent, then corresponding angles and corresponding sides of those two triangles are congruent.
- Corresponding sides of congruent triangles is written as c.s.c.t and corresponding angles of congruent triangles is written as c.a.c.t.
Test of Congruence :
(1) A-S-A test : In a correspondence, if two angles of Δ ABC are equal to two angles of Δ PQR and the sides included by the respective pairs of angles are also equal, then the two triangles are congruent.
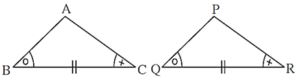
This property is called as angle-side-angle test, which in short we write A-S-A test.
(2) S-A-S test : In a correspondence, if two sides of Δ ABC are equal to two sides of ΔPQR and the angles included by the respective pairs of sides are also equal, then the two triangles are congruent.

This property is called as side-angle-side test, which in short we write S-A-S test.
(3) S-S-S test : In a correspondence, if three sides of Δ ABC are equal to three sides of ΔPQR , then the two triangles are congruent.
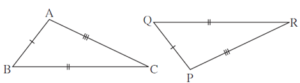
This property is called as side-side-side test, which in short we write S-S-S test.
(4) Hypotenuse side test : If in Δ ABC and Δ PQR, ∠B and ∠Q are right angles, hypotenuses are equal and AB = PQ, then the two triangles are congruent.

This property is called the hypotenuse side test.
(5) Extension of A-S-A test :
If two angles of one triangle are congruent to the two angles of another triangle, then the remaining angles of these triangles are congruent. ... (The sum of the measures of the angles of a triangle is 180°.)
∴ A-S-A test can be considered as A-A-S test or S-A-A test.

Thus, if in Δ ABC and Δ PQR, AB = PQ,
∠ABC ≅ ∠ PQR and ACB ≅ ∠PRQ, then the two triangles are congruent.
This property is called the 'S-A-A' or 'A-A-S' test.
Note : The congruent sides of two triangles must be opposite to the congruent angles.
Isosceles triangle :
Theorem : If two sides of a triangle are congruent then the angles opposite to them are congruent.
Given : In Δ ABC, side AB ≅ side AC
To prove : ∠ABC ≅ ∠ACB
Construction : Draw the bisector of ∠BAC which intersects side BC at point D.

Proof : In Δ ABD and Δ ACD
seg AB ≅ seg AC ....... given
∠BAD ≅ ∠CAD ........construction
seg AD ≅ seg AD ....... common side
∴ Δ ABD ≅ Δ ACD ...... [SAS test of congruence]
∴ ∠ABD ≅ [∠ACD] ....... (c.a.c.t.)
∴ ∠ABC ≅ ∠ACB ∵ B-D-C
Corollary : If all sides of a triangle are congruent then its all angles are congruent.
Given : Δ ABC is equilateral.

To prove :
Δ ABC is equiangular.
Proof : In ABC,
seg AB ≅ seg AC
∴ ∠ABC ≅ ∠ACB ... (Isosceles triangle theorem) ... (1)
In Δ ABC, Seg AC ≅ seg BC ... (Sides of an equilateral triangle)
∴ ∠ABC ≅ ∠BAC ... (Isosceles triangle theorem) ... (2)
∴ ∠ABC ≅ ∠ACB ≅ ∠BAC ... [From (1) and (2)]
∴ Δ ABC is equiangular.
Converse of isosceles triangle theorem
Theorem : If two angles of a triangle are congruent then the sides opposite to them are congruent.
Given : In Δ PQR, ∠PQR ≅ ∠PRQ
To prove : Side PQ ≅ side PR
Construction : Draw the bisector of ∠P intersecting side QR at point M
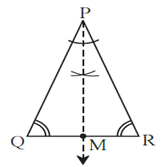
Proof : In Δ PQM and Δ PRM
∠PQM ≅ [∠PRM] ........ given
∠QPM ≅ ∠RPM ........ [Construction]
seg PM ≅ [seg PM] ...... common side
∴ Δ PQM ≅ Δ PRM ...... [ASA] test
∴ seg PQ ≅ seg PR....... c.s.c.t.
Corollary : If three angles of a triangle are congruent then its three sides also are congruent.
Given : In Δ ABC, ∠A ≅ ∠B ≅ ∠C
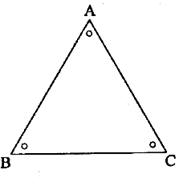
To prove : side AB ≅ side BC ≅ side AC.
Proof : In Δ ABC, ∠B ≅ ∠C …..(Given)
∴ side AB ≅ side AC …. (Converse of isosceles triangle theorem) ... (1)
In Δ ABC, ∠A ≅ ∠B .….(Given)
∴ side BC ≅ side AC ... (Converse of isosceles triangle theorem) ... (2)
∴ side AB ≅ side BC ≅ side AC ... (From 1 and 2)
Property of 30° − 60° − 90° triangle
Theorem : If the acute angles of a right angled triangle have measures 30° and 60°, then the length of the side opposite to 30° angle is half the length of the hypotenuse.
Given : In Δ ABC ∠B = 90°, ∠C = 30°, ∠A = 60°
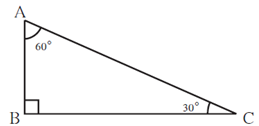
To prove : AB = \(\frac{1}{2}\)AC
Construction : Take a point D on the extended seg AB such that AB = BD. Draw seg DC.
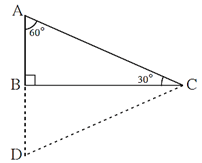
Proof : Δ ABC and Δ DBC
seg AB ≅ seg DB ...........[Construction]
∠ABC ≅ ∠DBC ........ [Each measures 90°]
seg BC ≅ seg BC ............ [Common side]
∴ Δ ABC ≅ Δ DBC ..... [SAS test of congruence]
∴ ∠BAC ≅ ∠BDC ........ (c.a.c.t.)
In Δ ABC, ∠BAC = 60° ∴ ∠BDC = 60°
∠DAC = ∠ADC = ∠ACD = 60° ... ∵ sum of angles of Δ ADC is 180°
∴ Δ ADC is an equilateral triangle.
∴ AC = AD = DC ........ corollary of converse of isosceles triangle theorem
But AB = AD ........ construction
∴ AB = \(\frac{1}{2}\)AC ........ ∵ AD = AC
Theorem : If the acute angles of a right angled triangle have measures 30°and 60° then the length of the side opposite to 60° angle is \(\frac{\sqrt{3}}{2}\) × hypotenuse.
Proof : In the above theorem we have proved AB = \(\frac{1}{2}\)AC

AB2 + BC2 = [AC2] .......... Pythagoras theorem
\(\frac{1}{4}\)AC2 + BC2 = [AC2]
∴ BC2 = AC2 - \(\frac{1}{2}\)AC2
∴ BC2 = \(\frac{4AC^2-AC^2}{4}\)
∴ BC2 = [ \(\frac{3}{4}\) AC2]
∴ BC = \(\frac{\sqrt{3}}{2}\)AC
Theorem : If measures of angles of a triangle are 45°, 45°, 90° then the length of each side containing the right angle is \(\frac{1}{\sqrt{2}}\) × hypotenuse.
Proof :
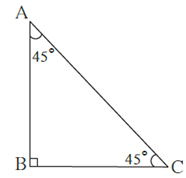
In Δ ABC, ∠B = 90° and ∠A = ∠C = 45°
∴ BC = AB
By Pythagoras theorem
AB2 + BC2 = [AC2]
AB2 + [AB2] = AC2 ... ∵ (BC = AB)
∴ 2AB2 = [AC2]
∴ AB2 = [\(\frac{1}{2}\)AC2]
∴ AB = \(\frac{1}{\sqrt{2}}\) AC
This property is called 45°- 45°- 90° theorem.
Remember :
- If the acute angles of a right angled triangle are 30°, 60°then the length of side opposite to 30° angle is half of hypotenuse and the length of side opposite to 60° angle is \(\frac{\sqrt{3}}{2}\) × hypotenuse This property is called 30°-60°-90°theorem.
- If acute angles of a right angled triangle are 45°, 45° then the length of each side containing the right angle is \(\frac{hypotenuse}{\sqrt{2}}\) .
This property is called 45°-45°-90° theorem.
Median of a triangle :
(1) The segment joining a vertex and the midpoint of the side opposite to it is called median of the triangle.
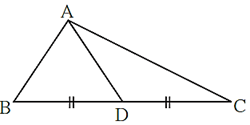
In the above figure, D is the midpoint of side BC. Therefore, seg AD is the median.
(2) A triangle has three medians.
(3) The medians of the triangle are concurrent and the point of concurrence of medians is called as centroid.

In the figure, the medians AD, CF and BE intersect at G (centroid).
Centroid Property :
The point of concurrence of medians of a triangle (centroid) divides each median in the ratio 2: 1. i.e. AG : GD = 2:1, BG : GE = 2:1, CG : GF = 2:1.
Property of median drawn on the hypotenuse of right triangle :
Theorem : In a right angled triangle, the length of the median of the hypotenuse is half the length of the hypotenuse.
Given : In Δ ABC, ∠B = 90°, seg BD is the median.
To prove : BD = \(\frac{1}{2}\)AC
Construction : Take point E on the ray BD such that B-D–E and l(BD) = l(DE). Draw seg EC.
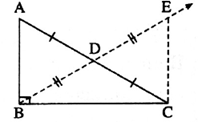
Proof : In Δ ADB and Δ CDE,
seg AD ≅ seg CD ... (D is the midpoint of seg AC)
∠ADB ≅ ∠CDE ... (Vertically opposite angles)
seg BD ≅ seg ED ... (Construction)
∴ Δ ADB ≅ Δ CDE ... (SAS test of congruence)
∴ seg AB ≅ seg CE ... (c.s.c.t.) ... (1)
∴ ∠BAD ≅ ∠ECD ... (c.a.c.t.)
∠BAC ≅ ∠ECA ... (A-D-C)
∴ line AB || line CE ... (Alternate angles test for parallel lines)
and line BC is the transversal,
∴ ∠ABC + ∠ECB = 180° ... (Interior angles)
∴ 90° + ∠ECB = 180° ... (Each measures 90°)
∴ ∠ECB = 180° - 90° ... (Common side)
∴ ∠ECB = 90° ... (SAS test of congruence)
In Δ ABC and Δ ECB, ... (c.s.c.t.)
seg AB ≅ seg EC ... [From (1)]
∠ABC ≅ ∠ECB .. (2)
seg BC ≅ seg CB ... (Construction)
∴ Δ ABC ≅ Δ ECB ... [From (2)]
∴ seg AC ≅ seg EB
∴ AC = EB
BD = \(\frac{1}{2}\)EB
∴ BD = \(\frac{1}{2}\)AC
Remember : In a right angled triangle, the length of the median on its hypotenuse is half the length of the hypotenuse.
Perpendicular bisector theorem :
Part I : Every point on the perpendicular bisector of a segment is equidistant from the end points of the segment.
Given : line l is the perpendicular bisector of seg AB at point M. Point P is any point on l,
To prove: PA = PB
Construction : Draw seg AP and seg BP.
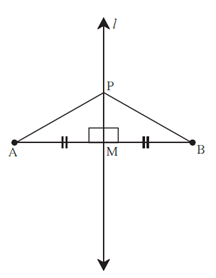
Proof : In Δ PMA and Δ PMB
seg PM ≅ seg PM ....... common side
∠PMA ≅ ∠PMB .........each is a right angle
seg AM ≅ seg BM .......given
∴ Δ PMA ≅ Δ PMB ...... S-A-S test
∴ seg PA ≅ seg PB .......c.s.c.t.
∴ l(PA) = l(PB)
Hence every point on the perpendicular bisector of a segment is equidistant from
the end points of the segment.
Part II : Any point equidistant from the end points of a segment lies on the perpendicular bisector of the segment.
Given : Point P is any point equidistant from the end points of seg AB. That is, PA = PB.
To prove: Point P is on the perpendicular bisector of seg AB.
Construction : Take mid-point M of seg AB and draw line PM.
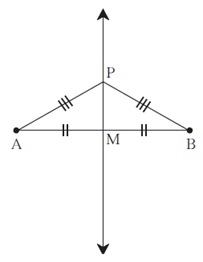
Proof : In Δ PAM and Δ PBM
seg PA ≅ seg PB ......... [(Given)]
seg AM ≅ seg BM .......[ (M is the midpoint of seg AB)]
seg PM ≅ [seg PM] ....... common side
∴ Δ PAM ≅ Δ PBM ...... [SSS] test.
∴ ∠PMA ≅ ∠PMB.......c.a.c.t.
But ∠PMA + [∠PMB] = 180°
∠PMA + ∠PMA = 180° ........ (∵ ∠PMB = ∠PMA)
2∠PMA = [180°]
∴ ∠PMA = 90°
∴ seg PM ⊥ seg AB ......(1)
But Point M is the midpoint of seg AB. ......(construction) .... (2)
∴ line PM is the perpendicular bisector of seg AB.
So point P is on the perpendicular bisector of seg AB
Angle bisector theorem :
Part I : Every point on the bisector of an angle is equidistant from the sides of the angle.
Given : Ray QS is the bisector of ∠PQR . Point A is any point on ray QS
seg AB ⊥ ray QP seg AC ⊥ ray QR

To prove : seg AB ≅ seg AC
Proof : In Δ ABQ and Δ ACQ,
seg QA ≅ seg QA ... (Common side)
∠ABQ ≅ ∠ACQ ... (Each measures 90°)
∠AQB ≅ ∠AQC ... (Ray QA bisects ZPQR)
∴ Δ ABQ ≅ Δ ACQ ... (SAA test of congruence)
∴ seg AB ≅ seg AC ... (c.s.c.t.)
Part II : Any point equidistant from sides of an angle is on the bisector of the angle.
Given : A is a point in the interior of ∠PQR.
seg AC ⊥ ray QR seg AB ⊥ ray QP and AB = AC

To prove : Ray QA is the bisector of ∠PQR.
That is ∠BQA = ∠CQA
Proof : In Δ ABQ and Δ ACQ,
∴ ∠ABQ = ∠ACQ = 90° ... (Given)
Hypotenuse AQ ≅ Hypotenuse AQ ... (Common side)
Side AB ≅ side AC ... (Given)
∴ Δ ABQ ≅ Δ ACQ ... (By hypotenuse side test of congruence)
∴ ∠BQA ≅ ∠CQA ... (c.a.c.t.)
∴ ray QA is the bisector of ∠PQR.
Properties of inequalities of sides and angles of a triangle
Theorem : If two sides of a triangle are unequal, then the angle opposite to the greater side is greater than angle opposite to the smaller side.
Given : In Δ XYZ, side XZ > side XY
To prove : ∠XYZ > ∠XZY
Construction : Take point P on side XZ such that XY = XP, Draw seg YP.

Proof : In Δ XYP
XY = XP .........construction
∴ ∠XYP = ∠XPY.....isosceles triangle theorem .....(I)
∠XPY is an exterior angle of D YPZ.
∴ ∠XPY > ∠PZY .........exterior angle theorem
∴ ∠XYP > ∠PZY ..........from (I)
∴ ∠XYP + ∠PYZ > ∠PZY ........If a > b and c > 0 then a + c > b
∴ ∠XYZ > ∠PZY, that is ∠XYZ > ∠XZY
Theorem : If two angles of a triangle are unequal then the side opposite to the greater angle is greater than the side opposite to smaller angle.
Given : In Δ ABC, ∠B > ∠C

To prove : AC > AB
Proof : There are only three possibilities regarding the lengths of side AB and
side AC of Δ ABC
(i) AC < AB (ii) [AC = AB] (iii) [AC > AB]
(i) Let us assume that AC < AB.
If two sides of a triangle are unequal then the angle opposite to greater side is [greater].
∴ ∠C > [∠B]
But ∠C < ∠B ......... (given)
This creates a contradiction.
∴ [AC] < [AB] is wrong.
(ii) If AC = AB
then ∠B = ∠C
But [∠B] > [∠C] ...... (given)
This also creates a contradiction.
∴ [AC] = [AB] is wrong
∴ AC > AB is the only remaining possibility.
∴ AC > AB
Theorem : The sum of any two sides of a triangle is greater than the third side.
Given : Δ ABC is any triangle.
To prove : AB + AC > BC
AB + BC > AC
AC + BC > AB
Construction : Take a point D on ray BA such that AD = AC.

Proof : In Δ ACD, AC = AD ..... construction
∴ ∠ACD = ∠ADC . ..... c.a.c.t.
∴ ∠ACD + ∠ACB > ∠ADC
∴ ∠BCD > ∠ADC
∴ side BD > side BC .........the side opposite to greater angle is greater
∴ BA + AD > BC .............. ∵ BD = BA + AD
BA + AC > BC ........ ∵ AD = AC
Similarly we can prove that AB + BC > AC and BC + AC > AB.
Similar triangles and Similarity of triangles :
(1) In Δ ABC and Δ PQR,
If (i) ∠A = ∠P, ∠B = ∠Q, ∠C = ∠R and
(ii) , then Δ ABC and Δ PQR are called similar triangle.
‘Δ ABC and Δ PQR are similar' is written as 'Δ ABC ~ Δ PQR'.
(2) If corresponding angles of two triangles are equal, then the two triangles are similar.
(3) If two triangles are similar, then their corresponding sides are in proportion and corresponding angles are congruent.
PDF-Notes,Solution,Text Book
PDF : Class 9th-Mathematics-2-Chapter-3-Triangles-Notes
PDF : Class 9th-Mathematics-2-Chapter-3-Triangles-Solution
Main Page : – Maharashtra Board Class 9th-Mathematics – All chapters notes, solutions, videos, test, pdf.
Previous Chapter : Chapter-2-Parallel Lines – Online Notes
Next Chapter : Chapter-4-Constructions of Triangles – Online Notes
We reply to valid query.