Units and Measurements
Class-11-Science-Physics-Chapter-1-Maharashtra Board
Notes-Part-1
|
Topics to be Learn : Part-1
|
Physics is a quantitative science, in which various physical quantities are measured during experiments.
Physical quantity : A physical quantity is a property of a material or system that can be quantified by measurement. Physical property of material or system means physical property of matter, radiation or interaction among them, which can be subjected equally to all three elements of scientific study, i.e.
- Detailed analysis
- Precise measurement
- Mathematical treatment.
Unit of physical quantity : The standard measure of any quantity is called the unit of that physical quantity. It is clearly and exactly stated and multiples of it are used to express the magnitudes of that quantity.
Measurement of a physical quantity : The process of measurement consists of finding how many times a unit measure of that physical quantity is present in the quantity being measured.
In the measurement of a physical quantity, the measured value is expressed as nu, where u is the unit and n is a numeric which gives the multiple of that unit measure. Example : The statement ‘The length of wall is 5.0 metre’ means that the wall is 5.0 times as long as the standard unit of length, the metre.
The essential characteristics of a standard unit :
- It must not change with time, place and environmental conditions.
- It must be easily end accurately reproducible.
- It must be comparable to the magnitude of the measured physical quantity.
- It should be easy to form multiples and submultiples of the unit.
| We must be able to agree in detail on what we have observed and to communicate it without being misunderstood. Therefore, "observations in physical sciences are generally quantitative, that is, they are expressed with numerical values. Measurement is the process of finding how many times a unit measure of that physical quantity is present in the quantity being measured. The result is expressed as a positive real number and the unit. Therefore It is meaningless to call a physical quantity ‘large’ or ’small' without specifying a standard for comparison. |
Fundamental quantity : A fundamental quantity is a physical quantity whose measurement does not depend upon other physical quantities, i.e., it can be directly measured. It is also called a basic quantity.
Examples : Length, mass, time. These three physical quantities are regarded by convention as dimensionally independent of one another.
They are also basic to the measurement of all other physical quantities in mechanics.
There are seven fundamental quantities: length, mass, time, temperature, electric current, luminous intensity and amount of substance.
Fundamental unit: The unit measure of a fundamental quantity is called a fundamental or base unit. A set of fundamental units forms the basis of a system of units.
Derived quantity : A derived quantity is a physical quantity whose measurement or determination involves the direct measurement of one or more fundamental quantities. A derived quantity is measured and expressed in terms of one or more basic quantities using the respective defining equation.
Examples :
- Area of a rectangle = Unit of length x Unit breadth = m x m = m2. As breadth is also a length measure, determination of area depends upon two length measures.
- Velocity = Unit of displacement / Unit of time =m/s = ms-1. Thus, determination of velocity depends upon measurement of distance, essentially, length, and time.
The above two units are derived units.
Derived unit : The unit measure of a derived quantity is called a derived unit.
A derived unit is formed from one or more fundamental units by means of the mathematical symbols of multiplication and division according to the algebraic relations linking the physical quantities concerned.
System of units: The whole set of units, i.e., all the fundamental and derived units taken together, forms a system of units.
Different systems of units : The concept of an internationally standardized system of units is one of the most fundamental in experimental science. Three major systems of units were used before SI was universally adopted.
- The British Imperial FPS (foot-pound- second) system (1824)
- The French metric CGS (centimetre—gram-second) system (1874)
- The MKS (metre-kilogram-second) system (1889) also a metric system.
- The International System of Units (1960), abbreviated SI.
[Note : The MKS system went through several revisions before its last version, RMKSA (rationalized metre-kilogram-second-ampere) system (1946), was adapted into the International System of Units (SI).]
International System of Units (SI) : The International System of Units, abbreviated SI, is the modern metric system of units of measurement. It was introduced in 1960 with three classes of units : -
- fundamental units (numbering seven)
- supplementary units (numbering two)
- derived units (all the rest).
SI was recently revised on 20th May 2019.
As per new SI units, each of the seven fundamental units (metre, kilogram, etc.) uses one of the following 7 constants which are proposed to be having exact values as given below: 1-The Planck constant, h = 6.62607015 × 10−34 joule-second (J-s or kg m2 s-1). 2-The elementary charge, e = 1.602176634 × 10−19 coulomb (C or A s). 3-The Boltzmann constant, k = 1.380649 × 10−23 joule per kelvin (J K−1 or kg m2 s-2 K-1). 4-The Avogadro constant (number), NA = 6.02214076 × 1023 reciprocal mole (mol−1). 5-The speed of light in vacuum, c = 299792458 metre per second (m s−1). 6-The ground state hyperfine structure transition frequency of Caesium-133 atom, ΔνCs = 9192631770 hertz (Hz or s-1). 7-The luminous efficacy of monochromatic radiation of frequency 540 × 1012 Hz, Kcd = 683 lumen per watt (lm⋅W−1) = 683 cd sr s3 kg-1 m-2, where sr is steradian; the SI unit of solid angle. |
Fundamental quantities in SI their units and symbols :
| Fundamental quantity | SI units | symbols |
| 1) Length | metre | m |
| 2) Mass | kilogram | Kg |
| 3) Time | second | s |
| 4) Temperature | kelvin | K |
| 5) Electric current | ampere | A |
| 6) Luminous Intensity | candela | cd |
| 7) Amount of substance | mole | mol |
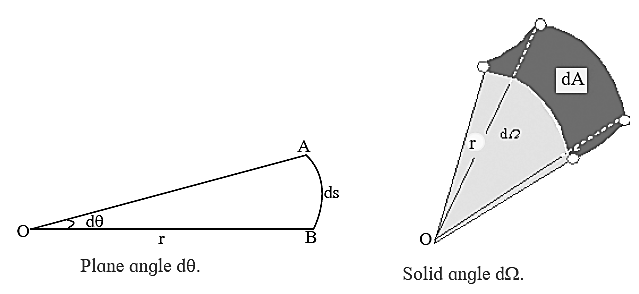
Supplementary units : Besides, the seven fundamental or basic units, there are two more units called supplementary units: (i) Plane angle dθ and (ii) Solid angle dΩ
(i) Plane angle (dθ) : This is the ratio of the length of an arc of a circle to the radius of the circle
dθ = ds/r is the angle subtended by the arc at the centre of the circle. It is measured in radian (rad). An angle θ in radian is denoted as θc.
(ii) Solid angle (dΩ) : This is the 3-dimensional analogue of dθ and is defined as the area of a portion of surface of a sphere to the square of radius of the sphere.
dΩ = dA/r2
Radian : The radian is the coherent unit for plane angle. One radian is the angle subtended at the centre of a circle by an arc that is equal in length to the radius. The radian is also the unit for phase angle. For periodic phenomena, the phase angle increases by 2π rad in one period.
Steradian : The steradian is the coherent unit for solid angle. One steradian is the solid angle subtended at the centre of a sphere by an area of the surface that is equal to the squared radius.
The radian and steradian were temporarily classified as supplementary units in SI, but this category was abolished in 1995.
Rules and style conventions for unit names and unit symbols in SI : Acceptable units in SI have internationally recognized symbols and names. Hence, CGPM gives the following rules and style conventions for unit names and unit symbols:
1- Unit names, including those derived from proper names, are written in lower-case.
2 -Unit symbols are printed in lower-case letters except that.
- The symbol or the first letter of the symbol is an upper-case letter when the name of the unit is derived from the name of a person,
- Examples : The symbol for the litre is L. m (metre), s (second), V (volt), Pa (pascal).
3- Unit symbols remain unchanged in the plural.
Example : l= 95 cm, not 95 cms.
4- Unit symbols are not followed by a period except at the end of a sentence.
Example : ”Its length is 85 cm.” or ”It is 85 cm long.” but not : "It is 85 cm. long.”
5- Multiplication of unit symbols must be indicated by a space or a half-high (centred) dot ( - ), since otherwise some prefixes could be misinterpreted as a unit symbol.
Examples : N-m, for the newton-metre; ms for millisecond but m-s for the metre times second.
6- Division of unit symbols must be indicated by a horizontal line, by a solidus (/ ) or by negative exponents. When several unit symbols are combined, care should be taken to avoid ambiguities, for example by using brackets or negative exponents. A solidus must not be used more than once in a given expression without brackets to remove ambiguities.
Examples : \(\frac{m}{s}\) or m/s or m-s-1, for the metre per second; m-s-1 is the symbol for the metre per second while ms-1 is the symbol for the reciprocal millisecond (103 s-1); mkg /(s3. A) or m-kg-s-3-A-1 but not mkg/s3/A nor mkg /s3A.
7- Unit symbols and unit names are not used together.
Example : C/ kg, C-kg-1, or coulomb per kilogram but not coulomb/kg nor coulomb per kg nor C/kilogram nor coulomb-kg-1 nor C per kg nor coulomb/kilogram.
It is not permissible to use abbreviations for their unit symbols or names, such as sec (for either s or second), sq. mm (for either mm2 or square millimetre), cc (for either cm3 or cubic centimetre), mins (for either min or minutes), hrs (for either h or hours), lit (for either L or litre), amps (for either A or amperes), AMU (for either u or unified atomic mass unit), or mps (for either m/s or metre per second). 0
8- A prefix symbol is used before the symbol of the unit. Thus prefix symbol and units symbol constitute a new symbol for the unit which can be raised to a positive or negative power of 10.
Example : 1ms = 1 millisecond = 10-3s
1μs = 1 microsecond = 10-6s
1ns = 1 nanosecond = 10-9s
Use of double prefixes is avoided when single prefix is available
10-6s =1μs and not 1mms.
10-9s = 1ns and not 1mμs
Derived quantities, Special names in SI of the units and symbol :
| Physical quantity | SI Unit | Symbol, name of the scientist | Equivalent combination of other SI units |
| Force | newton, N | Isaac Newton | kg.m/s2 |
| Energy, work | joule, J | James Joule | N-m=kg-m2/s2 |
| Power | watt, W | James Watt | J/s= kg-m2/s2 |
| Pressure | pascal, Pa | Blaise Pascal | N/m2=kg/s2.m |
| Frequency | hertz, Hz | Heinrich Hertz | s-1 |
| Electric charge | couloumb, C | Charles Couloumb | A.s |
| Electric potential | volt, V | Alessandro Volt | J/A.s=kg.m2/s3.A |
| Electric resistance | ohm, W | George Ohm | V/A= kg.m2/s3.A2 |
| Magnetic induction | tesla, T | Nikola Tesla | Wb/m2=kg/s2.A |
Measurement of Length: To measure the length or distance the SI unit used is metre (m).
- The SI unit of length, the metre, was taken to be the distance between two lines marked on the international prototype bar of platinum-iridium alloy kept at the International Bureau of Weights and Measures (BIPM), Sévres, France. This definition of the metre was replaced in 1960.
- In 1960, a standard for the metre based on the wavelength of orange-red light emitted by atoms of krypton was adopted.
- By 1983 a more precise measurement was developed. It says that a metre is the length of the path travelled by light in vacuum during a time interval of 1/299792458 second. This was possible as by that time the speed of light in vacuum could be measured precisely as c = 299792458 m/s
- Direct measurement of length using various measuring devices is possible for lengths from about 102 m to 10-5 m. A metre scale can measure up to 10-3 m. Vernier callipers, screw gauge and spherometer can measure up to 10-5 m.
- To measure distances beyond the reach of measuring instruments, such as a planet or a star, we use the method of trigonometric parallax.
Parallax method : Large distance, such as the distance of a planet or a star from the Earth, cannot be measured directly with a metre scale, so a parallax method is used for it.
- Parallax is an apparent shift in the position of a nearby object with respect to a more distant one when viewed from different vantage points.
- The angular displacement in the apparent position of a celestial body when observed from two different vantage points is called the parallax angle.
To understand what parallax is, let us do a simple experiment.
Hold your hand in front of you and look at it with your left eye closed and then with your right eye closed. You will find that your hand appears to move against the background. This effect is called parallax.
The parallax increases if you bring the thumb closer.
The line segment joining the two points of observations — here, the two eyes E1, E2 — is called the base line. The longer the base line, the more will be the parallax.
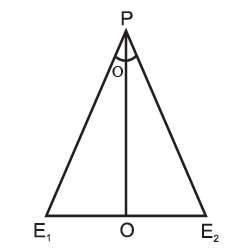
By measuring the parallax angle (θ) and knowing the distance between the eyes E1E2 as shown in Fig. we can determine the distance of the object from us, i.e., OP as E1E2/θ.
Parallax method to determine the distance of a planet and, its diameter :
To measure the distance D of a planet P from us, the planet is viewed simultaneously from two different positions, A and B, on the Earth (Fig.). From the observatory at A, the planet would appear in the direction of the star S1, whereas from B it would appear in the direction of the ‘star S2. The angle θ between these two directions is the parallax angle. Here, AB is the base line.

A, B : Observatories, S1, S2 : Distant stars, D : Earth-to-planet distance, AB = b : Baseline, θ : Parallax angle |
Since D >> b, we can take AB to be the arc of a circle of radius D.
b = D θ
Hence, by measuring b and θ, we can find the distance D of the planet from the Earth.
To determine the diameter of a planet, two diametrically opposite points of the planet are viewed from the same observatory see below Fig.

The angle on between these two directions gives the angular size of the planet, i.e., the angle subtended by the planet at A. If d is the diameter of the planet, d = αD. Hence, having determined D and measuring a, we can determine the diameter d of the planet.
Measurement of Distance to Stars from the earth :
Astronomers use the method of trigonometric parallax for measuring stellar distances from the Earth. Sun is the star which is closest to the Earth. The next closest star is at a distance of 4.29 light years. The parallax measured from two most distance points on the Earth for stars will be too small to be measured and for this purpose we measure the parallax between two farthest points (i.e. 2 AU apart) along the orbit of the Earth around the Sun (see figure). The longest baseline available to us is the distance between the Earth and the Sun, which is called one astronomical unit (au); 1 au = 1.496 x1011 m.
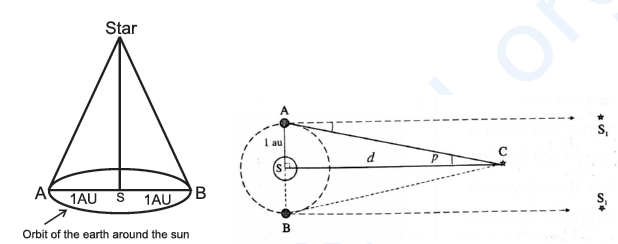
AB : Major axis of the Earth's orbit, S : Sun, C : Nearby star, S1 : Distant background star, d : Earth-to-star distance (AC) ≈ Sun-to-star distance (SC), AB : Baseline = 1 au, p : Annual parallax |
A picture of a nearby star is taken against the background of stars from opposite ends of the major axis of the Earth’s orbit (six months apart).
A very distant star S1 whose position does not appear to change in this period is taken as reference. The annual parallax p of the nearby star is one-half of the total angular shift of the nearby star with respect to the Sun. However, even with this large baseline, p is very small, less than an arcsecond (an arcsecond is an alternative name for one second of plane angle and is equal to 1/3600 of a degree).
Using a parsec for the distance unit for measurements from the Earth and an arcsecond for the parallax angle, the distance of a star d= 1/p
| Parallax angle is inversely proportional to the distance of the star from the Earth. |
Measurement of Mass: . Since 1889, a kilogram was the mass of a shiny piece of platinum-iridium alloy kept in a special glass case at the International Bureau of weights and measures. This definition of mass has been modified on 20th May 2019. The new measure of kilogram is defined in terms of magnitude of electric current.
Thus the kilogram mass can be described in terms of the amount of current which has to be passed through an electromagnet so that it can pull down one side of an extremely sensitive balance to balance the other side which holds one standard kg mass.
Mass is an intrinsic property of matter. Its SI unit, the kilogram, is now defined by taking the exact numerical value of the Planck constant when its value is expressed in the appropriate SI unit, together with the definitions of the metre and the second.
- Direct measurement of mass involves comparison with multiples and submultiples of the kilogram using a beam balance.
- Masses of planets and stars are found indirectly by measuring the period of revolution of their satellites and applying Newton's law of gravitation.
- Masses of atomic and subatomic particles are found indirectly using mass spectrometer, in which the radius of the circular path of a charged particle of a given velocity in a uniform magnetic field is proportional to the mass of the particle.
- In dealing with atomic, molecular and nuclear masses, we use a non-SI unit called the unified atomic mass unit.
Measurement of Time: The SI unit of time is the second (s). For many years, duration of one mean Solar day was considered as reference. A mean Solar day is the average time interval from one noon to the next noon. Average duration of a day is taken as 24 hours. One hour is of 60 minutes and each minute is of 60 seconds. Thus a mean
Solar day = 24 hours = 24x60x60 = 86400 s.
Accordingly a second was defined as 1/86400 of a mean Solar day.
However measurements showed that irregularities in the rotation of the Earth made this an unsatisfactory definition. In order to define the unit of time more precisely, this definition of the second was replaced by one based on atomic frequency standard of the monochromatic radiation of frequency 540 THz from a caesium—133 atom. A small number of national metrology laboratories around the world realize the unit of time with the highest accuracy using atomic clocks. Their data are combined to generate the International Atomic Time (TAI).
Main Page : – Maharashtra Board Class 11th-Physics – All chapters notes, solutions, videos, test, pdf.
Next Chapter : Chapter-2-Mathematical Methods – Online Notes
We reply to valid query.